Simplest Form Math Worksheet Guide for Easy Learning
Educating young minds to understand the fundamental concepts of mathematics has never been as imperative as it is today. Among these foundational concepts lies the idea of simplest form in numbers and equations. It’s a topic that frequently surfaces in various stages of elementary math education, offering students an essential skill set for simplifying expressions, which in turn simplifies problem-solving. This comprehensive guide aims to navigate parents, teachers, and students through the process of mastering simplest forms with clarity and ease, ensuring mathematical fluency and reducing the common intimidation that numbers often bring.
Understanding Simplest Form


The simplest form, often referred to as the lowest terms, is an expression where all the factors of numerator and denominator in a fraction have been cancelled out to their basic form, leaving no common divisors other than 1. The same principle applies to algebraic expressions, where variables are reduced to their least terms.
Key Points:
- Fractions or ratios are reduced to the simplest form by dividing the numerator and the denominator by their greatest common divisor (GCD).
- Expressions involving variables are simplified by canceling common terms.
- The process instills a clear understanding of numbers and their relationships.
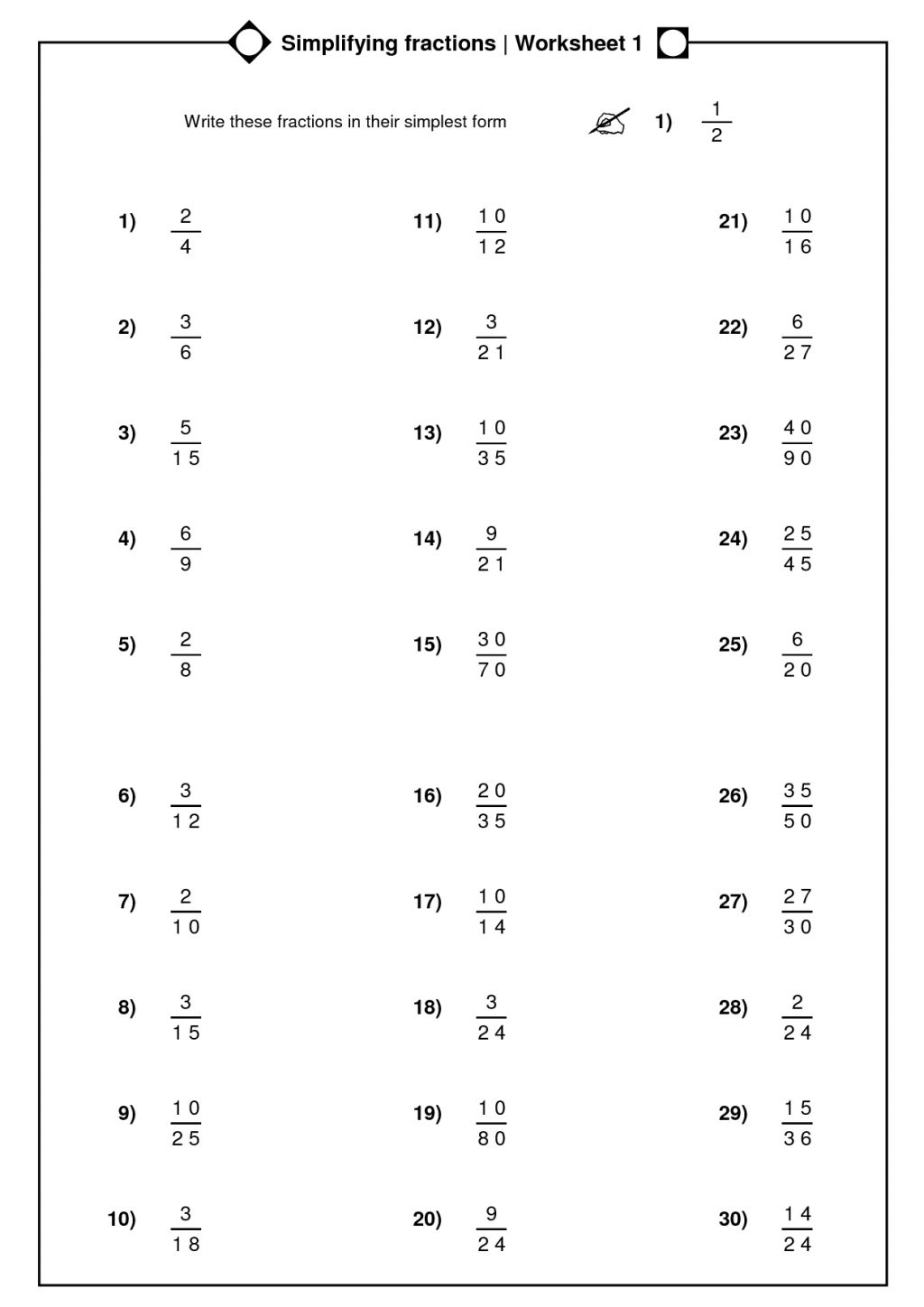
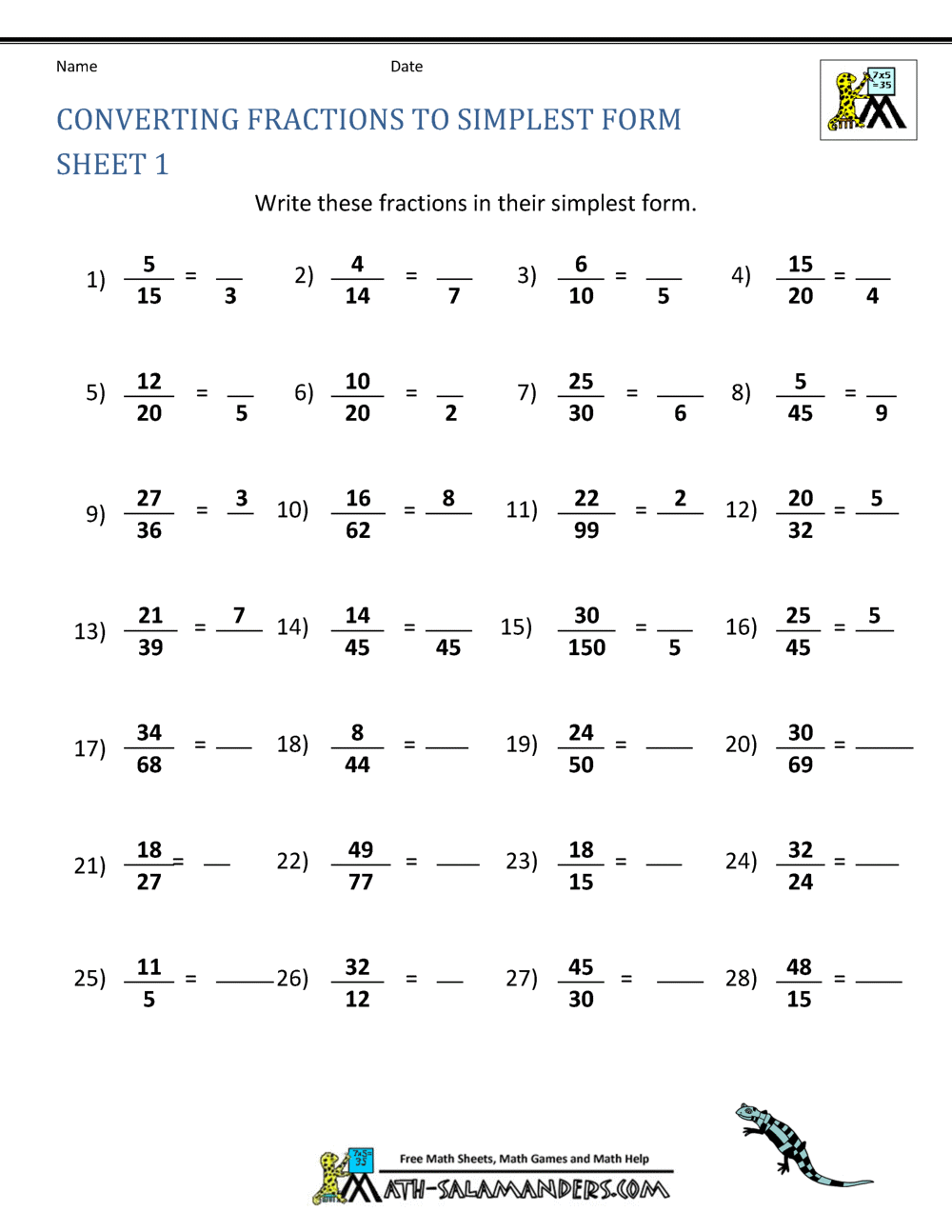
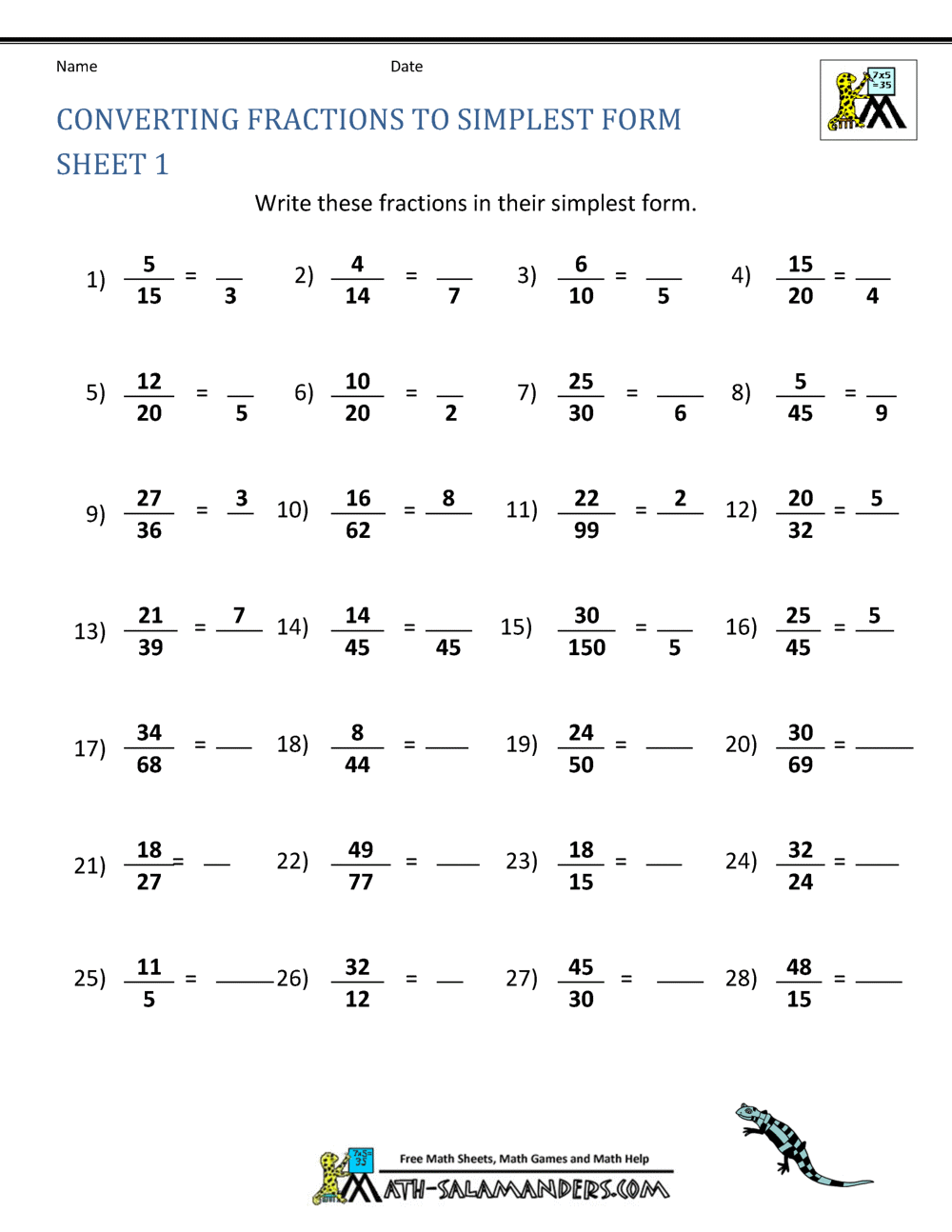
Step-by-Step Guide to Simplifying Fractions

Let’s walk through the process of reducing a fraction to its simplest form:
- Identify the Numerator and Denominator: For example, in the fraction (\frac{8}{12}), the numerator is 8, and the denominator is 12.
- Find the Greatest Common Divisor (GCD): Here, the GCD of 8 and 12 is 4.
- Divide Both Numbers by the GCD: (8 \div 4 = 2) and (12 \div 4 = 3), giving us the fraction (\frac{2}{3}).
- Verify that the Result is in Simplest Form: (\frac{2}{3}) has no common divisors other than 1, making it the simplest form.
💡 Note: Always check for the lowest terms; a common mistake is not fully simplifying, especially with larger numbers.
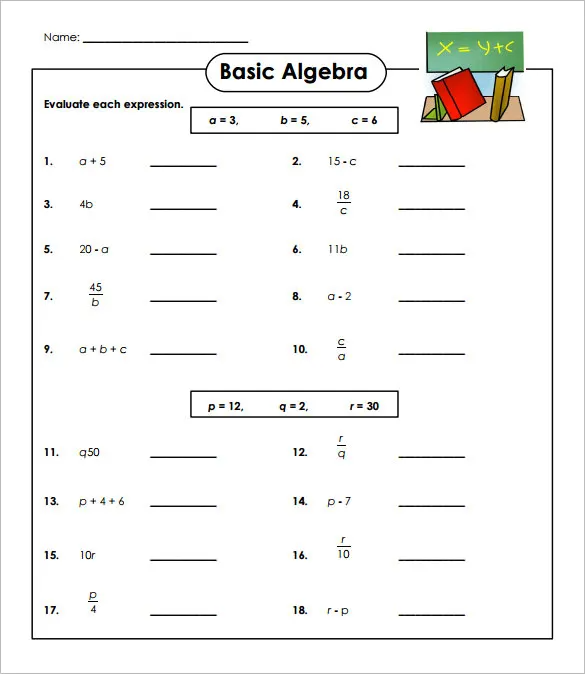
Simplifying Algebraic Expressions


Algebra brings its unique set of challenges, but the principles remain:
- Identify Like Terms: Group together terms with the same variables and exponents.
- Cancel Out Common Factors: Cancel out common factors between the numerator and denominator.
- Combine Like Terms: Simplify the expression by combining like terms.
For example, the expression (\frac{12x^2 + 6x}{3x}) can be simplified by:
- Factoring out the common (3x) from numerator and denominator: (\frac{3x(4x + 2)}{3x}).
- Dividing the entire expression by (3x), giving us (4x + 2).
✍️ Note: Variables with powers must match for cancellation to be valid. E.g., (x^2) cannot cancel with (x).
Common Mistakes and How to Avoid Them

| Mistake | How to Avoid |
|---|---|
| Overlooking simplification | Always ask if the fraction or expression can be simplified further. |
| Confusing variable degrees | Remember only like terms can be canceled or combined. |
| Neglecting common factors | Find the GCD of the entire expression, not just single terms. |
The journey towards mastering the concept of simplest forms in mathematics is an incremental process, filled with opportunities for learning, growth, and a deeper appreciation of numbers and their structures. The steps outlined above, from understanding the core concept to practical application, aim to demystify this essential math skill. By engaging in regular practice, both students and educators can foster a more intuitive understanding of numbers, nurturing a lifelong skill of problem-solving through simplification. The benefits extend beyond the classroom, as these principles of reduction and efficiency permeate various aspects of life, teaching us to find the essence in complexity, a skill that transcends mathematics itself.
What is meant by “simplest form” in mathematics?
+
In mathematics, the simplest form refers to a number, fraction, or expression in which all common factors have been cancelled out, leaving the most reduced, basic form possible without any common divisors other than 1.
How do I find the GCD for simplifying fractions?

+
Find the GCD by listing the factors of both the numerator and denominator and identifying the largest number that divides both without remainder. Alternatively, use methods like prime factorization or the Euclidean algorithm for efficiency.
Why is simplifying important in algebra?
+
Simplifying algebraic expressions reduces complexity, making equations easier to solve. It also helps in understanding the relationship between terms and provides insights into the properties of numbers and variables.



