Two Step Equations Made Easy: Simple Worksheet

Learning to solve two-step equations is a fundamental skill in algebra that empowers students to tackle more complex mathematical problems in the future. Imagine being able to break down seemingly intricate equations into simple, manageable steps. This guide offers a streamlined approach to mastering two-step equations, making the learning process not just straightforward but also enjoyable. Let's dive into the step-by-step methods of solving these equations with the help of a two-step equations worksheet.
Understanding Two-Step Equations

A two-step equation involves performing two operations to isolate the variable. These operations could be addition, subtraction, multiplication, or division. Here’s a basic structure:
- ax + b = c where:
- a and b are coefficients (numbers before or after variables).
- x is the variable we need to find.
- c is a constant number.
Solving Two-Step Equations

Let’s explore how to solve these equations using an example:
Example: 2x + 5 = 11

Step 1: Isolate the variable term
- Start by getting rid of the constant term (+5 in this case). To do this, subtract 5 from both sides:
2x + 5 - 5 = 11 - 5
2x = 6
Step 2: Solve for the variable
- Now, you need to isolate x. Divide both sides by the coefficient of x, which is 2:
(2x) / 2 = 6 / 2
x = 3
🌟 Note: Always check your answer by substituting it back into the original equation to ensure the equation holds true.
General Steps

- Isolate the variable term by applying the inverse operation to the constant term.
- Solve for the variable by applying the inverse operation to the coefficient.
Practicing with Worksheets

Using worksheets to practice can significantly improve your speed and accuracy when solving two-step equations. Here’s a simple table format to help you organize your practice:
| Equation | Step 1 | Step 2 | Solution |
|---|---|---|---|
| 2x + 3 = 7 | 2x + 3 - 3 = 7 - 3 | (2x) / 2 = 4 / 2 | x = 2 |
| 3y - 9 = 6 | 3y - 9 + 9 = 6 + 9 | (3y) / 3 = 15 / 3 | y = 5 |
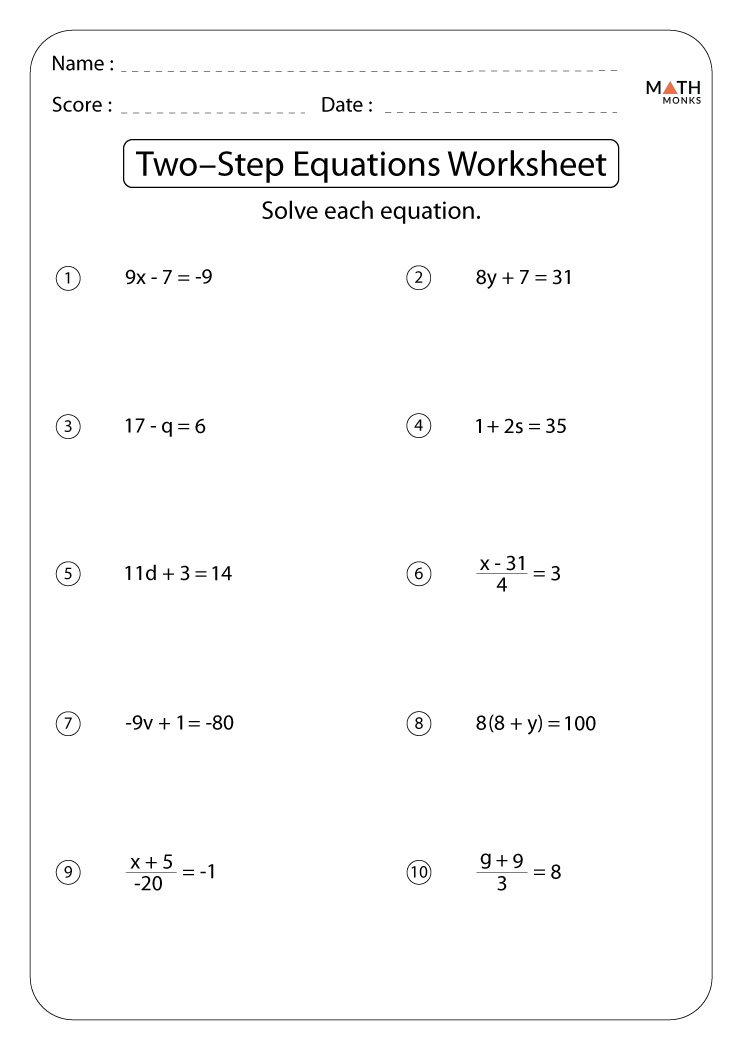
Tips for Practicing

- Start with simpler equations to build confidence.
- Use both positive and negative constants and coefficients.
- Increase complexity gradually by including fractions or decimals.
- Try reverse-solving where you start with the answer and create an equation.
Final Thoughts

We’ve covered the essentials of solving two-step equations, from understanding their structure to breaking them down into manageable steps. Through practice, especially with well-organized worksheets, you’ll find these equations become second nature. Remember, checking your solutions is as important as solving them to ensure accuracy. With these skills in hand, you’re set to tackle more complex algebraic problems with ease. Keep practicing, and let these equations become a game rather than a challenge!
Why is it important to check the solution of a two-step equation?

+
Checking the solution ensures that your answer is correct and provides verification that you’ve solved the equation accurately.
What are the common mistakes students make with two-step equations?

+
Frequent errors include:
- Not applying the same operation to both sides of the equation.
- Mistakes with signs, like forgetting to flip the sign when dividing or multiplying by a negative.
- Errors in arithmetic or not simplifying completely.
Can I use two-step equations to solve real-life problems?

+
Yes, two-step equations are often used in various fields like finance, engineering, or any scenario where one variable depends on two different quantities.
How can I make solving two-step equations more fun?
+
Consider turning equation solving into a game:
- Create a treasure hunt where solving equations leads to clues.
- Use timers to add a competitive element, racing against time.
- Work in pairs or small groups to discuss strategies.