5 Simple Interest Tricks to Boost Your Math Skills

Understanding how to calculate and manipulate simple interest isn't just a fundamental math skill; it's a gateway to mastering more complex financial concepts, improving problem-solving abilities, and even enhancing everyday decision-making. Here, we explore five simple interest tricks that can significantly boost your mathematical prowess, making you adept in both theoretical calculations and practical applications.
1. Master the Simple Interest Formula

The journey begins with the bedrock of simple interest calculation:
[ I = P \times r \times t ]
where:- I stands for Interest
- P is the Principal amount
- r is the rate of interest per period
- t is the time duration for which the interest is calculated
Commit this formula to memory, not just for exams but for everyday financial literacy. Understanding this foundational equation will make you feel confident in dealing with investments, loans, or even casual calculations.
2. Understand Time and Rate Conversions

The formula might seem straightforward, but it’s crucial to realize that r and t must be in the same unit. For instance, if the rate is annual, the time should be in years, not months or days. Here are some conversion tips:
- If the rate is given as a percentage, convert it to a decimal (e.g., 5% to 0.05).
- If time is given in days or months, convert it to years for annual rates.
💡 Note: To convert time to years, remember 365 days or 12 months equals 1 year for the sake of simple interest calculations.
3. Apply Simple Interest in Real-Life Scenarios

Simple interest isn’t just a classroom exercise; here are some practical applications:
- Short-term Loans: Calculate the exact interest you’ll pay or earn on short-term loans, like a car loan or a personal loan.
- Fixed Deposits: When investing, understand the potential earnings from fixed deposits where interest is calculated at simple rates.
- Billings and Discounts: Businesses can use simple interest to calculate late fees, discounts, or prorated charges.
By practicing these scenarios, you not only improve your math skills but also prepare yourself for real-world financial decisions.
4. Use Simple Interest for Problem Solving
Simple interest tricks can enhance your problem-solving skills:
- Find the Time: Given the interest, principal, and rate, rearrange the formula to solve for time, ( t = \frac{I}{P \times r} ).
- Determine the Rate: Use ( r = \frac{I}{P \times t} ) to find out the rate applied on an amount.
- Figure Out the Principal: If you know the interest, rate, and time, you can calculate the principal with ( P = \frac{I}{r \times t} ).
This trick allows you to dissect financial problems and find unknown variables, making you more resourceful in analytical thinking.
5. Dive into Compound Interest and Beyond

Once you’ve mastered simple interest, you’re ready to explore more advanced topics like:
- Compound Interest: Understand how compound interest works by using the formula ( A = P(1 + \frac{r}{n})^{nt} ), where n is the number of times interest is compounded per period.
- Continuous Compounding: Grasp the concept of interest compounding continuously, represented by the formula ( A = Pe^{rt} ), where e is the mathematical constant approximately equal to 2.71828.
- Amortization: Learn how loan payments work over time, including interest and principal repayment.
These concepts not only expand your financial knowledge but also increase your proficiency with complex calculations.
Mastering these simple interest tricks transforms you from a passive learner into an active problem solver. Not only will these skills give you an edge in academics or financial management, but they will also equip you with the ability to navigate through the intricacies of personal finance and investment with ease. The power of understanding simple interest opens doors to a lifetime of better decision-making, grounded in solid mathematical reasoning.
Why is simple interest important?

+
Simple interest is fundamental because it’s the baseline for understanding how interest works in general. It’s directly applicable in scenarios where interest rates don’t change, like certain types of loans, short-term investments, or when calculating discounts and late fees.
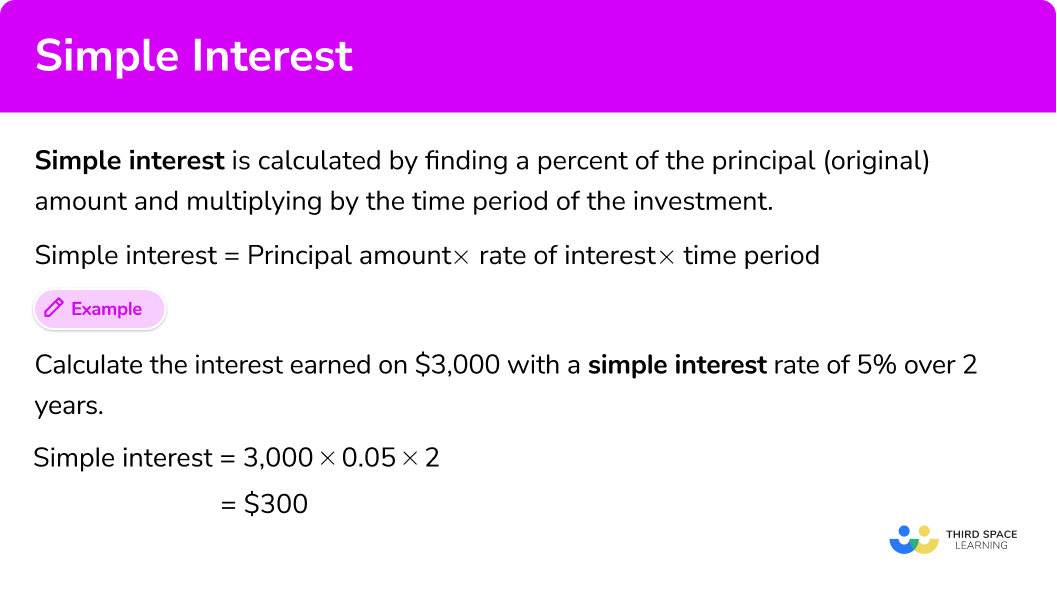
What’s the difference between simple interest and compound interest?
+
Simple interest is calculated only on the initial principal, whereas compound interest calculates interest not only on the initial principal but also on the accumulated interest from previous periods. This means compound interest can lead to exponential growth of your investment or debt.
Can I convert a simple interest rate into an effective annual rate (EAR)?

+
If the interest rate is simple and you need to compare it with compound interest rates, you can’t directly convert the simple rate into an EAR. However, understanding how compound interest works can help you comprehend different investment scenarios better.