5 Ways to Solve Similar Figures Worksheet With Answers
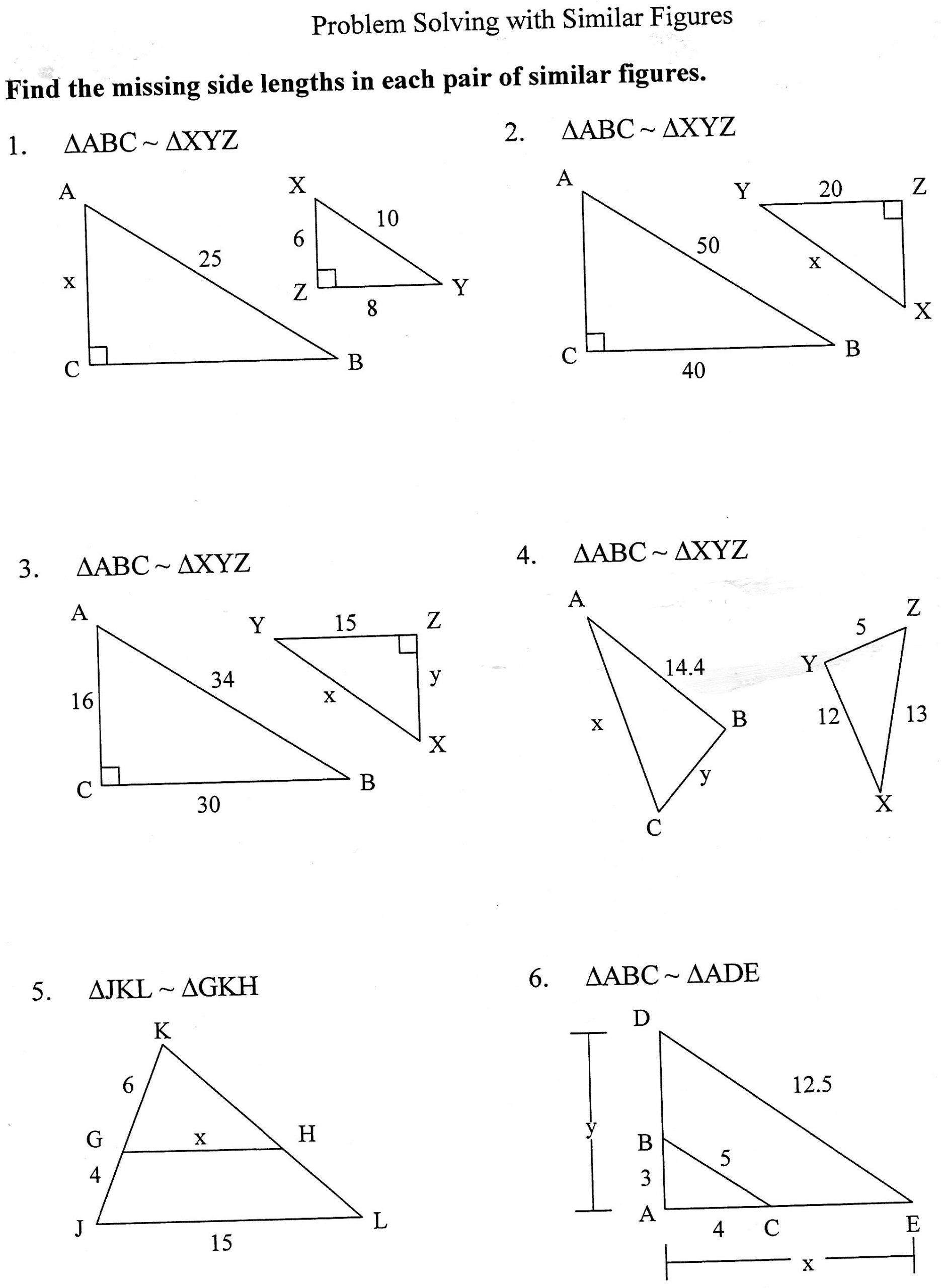
When students encounter similar figures worksheet in their Geometry or Mathematics curriculum, they often feel overwhelmed. However, with a systematic approach and understanding of the core principles, solving these problems can be straightforward. Let's dive into five methods to tackle these worksheets, complete with answers for practice.
1. Understand Similarity Basics

Before we delve into the methods, let's briefly go over what makes figures similar:
- Corresponding Angles: Are congruent.
- Corresponding Sides: Have proportional lengths.
- Similarity Ratio: The ratio of the lengths of corresponding sides is constant.
📝 Note: Ensure you recognize that all angles in similar figures must be congruent, or the figures are not similar.
2. Setting Up Proportions

The most common method to solve for unknown values in similar figures is by setting up proportions:
- Identify corresponding sides.
- Set up a proportion with the known lengths.
- Solve for the unknown by cross-multiplying.
Example: If a triangle has sides of 3, 4, and 5 cm, and another similar triangle has a side known to be 6 cm:
| Side in Original Triangle (cm) | Side in Similar Triangle (cm) |
| 3 | 6 |
| 4 | ? |
| 5 | ? |

Setting up the proportion:
\[
\frac{3}{6} = \frac{4}{x}
\]
Solving for x:
\[
x = \frac{4 \times 6}{3} = 8 \text{ cm}
\]
📚 Note: Cross-multiplying involves multiplying the numerator of one fraction by the denominator of the other and setting the product equal to the cross-products on the opposite side of the equation.
3. Use The Scale Factor

Another effective method is using the scale factor, which is the ratio of the lengths of corresponding sides:
- Determine the scale factor by dividing the corresponding sides.
- Apply the scale factor to find other sides or distances.
Example: If one triangle has sides of 8, 12, and 16 cm, and another has a corresponding side of 4 cm, the scale factor is:
[ \frac{8}{4} = 2 ]
Using the scale factor, the other sides are:
- New side 1: 12 \div 2 = 6 \text{ cm}
- New side 2: 16 \div 2 = 8 \text{ cm}
🔧 Note: When using the scale factor, ensure you apply it consistently to all sides or distances within the similar figures.
4. Apply the AA (Angle-Angle) Postulate

If two angles in one triangle are congruent to two angles in another triangle, the triangles are similar by the AA postulate. This method is useful when dealing with angles:
- Identify two congruent angles.
- Use the fact that the third angle must also be congruent because the sum of angles in a triangle is 180°.
5. Practical Examples and Practice

Let's go through some practical examples to solidify your understanding:
Example 1

A triangle has sides of 10 cm, 15 cm, and 20 cm. Another triangle has one side of 6 cm. Find the lengths of the other sides.
Solution:
- Set up proportion:
\[ \frac{10}{6} = \frac{15}{x} \]
x = 9 \text{ cm} - Other side:
\[ \frac{20}{6} = \frac{y}{6} \]
y = 12 \text{ cm}
Example 2

Two triangles are similar by AA with angles 40°, 75°, and 65°. If one triangle has sides 3, 4, and 5, find the sides of the other triangle with one side known as 6 cm.
Solution:
- Using the scale factor:
\[ \frac{6}{3} = 2 \] - The other sides would be:
4 \times 2 = 8 \text{ cm}
5 \times 2 = 10 \text{ cm}
In conclusion, mastering similar figures involves understanding the core principles like corresponding sides, angles, and the similarity ratio. By applying different methods like setting up proportions, using the scale factor, or recognizing angle-angle similarity, you can solve various types of problems efficiently. Remember to always double-check your calculations and ensure consistency in applying methods to both figures. Practice will make these concepts second nature, leading to confidence and success in solving similar figures worksheets.
What makes figures similar?

+
Figures are similar when their corresponding angles are congruent, and the ratios of the lengths of their corresponding sides are proportional.
How do you find the similarity ratio?

+
You find the similarity ratio by dividing the length of any corresponding side in one figure by the length of the corresponding side in the other figure.
What is the AA postulate?

+
The AA (Angle-Angle) postulate states that if two angles of one triangle are congruent to two angles of another triangle, then the triangles are similar.
Related Terms:
- Similar figures Worksheet PDF