Master Multiplication and Division with Significant Figures

Understanding Significant Figures

Before diving into the process of mastering multiplication and division with significant figures, it’s fundamental to grasp what significant figures are and why they matter. Significant figures, or “sig figs,” reflect the precision and accuracy of a measurement or calculation. They show how many digits in a number are trustworthy, thereby providing a level of certainty when performing calculations.
Significant figures are crucial in scientific and engineering fields where precision is paramount. Here’s a quick overview:
- Non-zero digits are always significant.
- Zeros between non-zero digits are significant.
- Leading zeros (zeros before a non-zero digit in a decimal) are not significant.
- Trailing zeros in numbers without a decimal point are ambiguous but can be considered significant if there is a decimal point present.
- Trailing zeros after a decimal point are significant.
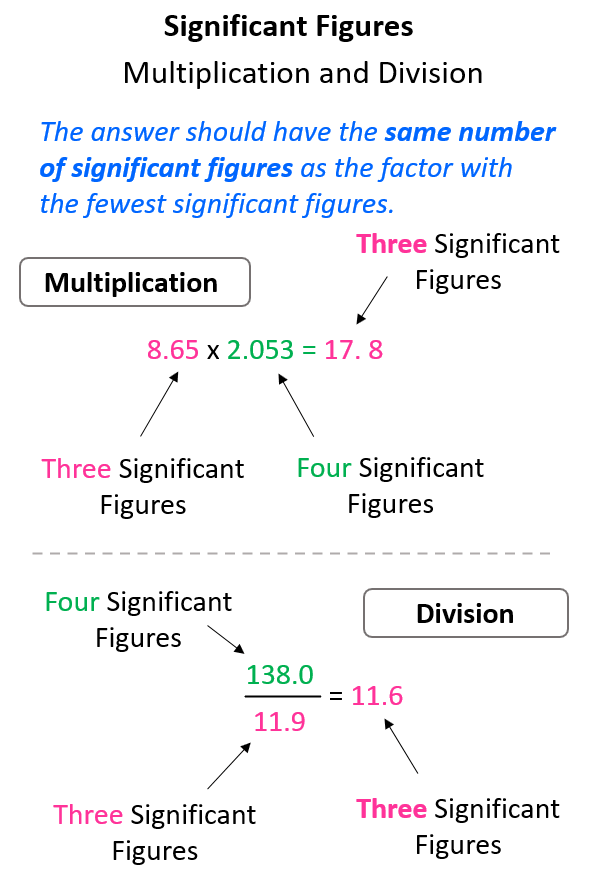
Rules for Multiplication and Division with Significant Figures

When you embark on performing multiplication or division involving numbers with significant figures, following these rules ensures your results are as precise as they can be:
Count the Significant Figures: Determine how many significant figures each number in the calculation has.
Perform the Calculation: Conduct the multiplication or division as you would normally.
Determine Final Significant Figures: The result should be rounded to the same number of significant figures as the measurement with the least significant figures in your initial calculation.
📌 Note: In cases of multiplication and division, the number of significant figures in the final result is equal to the smallest number of significant figures among all the numbers used in the calculation.
Example Calculation

Let’s walk through an example:
- If you multiply
12.15(4 significant figures) by4.0(2 significant figures):- The product is
48.60(without rounding). - Since
4.0has the least significant figures (2), the answer should be rounded to two significant figures: 49
- The product is
Mastering the Techniques
To truly excel in multiplication and division with significant figures:
Practice: Use various numbers with different sig figs and calculate. Check your work to build confidence.
Understand the Purpose: Remember, significant figures are a tool for communicating precision, not just a rule for rounding.
Use Calculators Wisely: Many scientific calculators have a significant figure mode. Familiarize yourself with it to quickly understand the correct level of precision in your answers.
Step-by-Step Guide

Here’s a step-by-step guide to ensure you get it right:
Identify Significant Figures: Analyze each number in your calculation to determine its significant figures.
- Example:
2.50has three significant figures,0.300has three, and5.020has four.
- Example:
Perform the Calculation: Do the actual multiplication or division.
Apply Sig Fig Rule: Round the result to the least number of significant figures in your initial numbers.
- If you multiply
2.50 x 5.020, your result before rounding is12.550. Since2.50has the least significant figures (3), your answer is 12.5.
- If you multiply
Common Challenges and Tips

Handling significant figures can sometimes feel like a challenge, especially when:
Complex Calculations: Dealing with logarithms, exponents, or compound calculations can complicate the process. Always break down complex problems into simpler steps.
Mixed Operations: When operations include both multiplication/division and addition/subtraction, significant figures must be managed for each step.
Exact Numbers: Remember, quantities like “5” in “5 pieces of paper” are considered to have infinite significant figures in calculations.
Here are some tips to navigate these challenges:
List Operations: When dealing with mixed operations, make a list to keep track of sig figs for each sub-operation before the final rounding.
Decimal Placement: Be cautious of decimal point placement, especially when rounding, to avoid erroneous results.
Practice with Real Data: Use real-world data or published experimental values to practice, enhancing your understanding of precision and accuracy in real scenarios.
🌟 Note: Significant figures aren't just about rounding; they're about conveying the precision of your measurements or calculations. Keep that in mind!
Key Applications of Significant Figures

Significant figures are not just a mathematical exercise; they have practical applications:
Error Estimation: Understanding sig figs helps in estimating the error in experimental measurements or calculations.
Engineering Design: Engineers use significant figures to determine the precision required in parts or components.
Scientific Research: Precision and accuracy, conveyed through significant figures, are crucial for replicable and reliable scientific findings.
Table of Significant Figures in Common Measurements

Here’s a small table to illustrate significant figures in common units:
| Unit | Measurement | Significant Figures |
|---|---|---|
| Meter | 12.00 | 4 |
| Gram | 0.500 | 3 |
| Liter | 50.0 | 3 |

Final Thoughts
Mastering multiplication and division with significant figures isn’t just about getting the numbers right; it’s about understanding the precision of your work, communicating that precision effectively, and making informed decisions based on the level of certainty in your measurements.
Significant figures bridge the gap between theoretical calculations and practical applications, ensuring the accuracy and reliability of scientific and engineering endeavors. Whether you’re solving a physics problem, designing a bridge, or performing complex chemical reactions, understanding and applying significant figures correctly is essential.
Why are significant figures important?
+
Significant figures help indicate the precision of a measurement or calculation. They ensure that the results of mathematical operations reflect the level of certainty in the original data, thereby communicating how reliable or accurate those results are.
How do I count significant figures in a number?

+
Here’s how to count significant figures: - All non-zero digits are significant. - Zeros between non-zero digits are significant. - Leading zeros in decimal numbers are not significant. - Trailing zeros in numbers without a decimal point are significant only if there’s a decimal point. - Trailing zeros after a decimal point are always significant.
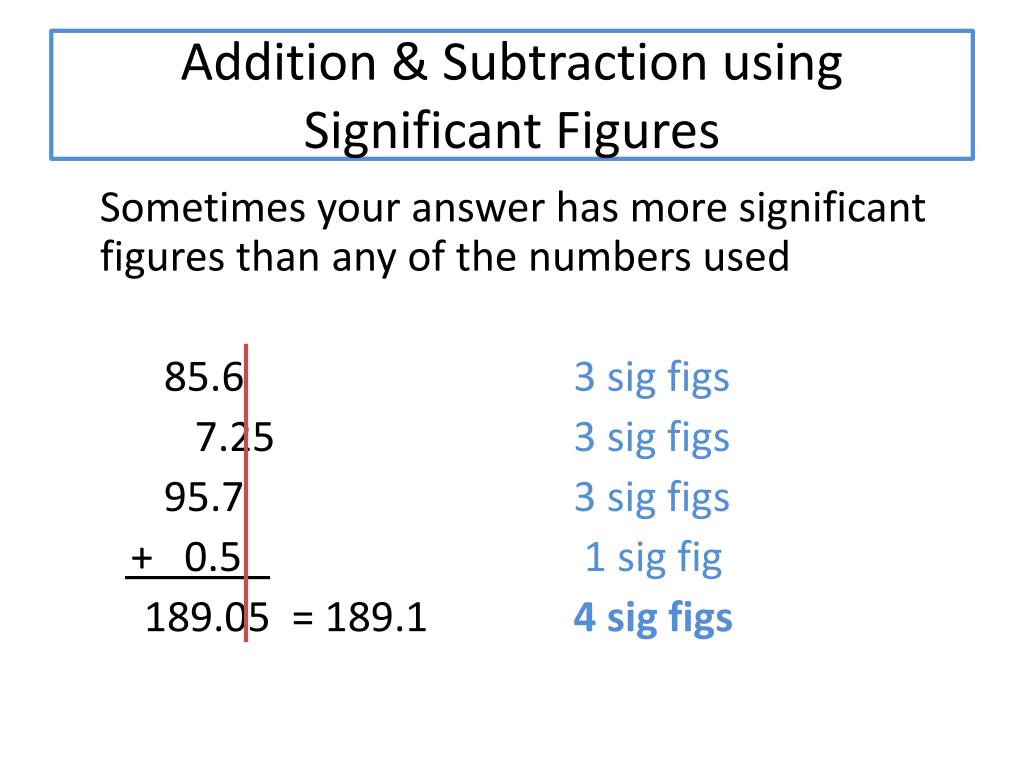
What happens to significant figures in addition and subtraction?

+
For addition and subtraction, the result should be rounded to the least number of decimal places among the numbers being added or subtracted. Unlike multiplication and division, here you look at the least number of decimal places, not the number of significant figures.



