Mastering Significant Figures in Addition and Subtraction

Significant figures, or "sig figs," play a pivotal role in scientific and mathematical computations where precision and accuracy are paramount. Mastering how to handle significant figures during addition and subtraction can elevate your computational accuracy and ensure you communicate results with the appropriate level of precision. Let's delve into a comprehensive guide on managing significant figures in these operations.
Understanding Significant Figures

Before diving into the rules for addition and subtraction, a basic understanding of what constitutes a significant figure is essential:
- All non-zero digits are significant.
- Zeros between non-zero digits (trapped zeros) are significant.
- Leading zeros are not significant.
- Trailing zeros in a number that includes a decimal point are significant.
- Exact numbers have an infinite number of significant figures.
Rules for Addition and Subtraction

The rule for determining significant figures in addition and subtraction differs from that of multiplication and division:
- The result should have the same number of decimal places as the number with the fewest decimal places in the operation.
Here's how you can apply this:
Step-by-Step Process for Addition
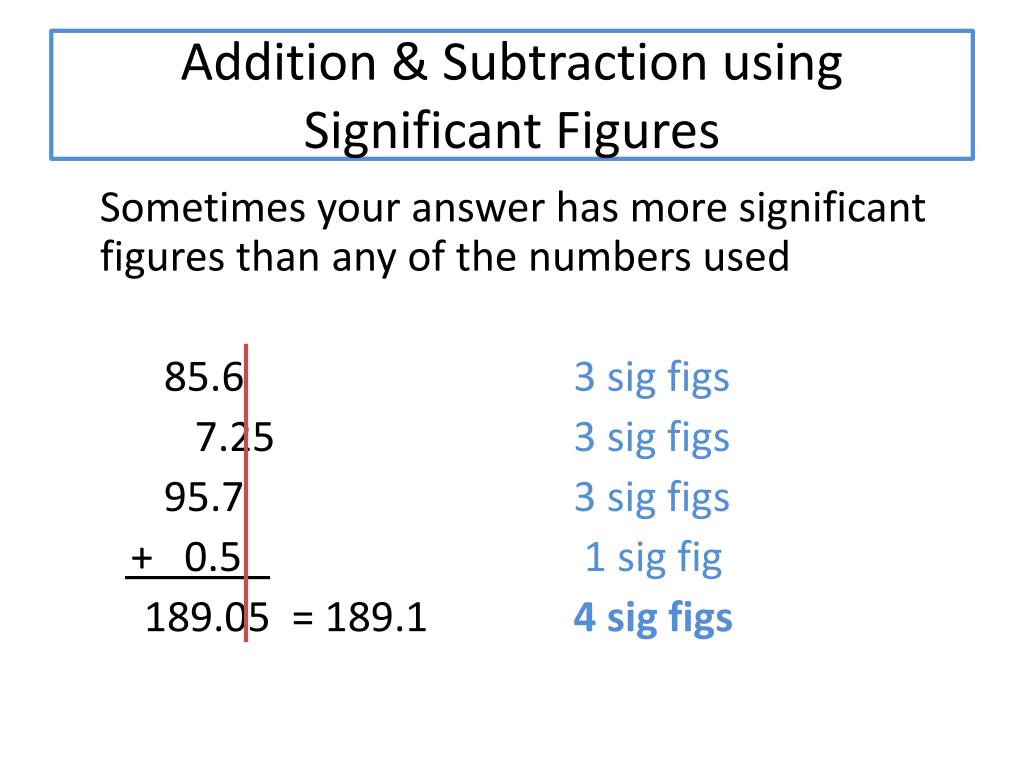
- Identify the numbers you are going to add.
- Determine the number of decimal places for each number.
- The result will have the same number of decimal places as the number with the fewest decimal places.
- Align the numbers by their decimal points and add as usual.
Example
| Operation | Decimals | Result |
|---|---|---|
| 22.45 + 0.700 | 2 and 3 | 23.15 |
| 1.3 + 56.243 | 1 and 3 | 57.5 |

🔍 Note: In the first example, both numbers have significant trailing zeros, which affects the final answer.
Step-by-Step Process for Subtraction

- Identify the numbers you are going to subtract.
- Determine the number of decimal places for each number.
- The result will have the same number of decimal places as the number with the fewest decimal places.
- Align the numbers by their decimal points and subtract as usual.
Example
| Operation | Decimals | Result |
|---|---|---|
| 88.24 - 24.1 | 2 and 1 | 64.1 |
Tricky Situations

Some operations can be more nuanced:
- Adding or Subtracting Zeroes: If zero is one of the numbers in the calculation, it has no effect on the significant figure rule.
- Trailing Zeros in Whole Numbers: If there are trailing zeros in a whole number without a decimal point, these zeros are not significant unless otherwise indicated.
- Final Digits in Results: If your result has a digit at the end that matches the least precise measurement, you can round or maintain it based on the context or additional information.
Common Mistakes to Avoid

- Ignoring significant figures when rounding or truncating your result.
- Not adhering to the rule of fewest decimal places, leading to over-precise results.
- Misinterpreting the significance of trailing zeros in whole numbers or numbers without decimal points.
As we've explored the rules and applications of significant figures in addition and subtraction, understanding these principles can significantly enhance the precision and accuracy of your scientific work. Remember, the key is to ensure that your final result reflects the least precise measurement used in your calculation. This approach not only maintains accuracy but also communicates the correct level of confidence in your measurements or calculations.
Why are significant figures important in scientific calculations?

+
Significant figures help to express the precision and uncertainty in measurements. They ensure that the results of calculations do not imply more precision than the data warrants, thus maintaining scientific integrity.
What happens when all the numbers in addition or subtraction have the same number of decimal places?

+
When all numbers have the same number of decimal places, the result should match this precision. For example, adding or subtracting 2.35, 4.65, and 3.25 all have two decimal places, so the result will also have two decimal places.
How do I handle significant figures when dealing with exact numbers?

+
Exact numbers, which include defined quantities or counted numbers, do not limit the number of significant figures in your result. They are considered to have an infinite number of significant figures.



