Master Series and Parallel Circuits with Answer Sheets

Understanding series and parallel circuits is fundamental for anyone delving into the world of electronics, whether for hobbyist projects, professional work, or academic pursuits. These basic electrical configurations form the backbone of many electronic devices, systems, and engineering concepts. In this comprehensive guide, we'll explore the principles behind series and parallel circuits, learn how to calculate key parameters, and ensure a solid understanding through practical exercises with answer sheets.
What are Series Circuits?

In a series circuit, components are connected end-to-end, forming a single path for the current to flow through:
- Current: The current is the same throughout the circuit because there is only one path for the electrons to travel.
- Voltage: The total voltage supplied to the circuit is equal to the sum of the voltage drops across each component.
- Resistance: The total resistance is the sum of all resistances in the circuit.
Series Circuit Calculations

Here’s how you can calculate various parameters in a series circuit:
- Total Resistance (R_total):
R_total = R1 + R2 + R3 + … - Total Current (I_total):
I_total = V_total / R_total - Voltage Drop Across Each Resistor (V):
V = I_total * R(where R is the resistance of the individual component)
⚠️ Note: Ohm’s Law (V = I * R) is the fundamental equation for understanding how voltage, current, and resistance interact in a circuit.
What are Parallel Circuits?

In a parallel circuit, components are connected across the same two points, creating multiple paths for the current to flow:
- Voltage: Each component has the same voltage as the power source.
- Current: The total current entering the circuit equals the sum of currents through all parallel branches.
- Resistance: The total resistance of the circuit is less than the smallest individual resistance.
Parallel Circuit Calculations

Here are the formulas for calculating parameters in a parallel circuit:
- Total Resistance (R_total):
1/R_total = 1/R1 + 1/R2 + 1/R3 + … - Total Current (I_total):
I_total = I1 + I2 + I3 + … - Current Through Each Resistor (I):
I = V_total / R(where R is the resistance of the individual component)
Comparing Series and Parallel Circuits

Here’s a table comparing key aspects of series and parallel circuits:
| Aspect | Series Circuit | Parallel Circuit |
|---|---|---|
| Current | Equal in all components | Distributed among components |
| Voltage | Divided among components | Equal across all components |
| Resistance | Sum of all resistances | Less than smallest individual resistance |
| Failure | Failure of one component breaks the entire circuit | Other paths remain functional if one component fails |

Practical Exercises
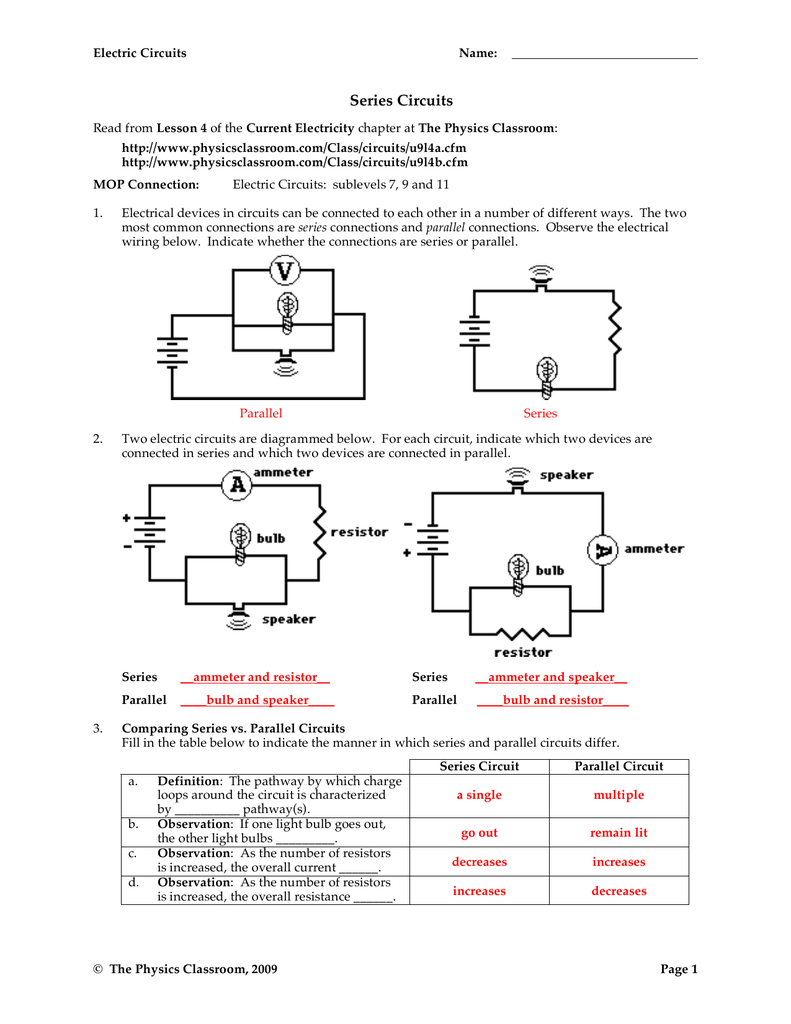
To solidify your understanding of series and parallel circuits, here are some exercises with answer sheets:
Exercise 1: Series Circuit

Calculate the total resistance, current through the circuit, and voltage drop across each resistor in a series circuit with R1 = 100Ω, R2 = 200Ω, and R3 = 300Ω with a total voltage of 12V.
Answer Sheet:
- R_total = 100Ω + 200Ω + 300Ω = 600Ω
- I_total = V_total / R_total = 12V / 600Ω = 0.02A
- V_R1 = I_total * R1 = 0.02A * 100Ω = 2V
- V_R2 = I_total * R2 = 0.02A * 200Ω = 4V
- V_R3 = I_total * R3 = 0.02A * 300Ω = 6V
Exercise 2: Parallel Circuit

Calculate the total resistance, current through each branch, and total current when resistors of 300Ω, 600Ω, and 900Ω are connected in parallel with a voltage source of 18V.
Answer Sheet:
- R_total = 1/(1/300Ω + 1/600Ω + 1/900Ω) ≈ 180Ω
- I_R1 = 18V / 300Ω = 0.06A
- I_R2 = 18V / 600Ω = 0.03A
- I_R3 = 18V / 900Ω = 0.02A
- I_total = I_R1 + I_R2 + I_R3 = 0.06A + 0.03A + 0.02A = 0.11A
From the exercises above, you can see how series and parallel circuits behave differently in terms of current, voltage, and resistance. This understanding is crucial for effectively designing, troubleshooting, and analyzing electronic circuits.
By mastering these fundamental circuit configurations, you are well on your way to becoming proficient in electronics. Whether it's for solving practical problems, designing new electronic systems, or simply satisfying your curiosity about how everyday devices work, this knowledge forms the bedrock of electrical engineering. Keep practicing with different scenarios to get a feel for how these circuits operate in real-world applications, and always remember the principles of Ohm's Law and Kirchhoff's Laws when analyzing circuits.
Why is it important to understand series and parallel circuits?

+
Understanding these circuits allows you to analyze how electrical components interact, which is crucial for troubleshooting, designing, and predicting the behavior of electronic systems.
How does a series circuit differ from a parallel circuit in terms of failure?

+
In a series circuit, failure of one component will break the entire circuit since there’s only one path for current. In a parallel circuit, if one component fails, the current can still flow through other branches.
Can you have a mix of series and parallel circuits?

+
Yes, most practical circuits are combinations of series and parallel configurations, known as series-parallel circuits, allowing for complex functionality in electronic devices.