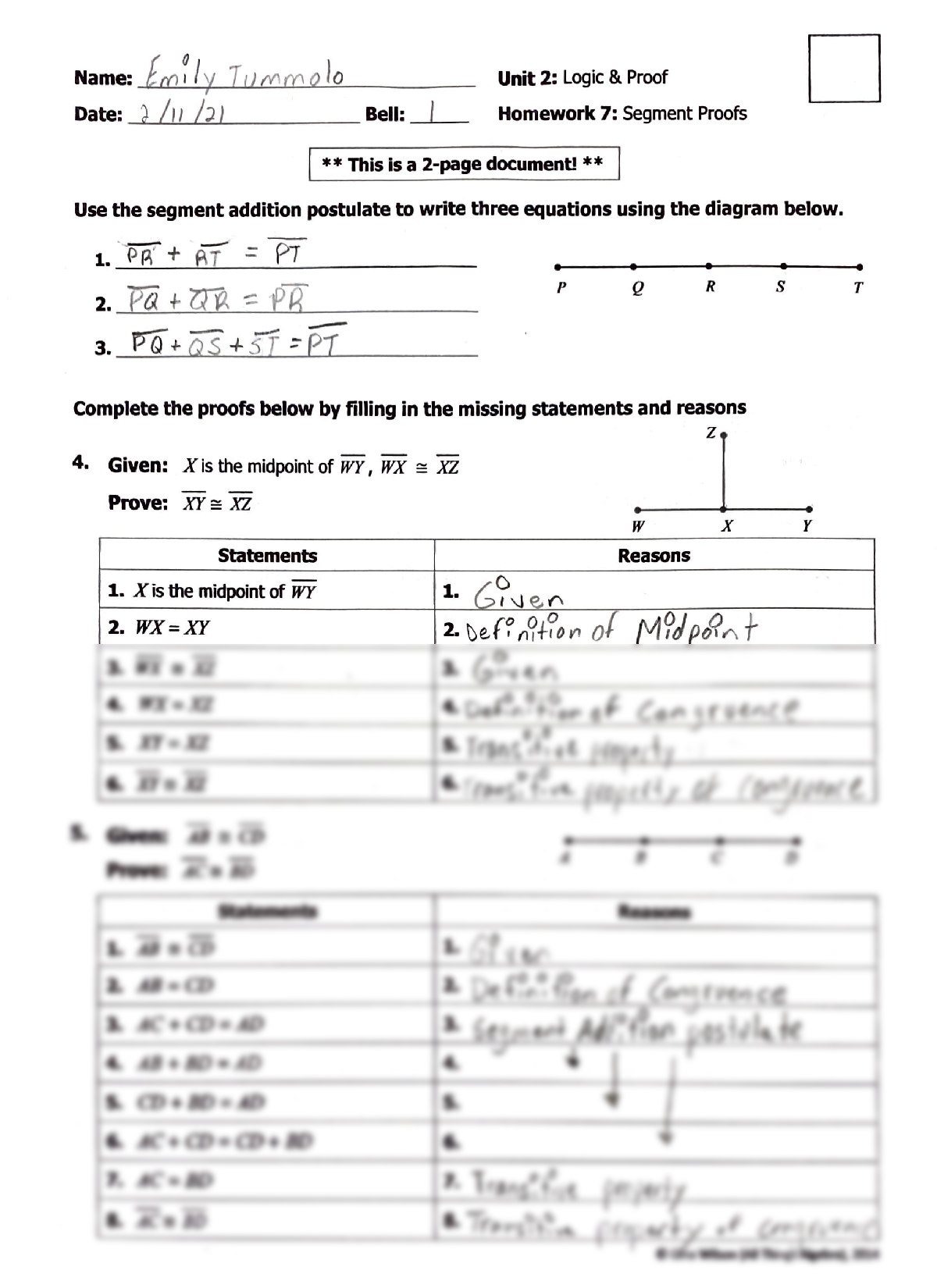
5 Essential Geometry Problems Solved: Segments and Angles

The study of geometry has captivated mathematicians for thousands of years, and the basic tenets of lines, segments, and angles continue to form the backbone of this elegant discipline. Today, we're going to explore five essential geometry problems, focusing on segments and angles, providing not just solutions, but also insights into why these problems matter. Here are the problems we'll address:
Problem 1: Midpoint Theorem

The Midpoint Theorem states that the line segment joining the midpoints of two sides of a triangle is parallel to the third side and half its length.
Proof: Consider triangle ABC with D and E as midpoints of AB and AC respectively.
- Draw line DE.
- Extend DE to F so that E is the midpoint of DF.
- Using congruent triangles and the SAS (Side-Angle-Side) axiom, prove that triangle ADE is similar to triangle ABC.
- Since DE is parallel to BC, DE is half the length of BC due to the similarity ratio.
📝 Note: This theorem helps understand how dividing a triangle can create similar triangles, which is fundamental in coordinate geometry and linear transformations.
Problem 2: Angles in a Semicircle
Any angle inscribed in a semicircle is a right angle (90°).
Explanation:
- Consider a diameter AB of a circle and point C on the semicircle.
- Triangle ACB is right angled at C because the angle subtended by the diameter at the circumference is always 90°.
- This can be proven using the Thales Theorem, which states that the angle subtended by a diameter of a circle is 90°.
💡 Note: This property is used in circle geometry problems to find missing angles or to verify the right angled triangle's properties.
Problem 3: Perpendicular Bisectors

The perpendicular bisector of a segment does not only cut the segment into two equal parts but also equidistant from both ends of the segment.
Solution:
- Construct a line perpendicular to AB at its midpoint M.
- Any point on this line is equidistant from A and B due to the properties of congruence in congruent triangles.
📐 Note: Understanding perpendicular bisectors is crucial for problems involving symmetry and finding centers of triangles or circles.
Problem 4: Exterior Angles and the Sum of Interior Angles

An exterior angle of a polygon is equal to the sum of the opposite non-adjacent interior angles. For a triangle, this means an exterior angle equals the sum of the two non-adjacent interior angles.
Explanation:
- Given triangle ABC with exterior angle at C, the exterior angle equals angle A plus angle B.
- This can be derived from the fact that the sum of the interior angles of a triangle is 180°, and the exterior angle forms a linear pair with one of the interior angles.
Problem 5: Central and Inscribed Angles

The measure of a central angle is twice the measure of an inscribed angle subtending the same arc.
Proof:
- Consider a circle with centre O and arc AB subtended by angles AOB (central) and ACB (inscribed).
- Using the properties of isosceles triangles and congruent arcs, show that angle AOB is twice angle ACB.
⚠️ Note: This relationship is a fundamental concept in circular arc length calculations and in trigonometry.
Geometry, with its foundational principles of segments, angles, and their relationships, provides us not just with mathematical beauty but also with practical applications in architecture, engineering, computer graphics, and beyond. The problems discussed here are not just about solving for unknown values; they are windows into the interconnected nature of geometric principles. Each theorem builds upon previous knowledge, creating a rich tapestry of logical connections.
Why is geometry important for students?

+
Geometry teaches logical reasoning and problem-solving skills, which are crucial in many fields including physics, engineering, and computer science.
How can understanding angles in a semicircle be applied in real life?

+
It’s used in architectural design, particularly in building domes or arches, where the curvature of the structure creates right angles at specific points.
What are some common mistakes students make when dealing with exterior angles?

+
One common error is forgetting that an exterior angle of a triangle is supplementary to one of its interior angles, leading to miscalculations in solving problems.