7 Tips for Mastering Segment Addition Postulate

The Segment Addition Postulate is an elementary but essential concept in geometry that can significantly enhance your understanding of lengths, distances, and positions within a plane. This principle, which states that if a point B lies on the line segment AB, then AB = AB + BC, where point C is another point on AB beyond B, forms the basis for more complex geometric constructions. Here are seven tips to master this postulate and apply it effectively in geometric problem-solving:
Understand the Basics

Before diving into complex applications, ensure you understand the basics:
- The segment addition postulate applies when three points are collinear.
- The point you’re considering must lie between the other two points.
📌 Note: It's easy to confuse the Segment Addition Postulate with the Betweenness of Points. While related, they are not the same. The Segment Addition Postulate specifies addition of lengths on a single line.
Visualize the Line Segment

Imagine the line segment visually to better understand where the points are positioned:
- Draw line AB with B somewhere in between A and C.
- Recognize that AB is composed of the two smaller segments, AB and BC.

Recognize Common Pitfalls

Here are some common mistakes to avoid:
- Not realizing that the points must be collinear.
- Confusing the point of reference for addition (which must always be the same point).
⚠️ Note: Points must be aligned on the same line for the Segment Addition Postulate to apply.
Practice with Real-World Examples

Connect the postulate to real-life scenarios:
- Consider a straight road with three points representing your house (A), a store (B), and a gas station ©.
- If you want to find the distance from your house to the gas station, you would use the Segment Addition Postulate.
Use Algebraic Equations

To apply the postulate in problem-solving:
- Set up equations where lengths are represented by variables. For example, if AB = x, BC = y, then AC = x + y.
- Solve for the unknown variables using the given information.
Explore Extensions of the Postulate
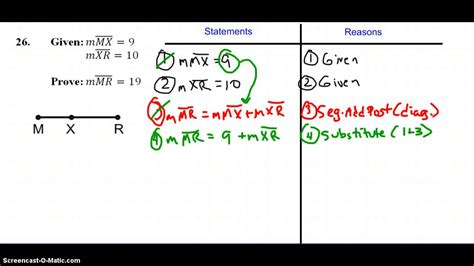
Look into how the Segment Addition Postulate connects with other geometry concepts:
- Consider the Subtraction Postulate where if point B is between A and C, then AC = AB - BC.
💡 Note: The Segment Addition Postulate can be reversed to understand subtraction in a line segment.
Problem Solving with Multiple Segments

When dealing with more complex problems:
- Divide a long segment into smaller segments using intermediate points.
- Apply the postulate repeatedly to find the total length or individual segment lengths.
By mastering these tips and applying them consistently, you'll not only grasp the Segment Addition Postulate but also enhance your overall geometric problem-solving skills. This knowledge will serve as a foundation for tackling more advanced geometric concepts and problems, ensuring you have a solid base to build upon.
To wrap up, understanding and mastering the Segment Addition Postulate can simplify many geometric problems, providing a clear and logical framework for thinking about lengths, distances, and spatial relationships. Keep practicing with different scenarios, and remember that geometry is about connecting abstract concepts to tangible realities, making it both fascinating and functional.
What is the Segment Addition Postulate?

+
The Segment Addition Postulate states that if point B lies on segment AC, then the length of segment AC is the sum of the lengths of segments AB and BC.
Does the Segment Addition Postulate apply only to lines?

+
No, it applies to any collinear points. The key requirement is that the points lie on the same straight line or path.
Can the Segment Addition Postulate be used in three-dimensional space?

+
Yes, as long as the points are collinear, the Segment Addition Postulate remains valid in three-dimensional space.
Is there a Segment Subtraction Postulate?

+
While not explicitly called a “postulate,” if point B lies on segment AC and C is past B, then AC can be expressed as AB minus BC.
How does understanding this postulate help in solving geometry problems?
+
It provides a framework for solving problems involving lengths of line segments, which is fundamental in understanding distance, position, and geometric constructions.