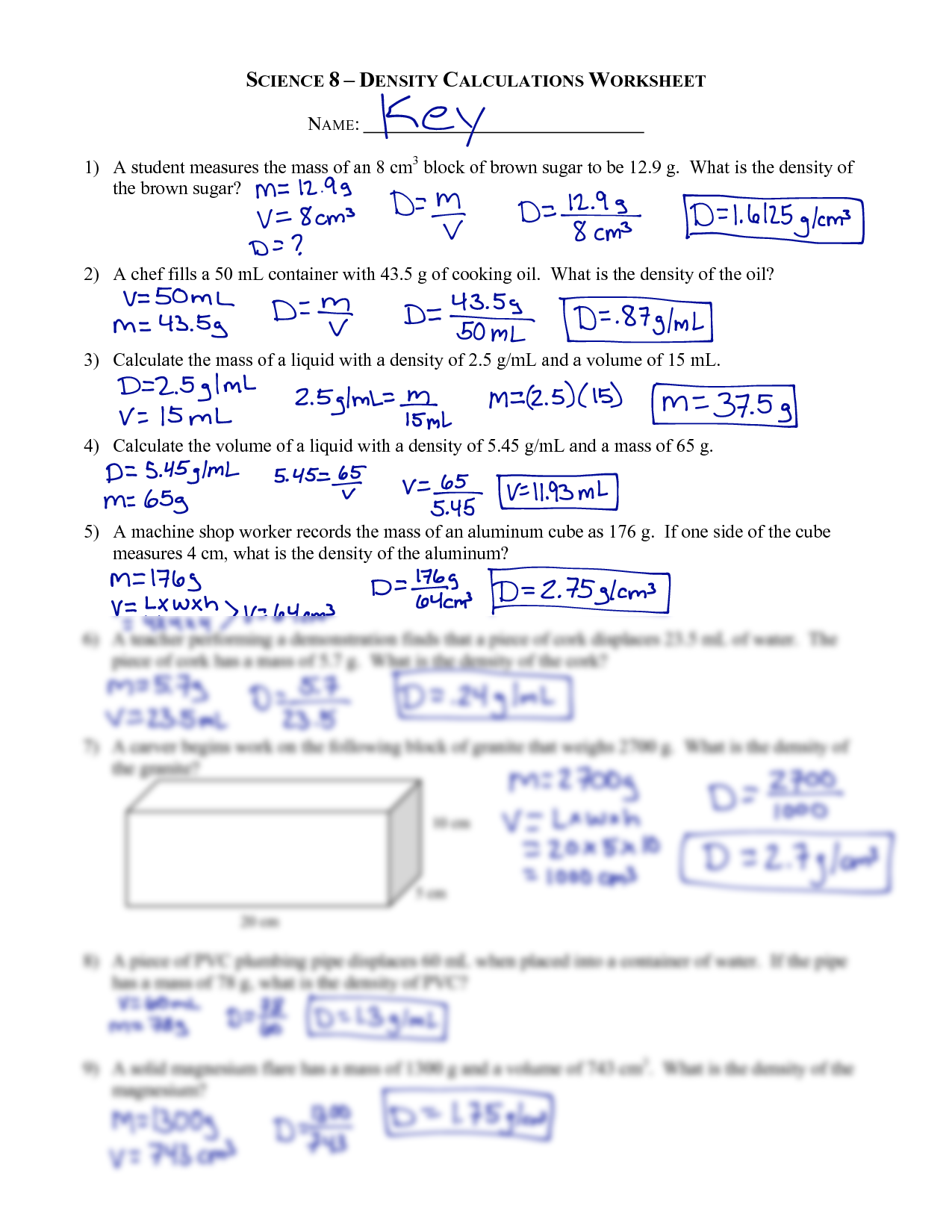
5 Easy Steps for Science 8 Density Calculations
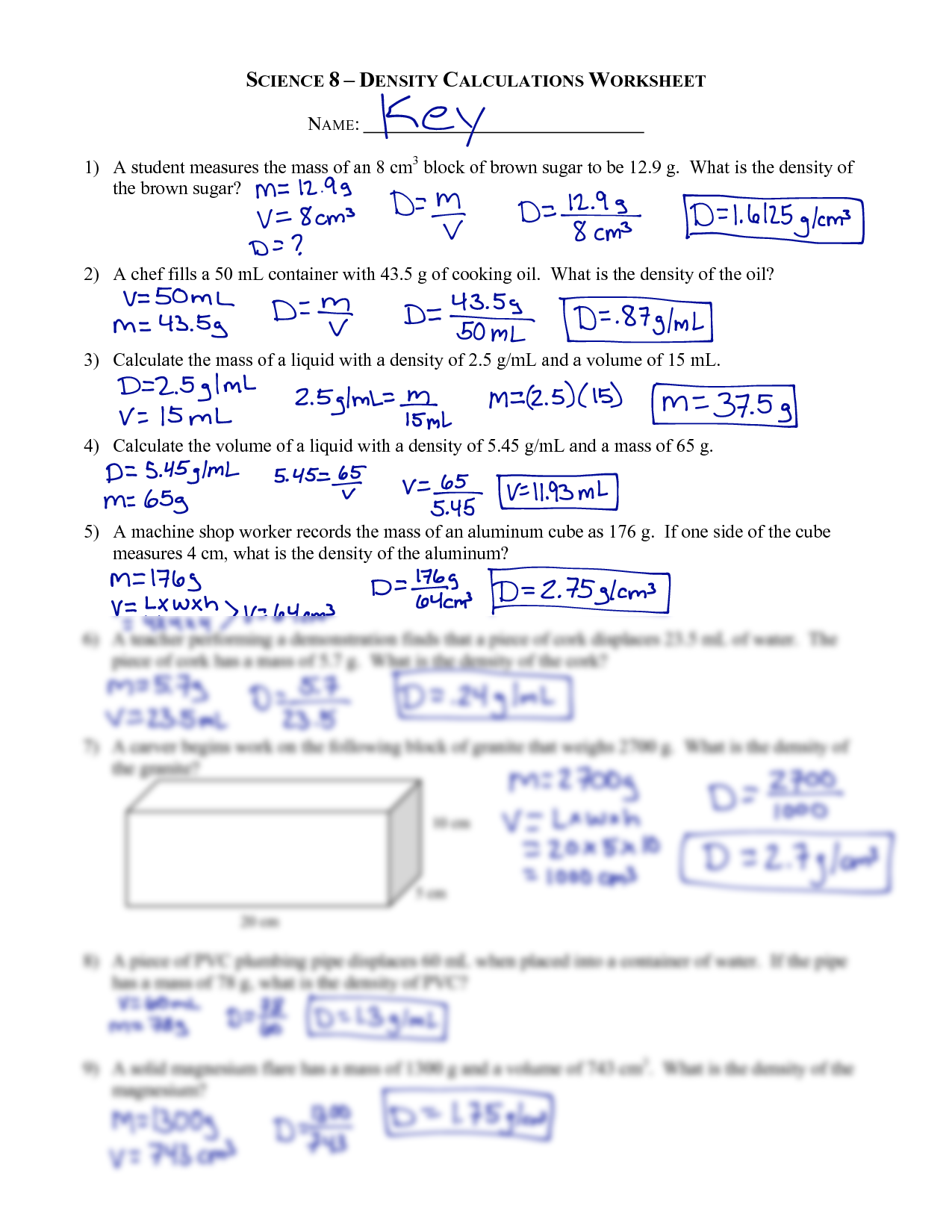
Density is a fundamental concept in physical science, crucial for understanding how different materials interact under various conditions. Here are five easy steps to perform density calculations in your Science 8 class:
Step 1: Understand Density
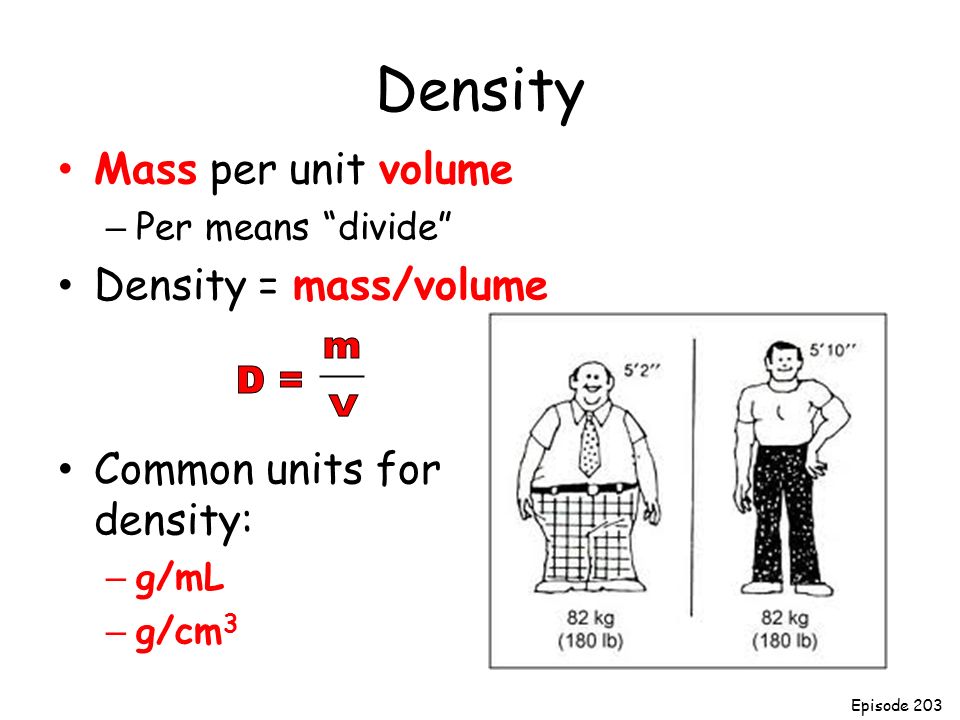
First and foremost, density is defined as the mass of a substance per unit volume. The formula to calculate density is:
[ \text{Density} = \frac{\text{Mass}}{\text{Volume}} ]This relationship can be expressed using the Greek letter rho (ρ) for density:
[ \rho = \frac{m}{V} ]
- Mass (m) is typically measured in grams (g) or kilograms (kg).
- Volume (V) is measured in cubic centimeters (cm3) or liters (L).
- Density (ρ) is commonly expressed in grams per cubic centimeter (g/cm3).
Step 2: Measure Mass

To calculate density, begin by measuring the mass of the object you’re analyzing. Use a balance or scale to get an accurate mass measurement:
- Place the object on the balance or scale.
- Read the mass in grams (g) or kilograms (kg).
Step 3: Measure Volume
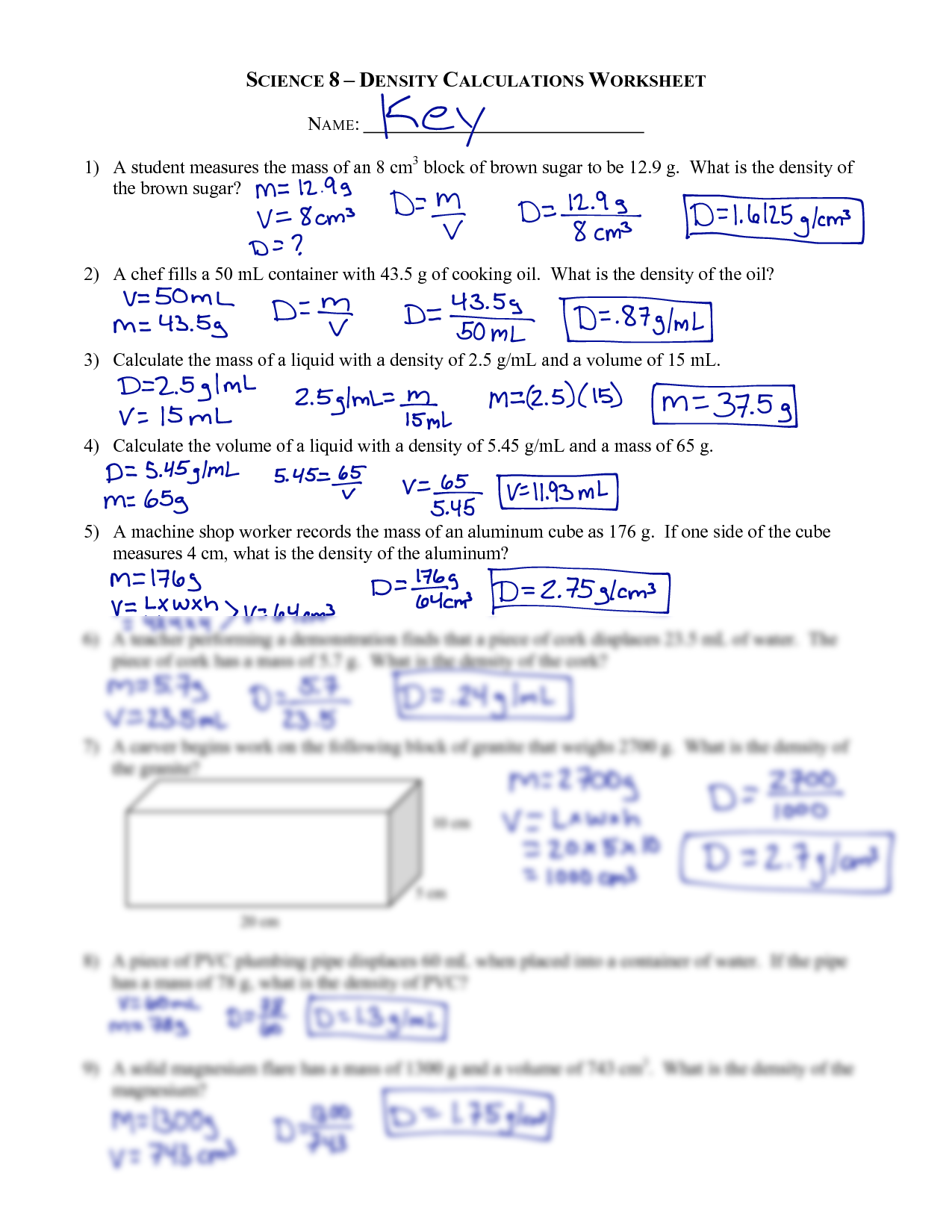
Now measure the volume:
- For regular geometric shapes, use the appropriate volume formula:
- Cube: ( V = \text{side}^3 )
- Rectangular prism: ( V = \text{length} \times \text{width} \times \text{height} )
- Cylinder: ( V = \pi \times \text{radius}^2 \times \text{height} )
- For irregular shapes, use the water displacement method:
- Fill a graduated cylinder or container with a known volume of water.
- Note the initial volume of water.
- Gently submerge the object into the water, ensuring no air bubbles are trapped.
- Read the new volume of water and subtract the initial volume to get the object’s volume.
💡 Note: When measuring volume using displacement, ensure the object is completely submerged to avoid inaccurate measurements.
Step 4: Calculate Density
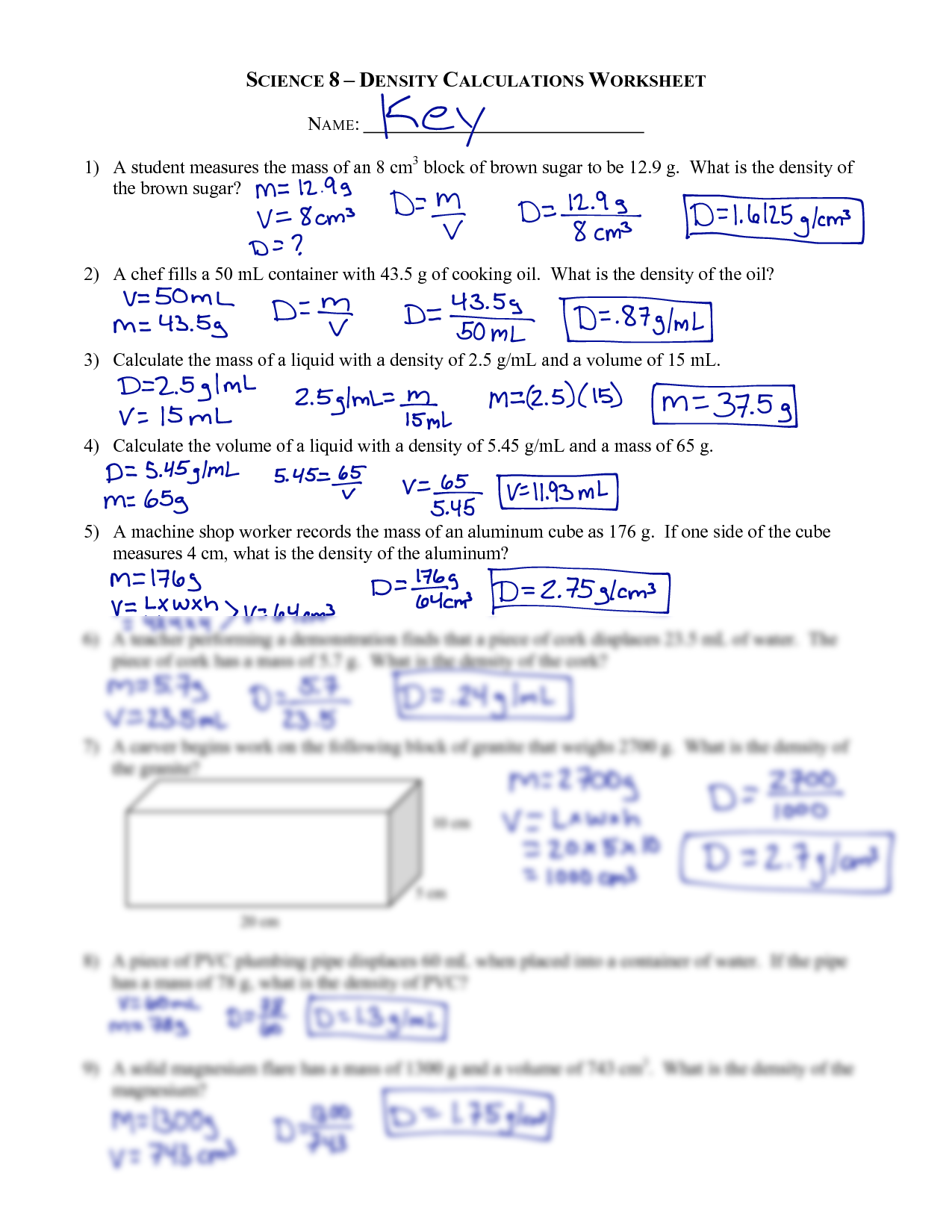
Once you have the mass and volume:
- Divide the mass by the volume to get the density using the formula: [ \rho = \frac{m}{V} ]
- Ensure your units are consistent.
For example, if an object has a mass of 50 grams and a volume of 10 cm3, its density would be:
\[ \rho = \frac{50 \, \text{g}}{10 \, \text{cm}^3} = 5 \, \text{g/cm}^3 \]Step 5: Interpret Results

Understanding what your calculated density means:
- Density can help identify materials or substances. For instance, the density of water at 4°C is approximately 1 g/cm3. If an object has a density less than this, it will float; if more, it will sink.
- Density is useful in various applications from engineering to environmental science, aiding in understanding buoyancy, material selection, and more.
| Substance | Density (g/cm3) |
|---|---|
| Water | 1.00 |
| Iron | 7.87 |
| Gold | 19.30 |
| Alcohol (Ethanol) | 0.79 |

To sum up, performing density calculations involves understanding the concept, measuring mass and volume accurately, applying the formula, and interpreting the results effectively. These steps help you in mastering density calculations, an essential skill in physical science.
When practicing density calculations, keep these points in mind:
How do I ensure the accuracy of my measurements?
+
Ensure the balance or scale is properly calibrated. For volume, make sure no air bubbles are trapped when using the displacement method.
Why is density important?

+
Density helps in identifying substances, determining buoyancy, and understanding material properties for engineering and scientific applications.
Can the density of a substance change?
+
Yes, the density of a substance can change with temperature and pressure. For instance, water’s density changes when it freezes or boils.
What is the difference between density and specific gravity?

+
Specific gravity is the ratio of the density of a substance to the density of a reference substance (usually water at 4°C). While density is an absolute measure, specific gravity is a dimensionless quantity.