Rotations on Coordinate Plane Worksheet Key: Mastering Math!
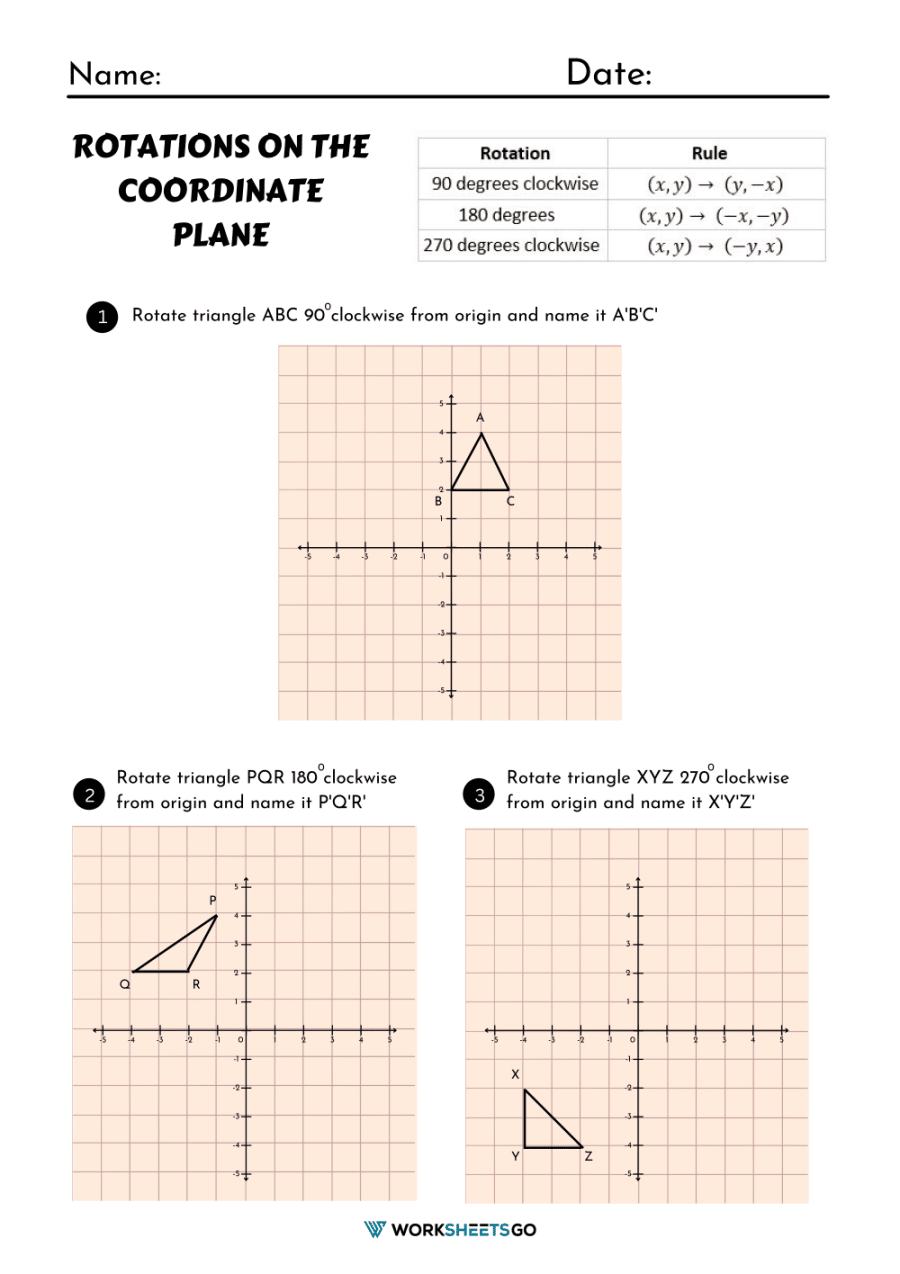
Understanding how to rotate points, shapes, and angles around the coordinate plane is a fundamental skill in geometry. Whether you're a student preparing for an exam, a teacher looking to create instructional material, or someone who enjoys mathematics for its own sake, mastering rotations is an essential part of spatial reasoning. In this comprehensive guide, we'll dive deep into rotations, provide worksheets with key answers, and ensure that you feel confident in your ability to handle any rotation problem that comes your way.
The Basics of Rotations

Before we get into the nitty-gritty of our worksheet, let's go over the basics of rotations on the coordinate plane:
- Definition: A rotation is a transformation in which a figure or point moves around a fixed point known as the center of rotation.
- Angles of Rotation: Common rotations are 90°, 180°, and 270°, but you can rotate a figure by any angle.
- Direction: Rotations can be clockwise or counterclockwise. A positive angle is typically counterclockwise.
- Rotation Rules:
- 90° counterclockwise (or 270° clockwise): (x, y) → (-y, x)
- 180° rotation: (x, y) → (-x, -y)
- 270° counterclockwise (or 90° clockwise): (x, y) → (y, -x)
📝 Note: Always remember that for a rotation around the origin, the rotation angle determines how the coordinates change.
Worksheet Example

Let's explore some practical examples to apply our knowledge of rotations:
Rotate Point A: Given Point A at coordinates (3, 4), what are the new coordinates if it is rotated:
- 90° counterclockwise around the origin?
- 180° around the origin?
- 270° counterclockwise around the origin?
| Initial Coordinates | 90° Counterclockwise | 180° | 270° Counterclockwise |
|---|---|---|---|
| (3, 4) | (-4, 3) | (-3, -4) | (4, -3) |

Rotating Shapes

When rotating shapes, we use the same rules for individual points but must consider all vertices:
Example: Rotate the triangle with vertices A(1,1), B(2,3), and C(4,1) by 90° counterclockwise around the origin:
- A(1,1) becomes A'(-1,1)
- B(2,3) becomes B'(-3,2)
- C(4,1) becomes C'(-1,4)
This creates a new triangle with vertices A'(-1,1), B'(-3,2), and C'(-1,4).
Key Points to Remember

- The center of rotation does not always have to be the origin. You can rotate around any point.
- If the rotation is not around the origin, you may need to translate the shape first, perform the rotation, and then translate it back.
- Make sure to check the direction of rotation; counterclockwise is standard in many mathematical contexts.
💡 Note: The sign of the coordinates will change with certain rotations. Always keep track of whether you are moving from positive to negative or vice versa.
As we conclude our exploration into rotations on the coordinate plane, it's clear that understanding and applying these transformations are not only crucial for geometry but also for a wide array of mathematical disciplines and real-world applications. Whether it's in computer graphics, engineering, or even everyday problem-solving, knowing how to rotate points and shapes provides a powerful tool for analysis and design.
What is the difference between a clockwise and counterclockwise rotation?

+
A clockwise rotation turns a figure in the same direction as the hands of a clock. Counterclockwise rotation, conversely, turns in the opposite direction. Standard mathematical rotations are generally counterclockwise unless specified otherwise.
How do I rotate around a point other than the origin?

+
Rotate around another point by first translating the point or shape so that the point of rotation becomes the origin, then apply the rotation, and finally translate back.
Can rotations change the shape or size of an object?

+
No, rotations are rigid motions in geometry, meaning they do not change the size or shape of an object, only its orientation and position.
Related Terms:
- Rotation worksheet with answers PDF
- Rotations Worksheet answer Key
- Geometry Rotations Worksheet pdf