5 Tips for Mastering Rotation Reflection Translation Worksheets

Mastering transformation geometry can be a challenging yet rewarding experience. Rotation, reflection, and translation, the trio of transformations, are essential concepts that students of geometry must master to excel in math. These concepts not only have profound implications in mathematical theory but also have real-world applications in fields like robotics, animation, and video game design. Here, we delve into five tips to help students conquer rotation, reflection, and translation worksheets with confidence and precision.
1. Understand the Basics

Before you dive into complex problems, ensure you have a strong grasp of the fundamental concepts. Here's what you need to know:
- Rotation: Involves turning a figure around a fixed point, which we call the center of rotation. Degrees are used to measure how far the object turns.
- Reflection: Creating a mirror image of a figure over a line known as the line of reflection.
- Translation: Sliding a figure without changing its orientation or size. It's described by a set of distance and direction (often a vector).
To solidify these concepts:
- Visualize or draw these transformations. Use graph paper to practice rotations, reflections, and translations.
- Recognize and understand how each transformation alters a figure's position, shape, and orientation.
2. Utilize Tools and Visual Aids

Geometry is a visual subject. Here's how you can leverage tools to enhance your learning:
- Graph Paper: Use graph paper to plot points and perform transformations manually. It helps in visualizing the changes in position and orientation accurately.
- Tracing Paper: Place a piece of tracing paper over a figure to practice rotations or reflections by tracing the original figure and then moving the tracing paper as needed.
- Geometry Software: Applications like Geogebra or Desmos can simulate these transformations dynamically. This interactive approach allows for a deeper understanding through experimentation.
These tools not only aid in accuracy but also provide an opportunity to explore different scenarios, enhancing your understanding and problem-solving skills.
3. Master the Vocabulary

Transformation geometry has its own set of terms and symbols. Here are some key words you need to be familiar with:
| Term | Definition |
|---|---|
| Line of Symmetry | A line where a figure is symmetric; reflections across this line produce identical images. |
| Center of Rotation | A fixed point around which a figure rotates. |
| Translation Vector | A vector that describes the direction and distance a figure moves during a translation. |
| Orientation | The way in which a figure is positioned or oriented relative to a coordinate plane or other figures. |

Remembering these terms will not only help in understanding questions but also in describing transformations accurately in both written and oral communication.
4. Practice with Varied Problems
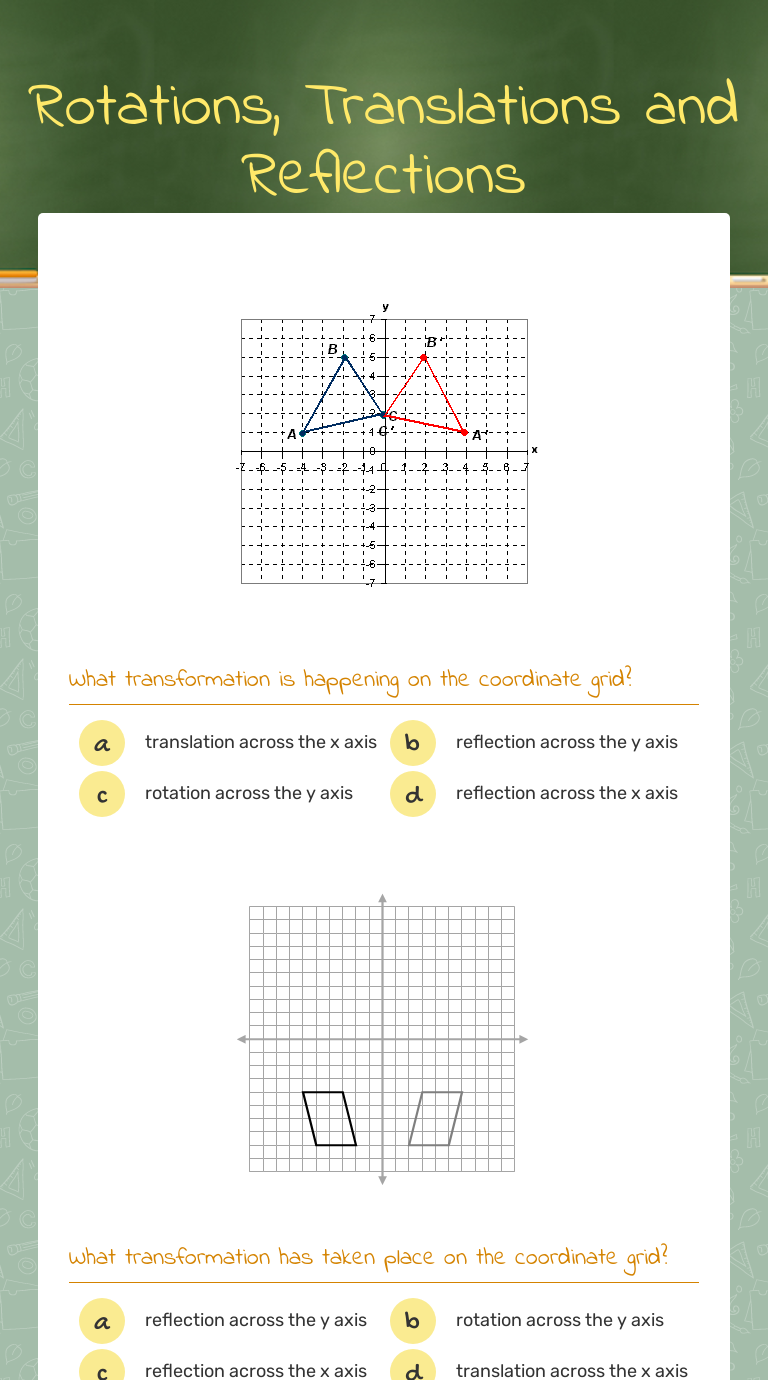
Transformation worksheets often come with different types of questions:
- Simple Transformations: Where a figure is rotated, reflected, or translated once. Start with these to build confidence.
- Multiple Transformations: Problems where figures are subjected to multiple transformations, either in sequence or combined. This tests your ability to maintain accuracy through several steps.
- Identification and Verification: Determining which transformation has taken place or verifying whether a given set of transformations can produce an image.
Here are some strategies for effective practice:
- Begin with single-step problems to reinforce your foundational knowledge.
- Gradually introduce more complex scenarios where multiple transformations need to be applied.
- Use color coding or labeling in your solutions to keep track of the original figure and transformed images.
📝 Note: Always double-check your work by reverse transforming or using an alternative method to verify the final image.
5. Reflect and Learn from Mistakes

Mistakes are a part of learning, especially in transformation geometry where precision is key. Here's how to learn from your errors:
- Review Mistakes: When you make a mistake, don't just move on. Understand why it happened. Was it a conceptual error, a calculation mistake, or perhaps an oversight in setting up the problem?
- Ask for Feedback: If possible, consult with a teacher or peers to get an external perspective on your solutions and identify common pitfalls.
- Learn Patterns: Over time, you'll notice patterns in your mistakes. If you consistently struggle with a particular transformation, focus on practicing that one until you're confident.
- Keep a Log: Document your progress, including mistakes and corrections. This log can serve as a reference for future study sessions or when you need to prepare for an exam.
Remember, the journey of learning geometry is a marathon, not a sprint. Each misstep is an opportunity to grow and refine your understanding.
In conclusion, mastering rotation, reflection, and translation worksheets requires patience, practice, and a keen eye for detail. By understanding the basics, utilizing visual aids, mastering the vocabulary, practicing with varied problems, and reflecting on your mistakes, you’ll be well on your way to excelling in transformation geometry. These strategies not only help in tackling worksheets but also equip you with skills applicable to advanced mathematical concepts and real-world applications.
What is the difference between rotation and reflection?

+
Rotation involves turning a figure around a fixed point, keeping the same orientation. Reflection, on the other hand, creates a mirror image of the figure over a line, which changes its orientation.
How do I perform a translation on a coordinate plane?
+
To perform a translation, you add the horizontal and vertical components of the translation vector to each point of the figure. For example, if the translation vector is (3, -2), you would move every point 3 units to the right and 2 units down.
Why is understanding transformations important in geometry?

+
Understanding transformations allows you to visualize and manipulate geometric shapes, which is fundamental in fields like architecture, engineering, animation, and more. It also helps in problem-solving by providing different perspectives and methods to approach spatial questions.