5 Easy Steps to Master Arithmetic Sequence Formulas

Learning arithmetic sequences is fundamental in both algebra and mathematics, as it provides a clear method for understanding patterns and predicting values. This guide will demystify arithmetic sequences through a practical approach, ensuring that you can easily master their formulas and apply them effectively in real-world scenarios.
Understanding Arithmetic Sequences

Arithmetic sequences are a series of numbers where the difference between each consecutive term remains constant. This constant difference, known as the common difference, is crucial for all calculations involved.
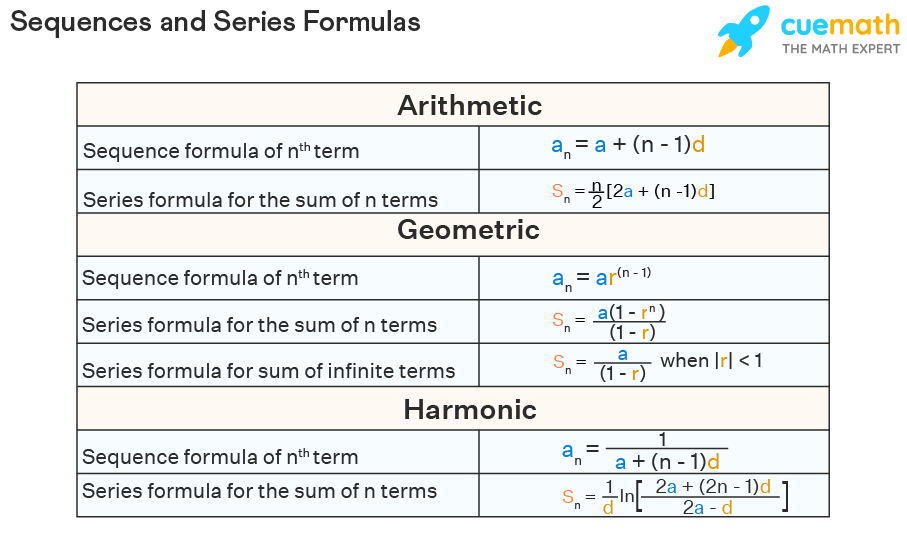
- Formula for the n-th term: (a_n = a_1 + (n-1)d)
- Sum of the first n terms: (S_n = \frac{n}{2} (a_1 + a_n))
Step 1: Identify the First Term and Common Difference


- Locate (a_1), the first term, from the sequence provided.
- Find the common difference (d) by subtracting any term from the preceding term, (d = a_2 - a_1).
🔹 Note: The common difference d can be positive, negative, or zero. Ensure to maintain its sign during calculations.
Step 2: Calculate the N-th Term

To find the value of any specific term:
- Use the formula (a_n = a_1 + (n-1)d), where (n) is the position of the term you need.
⚠️ Note: Remember to use parentheses to maintain the correct order of operations.
Step 3: Summing Up

Calculating the sum of the first (n) terms:
- Apply (S_n = \frac{n}{2} (a_1 + a_n))
- You can find (a_n) from the previous step or use (S_n = \frac{n}{2} (2a_1 + (n-1)d))
👉 Note: This formula is efficient for a quick summation of a large number of terms.
Step 4: Applying Sequence in Real Life
Arithmetic sequences appear in many practical situations:
- Financial planning: Monthly savings, loan amortization, or investment growth.
- Geometry: Regular polygonal vertices, equidistant points.
- Physics: Uniform acceleration scenarios.
Step 5: Practice and Mastery

Mastery comes with:
- Problem Solving: Work on numerous arithmetic sequence problems.
- Online Resources: Utilize educational sites and video tutorials for dynamic learning.
| Tool | Use |
|---|---|
| Scientific Calculator | Calculates terms and sums quickly. |
| Spreadsheets | Set up formulas to analyze long sequences. |

In summary, arithmetic sequences are integral for pattern recognition, prediction, and problem-solving in mathematics and beyond. From understanding the simple arithmetic progression to applying these concepts in various real-world contexts, you now have the tools to confidently tackle arithmetic sequences. Remember, consistent practice is the key to truly mastering these mathematical sequences.
What is a common difference in arithmetic sequence?
+
The common difference in an arithmetic sequence is the fixed value between each pair of consecutive terms. It’s denoted as (d).
Can the common difference be zero?

+
Yes, but if the common difference (d) is zero, the sequence would remain the same throughout, producing a constant sequence, not an arithmetic one.
How can I recognize an arithmetic sequence?

+
An arithmetic sequence can be identified if the difference between any two consecutive terms is the same, indicating a consistent pattern.



