5 Key Answers for Geometry Worksheet: Rectangles, Rhombuses, Squares
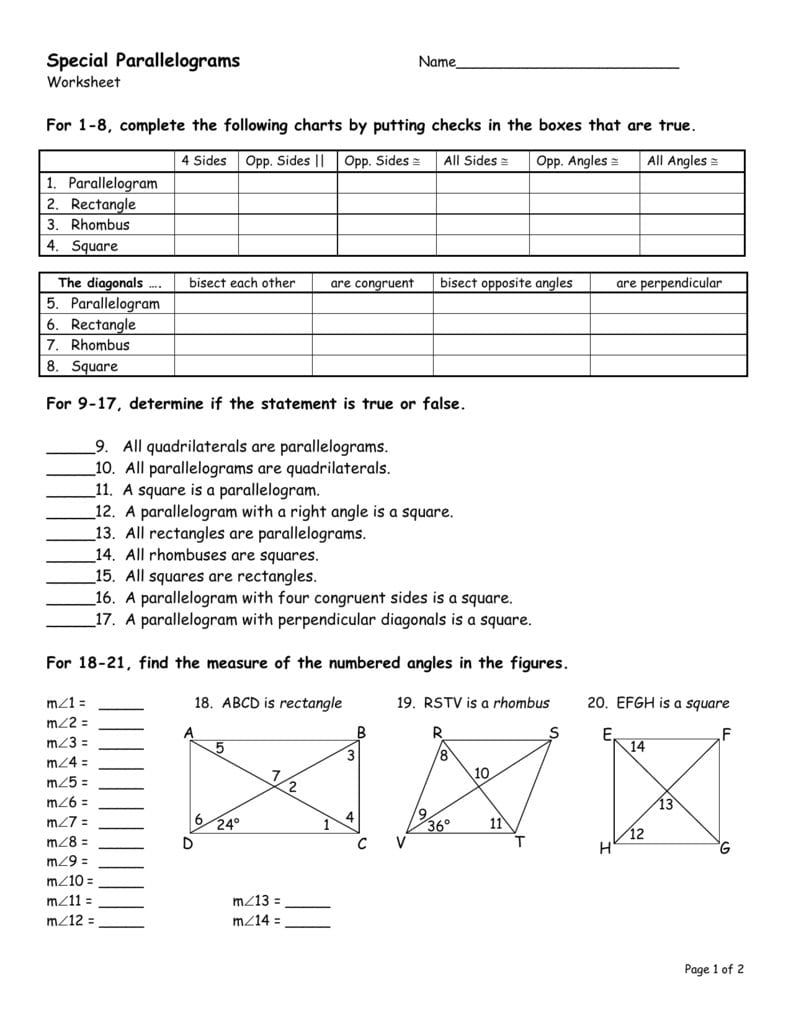
In the world of geometry, rectangles, rhombuses, and squares play a pivotal role due to their symmetrical properties and wide application in real-world scenarios. Understanding these shapes not only boosts one's proficiency in geometry but also enhances problem-solving skills applicable in various fields. Here, we will delve into five key answers related to these shapes, offering a deep dive into their properties and how they can be applied in problem-solving.
Key Properties of Rectangles

A rectangle is a quadrilateral where all angles are right angles (90 degrees). Here are some essential properties:
- Opposite sides are congruent: This means that if one side measures 5 cm, its opposite side will also be 5 cm.
- Diagonals are equal in length: The length of the diagonal (d) in a rectangle with sides a and b can be calculated using the Pythagorean theorem, d = √(a² + b²).
- Perpendicular diagonals: Diagonals in a rectangle cross at right angles, bisecting each other.
- Equal sum of opposite angles: Each pair of opposite angles adds up to 360 degrees (4 right angles).
📝 Note: Although the Pythagorean theorem applies, it's specifically useful when dealing with diagonals in rectangles or squares.
Understanding Rhombuses

A rhombus is a special case of a parallelogram where all sides are of equal length. Here's what you need to know:
- All sides are congruent: If one side of a rhombus measures 7 units, then all sides are 7 units long.
- Opposite angles are equal: A rhombus can have different angles, but opposite angles are always equal.
- Diagonals bisect each other at right angles: Like in squares, diagonals of a rhombus also bisect at 90 degrees.
- The sum of its angles equals 360 degrees: Each pair of adjacent angles in a rhombus add up to 180 degrees.
📝 Note: Rhombuses have no right angles unless they are also squares.
Exploring Squares

A square is a special rectangle where all sides are equal, and all angles are right angles. Here's an overview:
- All sides are congruent: Much like a rhombus but with added right angles.
- Diagonals are equal in length: Diagonals in a square are congruent and bisect at right angles.
- All angles are right angles: Similar to rectangles, but with the added symmetry of equal sides.
- The diagonals create four right triangles: Each triangle has legs equal to the side of the square and hypotenuse equal to the diagonal.
| Shape | Equal Sides | Equal Angles | Right Angles | Diagonal Properties |
|---|---|---|---|---|
| Rectangle | Opposite sides | All angles | Yes | Equal length |
| Rhombus | All sides | Opposite angles | No, unless it's a square | Bisect at right angles |
| Square | All sides | All angles | Yes | Bisect at right angles, Equal length |

Application in Problem Solving

Understanding these properties facilitates solving geometry problems. Here's how:
- Finding the area: For a rectangle or square, area = side × side or length × width. For a rhombus, area can be calculated as 1/2 × (d1 × d2), where d1 and d2 are the lengths of the diagonals.
- Determining perimeter: Sum of all sides. For a rectangle, 2(length + width); for a square or rhombus, 4 × side.
- Using Pythagorean theorem: For diagonal length in rectangles and squares.
- Symmetry and rotation: Squares have four lines of symmetry, rectangles two, and rhombuses two; this can help in geometric puzzles and constructions.
📝 Note: In real-world applications, understanding these shapes aids in designing, architecture, and even in everyday measurements.
Common Geometric Pitfalls

Here are some common mistakes people make when dealing with these shapes:
- Confusing Properties: Believing that all rhombuses have right angles or that all rectangles are squares.
- Incorrect Diagonal Use: Forgetting that diagonals in rectangles bisect at 90 degrees only if the rectangle is a square.
- Area Calculation Errors: Mixing up the formulas for area calculation in rhombuses, rectangles, and squares.
📝 Note: Always verify the shape you're dealing with before applying geometric properties or formulas.
In wrapping up this exploration, the distinct properties of rectangles, rhombuses, and squares provide a solid foundation for understanding geometry. Recognizing these shapes, their attributes, and how to apply their properties in problem-solving is crucial. From calculating areas and perimeters to understanding symmetry and using diagonals, these shapes offer a myriad of practical applications. The keys to mastering geometry lie in recognizing these properties and applying them correctly, which not only enriches one’s understanding of the subject but also equips one to tackle various real-world challenges.
What is the difference between a rectangle and a rhombus?

+
The main difference is that a rectangle has all angles as right angles, while a rhombus has all sides equal in length, but not necessarily right angles. A rhombus can be a square, but a rectangle cannot.
Can a rhombus have one right angle?

+
No, if a rhombus had one right angle, its opposite angle would also have to be right, making all angles right due to the properties of rhombuses, and thus it would be a square.
How do diagonals help in geometry?

+
Diagonals play a key role in geometry as they help determine properties like symmetry, angle bisectors, and even the shape of the quadrilateral. They can be used to find area, perimeter, and length when certain properties are known.



