5 Essential Exercises for Mastering Real Numbers

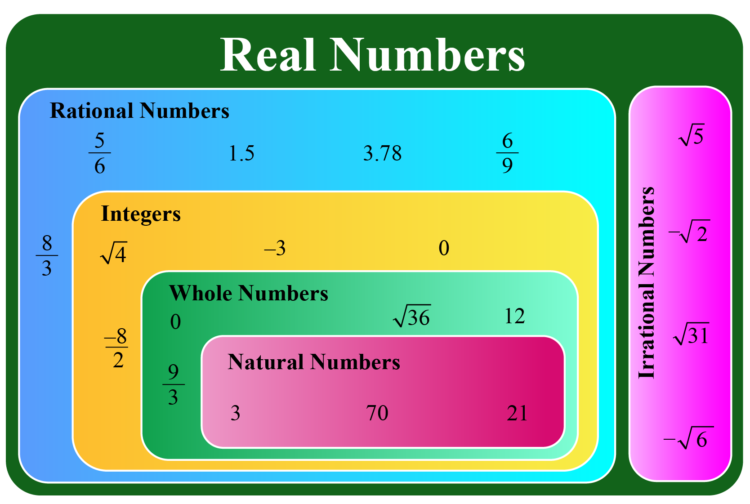
In the vast and often complex world of mathematics, understanding the foundation of numbers is crucial for progressing to higher levels of study and practical applications. Real numbers form the backbone of everyday calculations as well as advanced mathematical theories. To master real numbers, one must not only understand their definitions but also engage in exercises that deepen comprehension and apply these concepts effectively. Here, we'll explore five essential exercises designed to master the art of working with real numbers.
1. Number Line Visualization

One of the first steps in mastering real numbers is visualizing them on a number line. This exercise helps in understanding:
- Concept of Density: Real numbers are dense, meaning between any two real numbers, there is always another real number.
- Representation of Rational and Irrational Numbers: Visualizing how rational numbers like 1⁄2, 1⁄4, etc., and irrational numbers like √2 or π fit onto a number line.
- Continuity: Appreciating how real numbers form a continuous, unbroken line.
📝 Note: Ensure the number line is drawn with accurate intervals, especially for irrational numbers which cannot be precisely placed on a standard scale.
2. Comparing and Ordering Real Numbers

Developing the skill to compare and order real numbers, including both rational and irrational, is fundamental. This exercise involves:
- Ordering a mixed set of real numbers from smallest to largest.
- Using approximation techniques to compare numbers like √2 and 1.415.
- Understanding that comparisons between irrational numbers might require logical reasoning beyond simple arithmetic.
3. Operations with Real Numbers
Real numbers behave uniquely under arithmetic operations. Here’s how you can exercise:
- Addition and Subtraction: Practice operations involving both rational and irrational numbers.
- Multiplication and Division: Understand how these operations affect the magnitude and the type of the result (rational vs. irrational).
- Powers and Roots: Explore how these operations can create or simplify irrational numbers.
🔍 Note: When dealing with irrational numbers, results are often approximations, so learning to estimate or use scientific calculators is beneficial.
4. Real Number Properties and Proofs

This exercise focuses on understanding and proving properties like:
- Completeness Property: Every non-empty set of real numbers that has an upper bound has a least upper bound.
- Archimedean Property: For any real numbers x and y (with x positive), there exists a natural number n such that nx > y.
- Density of Rational Numbers: Between any two different real numbers, there is a rational number.
Practice writing proofs or providing counterexamples to deepen your understanding of these foundational properties.
5. Application of Real Numbers

Applying real numbers in practical problems or real-life scenarios:
- Use real numbers to solve equations or systems of equations.
- Model growth or decay in financial applications using real numbers.
- Explore geometry where lengths or angles might require the use of irrational numbers.
Understanding how real numbers behave in different contexts not only reinforces the theory but also their utility in the real world.
Mastering real numbers goes beyond mere theoretical knowledge. It involves visualizing, comparing, operating, proving, and applying these numbers in various contexts. Each exercise above serves as a stepping stone, building upon the others to create a comprehensive understanding. By engaging in these exercises regularly, you'll develop a deep and practical insight into real numbers, enabling you to navigate the complexities of mathematics with confidence and precision.
What is the difference between rational and irrational numbers?

+
Rational numbers can be expressed as a fraction, where both the numerator and denominator are integers (e.g., 1⁄2, 5⁄1). Irrational numbers cannot be expressed as such a fraction and have non-repeating, non-terminating decimal expansions (e.g., √2, π).
How can I visually compare √2 and 1.414?
+
Using a number line, you can mark 1.414 as a close approximation to √2. Although √2 is approximately 1.41421356, you can’t precisely mark it due to its infinite non-repeating nature. However, for comparison, you can use a series of approximations to show that √2 is slightly greater than 1.414.
Why is understanding real numbers important?

+
Real numbers are crucial because they are used to measure and model almost everything in the physical world. From distances, weights, to economic models, mastering real numbers provides the foundation for quantitative analysis in various scientific and engineering fields.