5 Ways to Master Rational Inequalities Easily
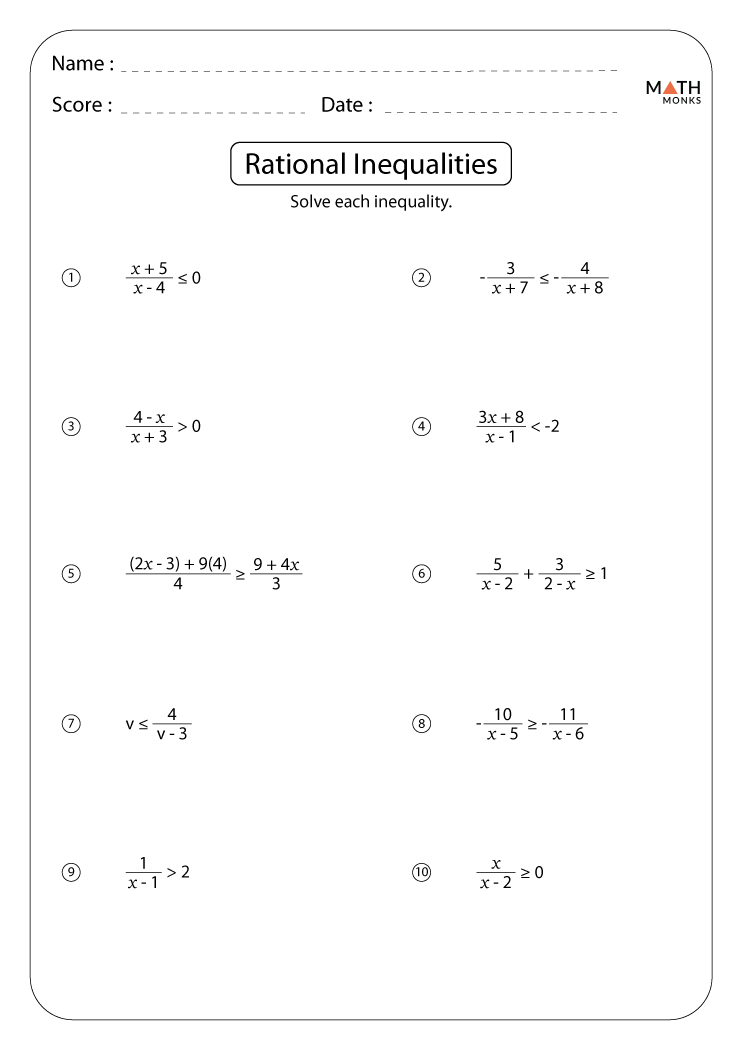
Solving rational inequalities can be a challenging task for students, but mastering them is not just about conquering a segment of algebra; it's about strengthening your problem-solving skills which are crucial in various mathematical and real-world applications. Whether you're looking to ace your upcoming exam or simply refine your mathematical prowess, understanding how to solve rational inequalities will equip you with valuable analytical skills. Here, we explore five straightforward methods that can help you master rational inequalities with ease.
1. Understand the Basics
Before diving into the complexity of rational inequalities, it’s critical to solidify your foundation in rational expressions and the concept of inequality.
- Rational Expressions: These are fractions where the numerator and denominator are polynomials. Understanding how to simplify and work with these expressions is key.
- Inequality: An expression involving a less than (<), greater than (>), less than or equal to (≤), or greater than or equal to (≥) sign.

💡 Note: Always simplify your rational expressions before attempting to solve an inequality, to avoid unnecessary complications.
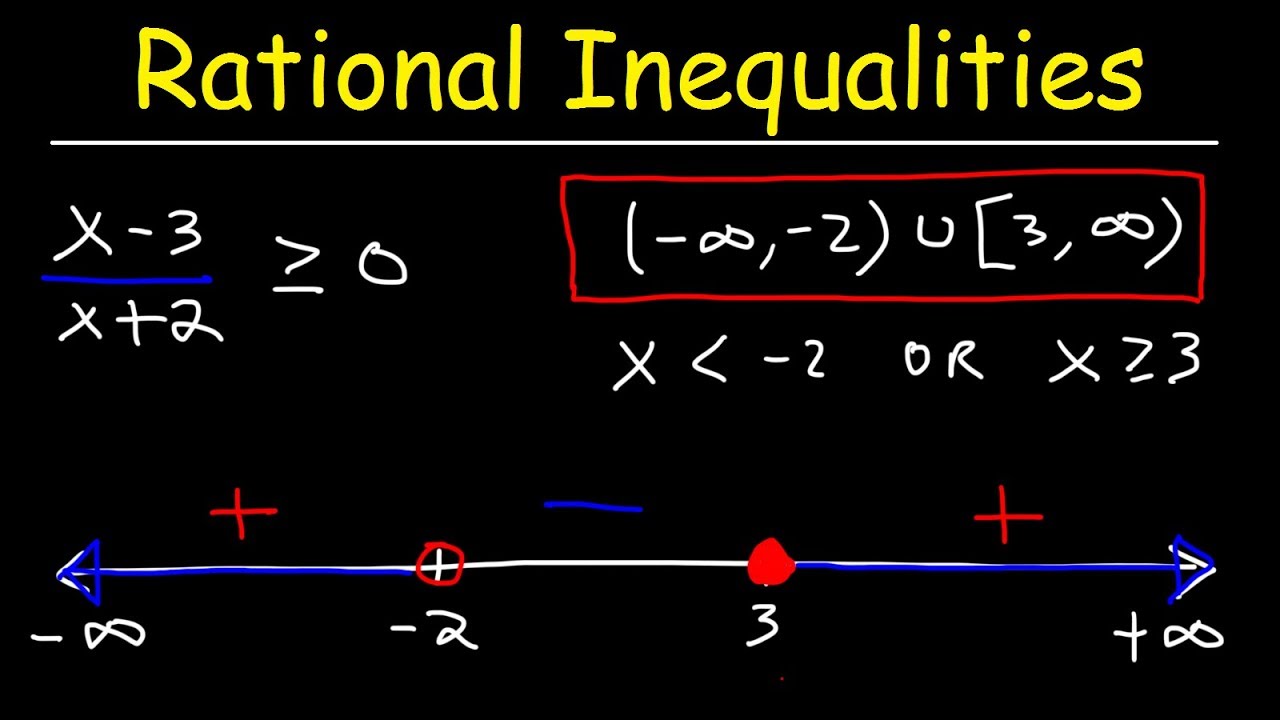
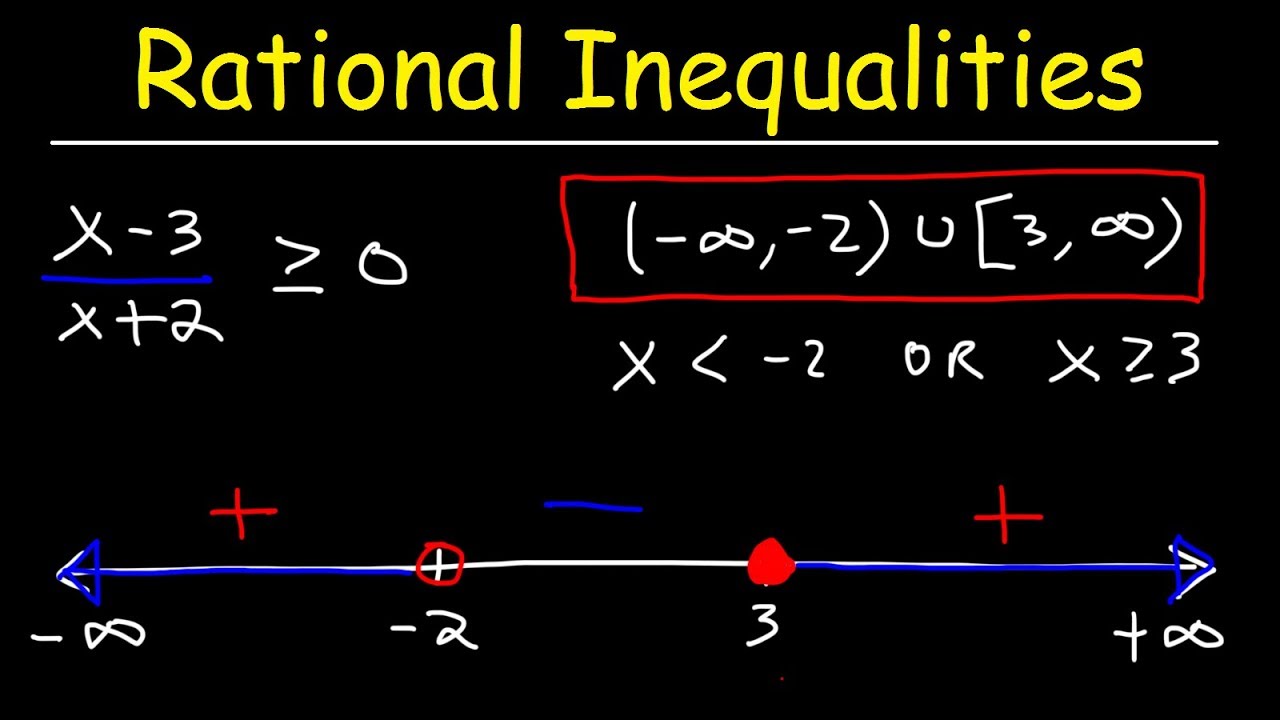
2. Identify Critical Points

To solve a rational inequality, you first need to identify the points where the rational expression changes its sign or becomes undefined:
- Set the numerator equal to zero to find the roots of the numerator.
- Set the denominator equal to zero to find where the expression is undefined.
These points, known as critical points, help divide the number line into intervals where the inequality holds true.
| Numerator | Denominator | Critical Points |
|---|---|---|
| x2 - 4 = 0 → x = ±2 | x - 3 = 0 → x = 3 | -2, 2, 3 |
3. Test Intervals
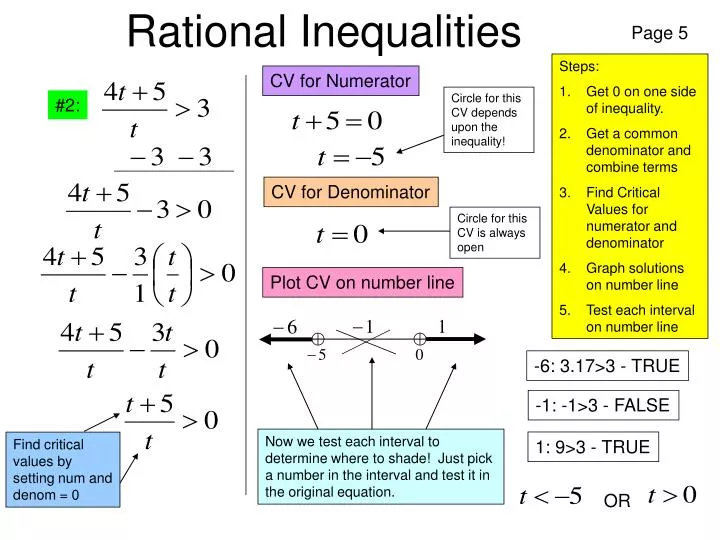
Once you have identified the critical points, you test each interval created by these points to see where the inequality is satisfied:
- Choose a test point from each interval.
- Substitute these test points into the simplified rational inequality.
- Determine if the inequality holds true within that interval.
By testing intervals, you effectively check the behavior of the function within those regions.
4. Consider the Behavior at Critical Points
After testing intervals, we must also consider the behavior of the function at the critical points:
- If the inequality involves a “≥” or “≤”, the critical points where the rational expression equals zero are included in the solution.
- If the inequality involves a “>” or “<”, these points are not included, and we need to verify the behavior near these points for any change in sign.
5. Combine Intervals for the Solution

Finally, combine the intervals where the inequality is true to form your solution set:
- Write down the intervals where the inequality holds true.
- Use interval notation to clearly represent the solution on a number line.
This methodical approach ensures you cover all possible solutions, while also being accurate and logical.
💡 Note: Always plot your solution on a number line to visualize and ensure you’ve covered all cases.
Understanding and implementing these five methods can significantly ease the process of solving rational inequalities. These strategies not only help in solving these specific problems but also reinforce your overall mathematical proficiency, allowing you to tackle more complex problems with confidence.
As you become more proficient in these techniques, you'll find that your ability to analyze and solve mathematical inequalities will improve dramatically. Remember, practice is key; the more rational inequalities you solve, the better you'll get at recognizing patterns and anticipating solutions. This skill set will serve you well, not just in mathematics but in any field requiring logical problem-solving and analytical thinking.
What is the difference between a rational equation and a rational inequality?

+
A rational equation sets two expressions equal, while a rational inequality involves a comparison using inequality signs (<, ≤, >, ≥) between two expressions.
Why do we need to find the critical points?
+
Critical points help in determining where the sign of the expression changes, allowing us to divide the number line into intervals to test for the inequality.
Can a rational inequality have no solution?

+
Yes, if after testing the intervals, the inequality is never satisfied within any interval or at any critical point, then there is no solution.