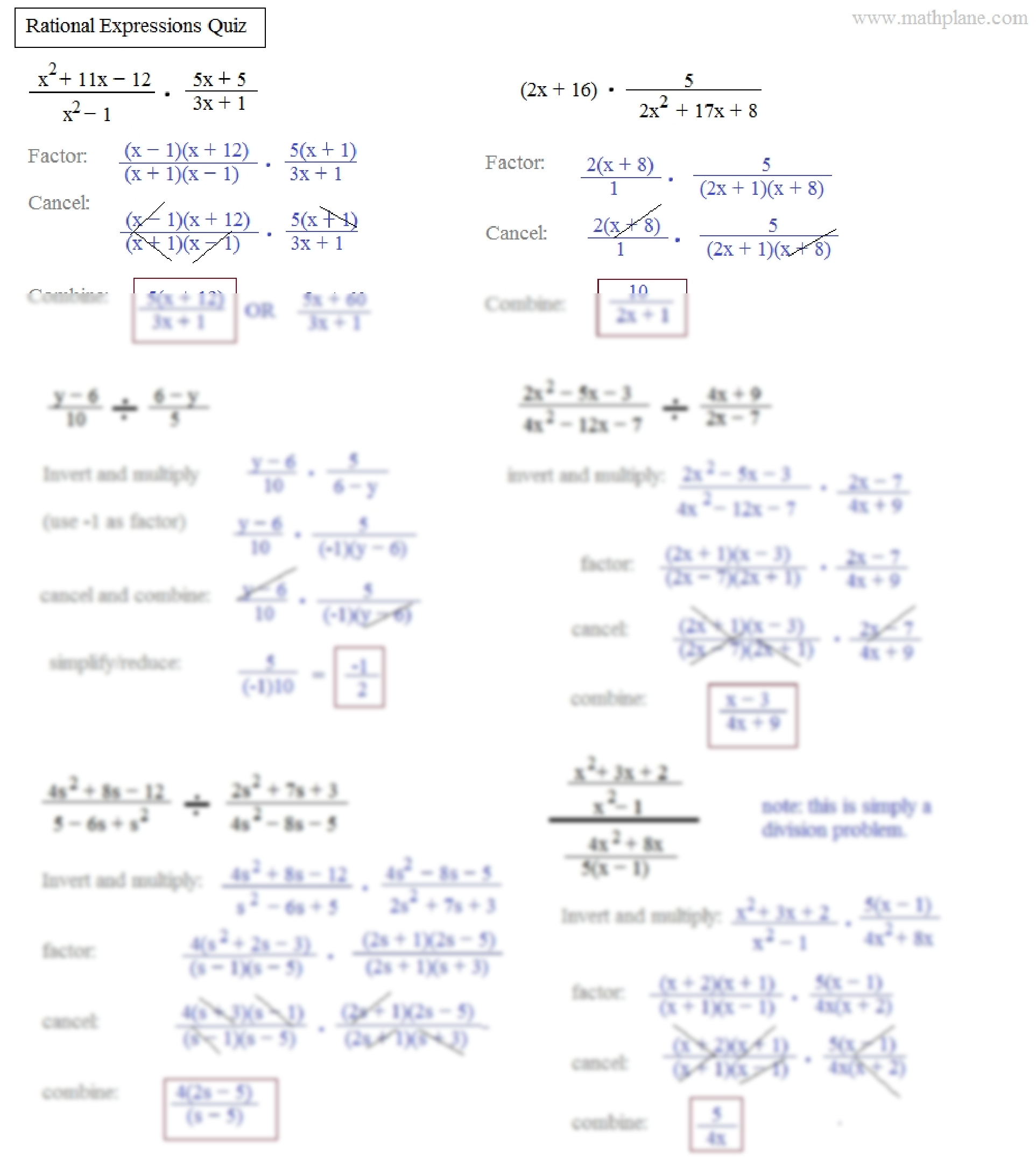
Rational Expressions Worksheet: All Answers Revealed

Engaging with mathematics often involves diving into complex topics like rational expressions. These mathematical constructs can sometimes be confusing, but with the right guidance and practice, mastering them becomes entirely possible. This guide will walk you through what rational expressions are, how to solve them, and importantly, how to ensure you are not making common mistakes in your algebra homework.
Understanding Rational Expressions

Rational expressions are fractions where both the numerator and the denominator are polynomials. They look similar to regular fractions but require special attention when performing operations like addition, subtraction, multiplication, or division.

Key Features of Rational Expressions

- Numerator and Denominator: Both are polynomials.
- Domain Restriction: The denominator cannot be zero, which influences the expression’s domain.
- Operations: Addition and subtraction require finding a common denominator, while multiplication and division involve simplifying first.
Simplifying Rational Expressions

The initial step in tackling rational expressions is simplifying them:
Step 1: Factorize

Factor both the numerator and the denominator into their simplest forms.
| Expression | Factored Numerator | Factored Denominator |
|---|---|---|
| (\frac{x^2 - 4}{x - 2}) | (\frac{(x - 2)(x + 2)}{1}) | (\frac{1}{x - 2}) |

After factoring, you can identify and cancel out common factors.
Step 2: Cancel Common Factors

Cancel any common factors in the numerator and the denominator to simplify the expression.
⚠️ Note: Remember, canceling factors does not mean you can cancel terms in a sum!
Addition and Subtraction

To add or subtract rational expressions, follow these steps:
Step 1: Find the Least Common Denominator (LCD)
The LCD is the smallest polynomial that is divisible by both denominators. Use factorization to find this.
For example:
- (\frac{x}{x+2} + \frac{3}{x-1})
The LCD here would be ((x+2)(x-1)).
Step 2: Rewrite Expressions

Rewrite each expression using the LCD:
- (\frac{x(x-1)}{(x+2)(x-1)} + \frac{3(x+2)}{(x+2)(x-1)})
Step 3: Combine

Combine the numerators over the common denominator and simplify:
- (\frac{x(x-1) + 3(x+2)}{(x+2)(x-1)})
Multiplication and Division

Multiplication and division of rational expressions are somewhat straightforward:
Step 1: Factor and Simplify

Factor each numerator and denominator, then simplify by canceling common factors:
- (\frac{x+2}{x-3} \times \frac{x-1}{x+2} = \frac{(x+2)(x-1)}{(x-3)(x+2)})
Which simplifies to (\frac{x-1}{x-3}).
Step 2: Division to Multiplication

To divide rational expressions, multiply by the reciprocal of the divisor:
- (\frac{1}{x-2} \div \frac{x-1}{x-3} = \frac{1}{x-2} \times \frac{x-3}{x-1})
With these steps, you can tackle any rational expression problem with confidence. Here are some important tips:
- Always check for conditions where your expression is undefined.
- Ensure you don’t cancel out terms in a sum; you can only cancel factors!
- When performing operations, keep an eye on the signs (positive or negative).
In wrapping up, rational expressions might seem daunting at first, but by understanding their structure, mastering the techniques to simplify, add, subtract, multiply, and divide them, you can handle any problem that comes your way. From basic simplification to solving complex equations, rational expressions are an essential part of algebra that, once mastered, will enhance your mathematical prowess significantly.
Why can’t the denominator of a rational expression be zero?

+
A denominator of zero in a fraction or rational expression makes it undefined in mathematics. Division by zero is not allowed, as it leads to situations where the laws of algebra fail.
How do I know if a rational expression can be simplified?

+
Look for common factors in the numerator and the denominator. If there are any, you can cancel them out, but remember to only cancel factors, not terms in a sum.
What is the significance of finding the Least Common Denominator (LCD) when adding rational expressions?
+Finding the LCD is crucial because it allows you to combine the expressions into one fraction with a common denominator, making the process of adding or subtracting rational expressions possible.



