Master Rational Expressions: Your Ultimate Worksheet Guide
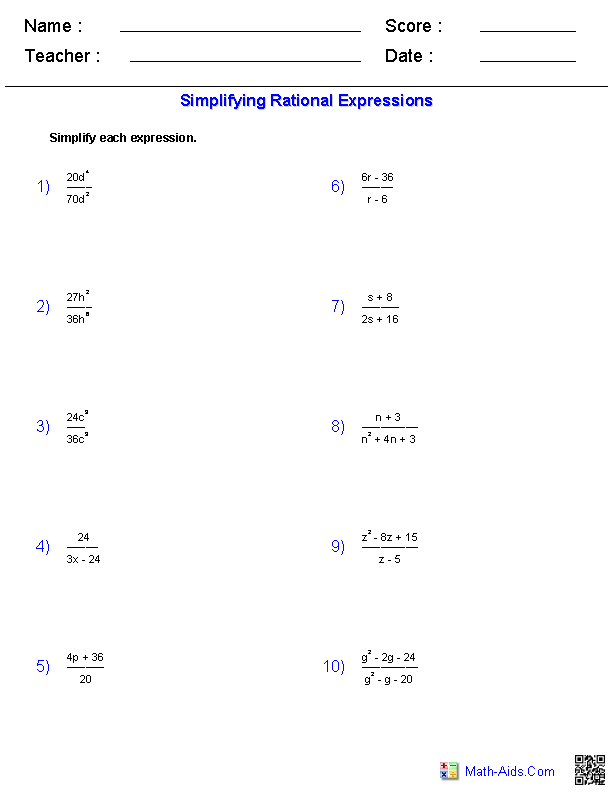
Welcome to your comprehensive guide on mastering rational expressions! If you're a student struggling with algebra or an educator seeking effective teaching methods, this guide will provide you with invaluable insights into understanding, simplifying, and solving rational expressions.
Understanding Rational Expressions

Rational expressions are fractions where both the numerator and the denominator are polynomials. Here’s a quick overview of what they involve:
- Numerator: The polynomial at the top of the fraction.
- Denominator: The polynomial at the bottom of the fraction.
📘 Note: A polynomial is an expression consisting of variables and coefficients combined using addition, subtraction, and multiplication, and non-negative integer exponents of variables.

Simplifying Rational Expressions

Simplifying rational expressions is about making them as easy to work with as possible. Here’s how you can do it:
- Factor out Common Factors: Identify any common factors in both the numerator and the denominator and simplify them.
- Cancel out Like Terms: After factoring, you can cancel out any like terms in the numerator and denominator, but remember, you can only cancel factors, not terms.
- Watch for Domain Restrictions: Simplify with caution as values that make the denominator zero are not part of the domain.
Solving Rational Equations

Solving equations with rational expressions involves these steps:
- Find a Common Denominator: All fractions should have the same denominator to combine terms.
- Multiply Through: Multiply every term by this common denominator to eliminate the denominators.
- Solve the Resulting Polynomial: Solve the simplified polynomial equation.
🧮 Note: Ensure to check your solutions for extraneous solutions, which might arise if your original denominator was zero for any solutions.
Applying Rational Expressions

Rational expressions aren’t just theoretical; they have practical applications in:
- Engineering: For designing circuits and systems.
- Physics: To describe relationships in laws like Ohm’s Law.
- Economics: In calculating cost, revenue, and profit functions.
| Field | Application |
|---|---|
| Engineering | Designing efficient signal processing systems |
| Physics | Calculating force using Hooke’s Law |
| Economics | Formulating production functions |

Worksheet Tips for Practice

Here are some tips to maximize your learning experience with rational expressions:
- Start with simpler expressions and gradually increase complexity.
- Use visual aids or diagrams to understand how expressions relate.
- Focus on understanding concepts rather than just doing problems.
Throughout your journey with rational expressions, remember that practice is key. The steps provided above should help you simplify, solve, and understand these expressions in various contexts. Whether you're solving for variable values, simplifying complex fractions, or exploring real-world applications, these strategies will guide you effectively.
Why do I need to check for extraneous solutions?

+
Extraneous solutions can arise in equations involving rational expressions when the process of solving might accidentally include numbers that do not work in the original equation, typically because they make the denominator zero.
Can rational expressions have negative exponents?

+
Yes, rational expressions can include terms with negative exponents, which can be rewritten in fractional form. However, these exponents are generally positive after simplification, as negative exponents indicate division.
How can I practice rational expressions effectively?

+
Practice by starting with basic simplification, moving to solving equations, and applying rational expressions in word problems. Use resources like textbooks, online worksheets, and problem sets for comprehensive practice.