Rational Expressions Worksheet Answers: Simplify and Solve
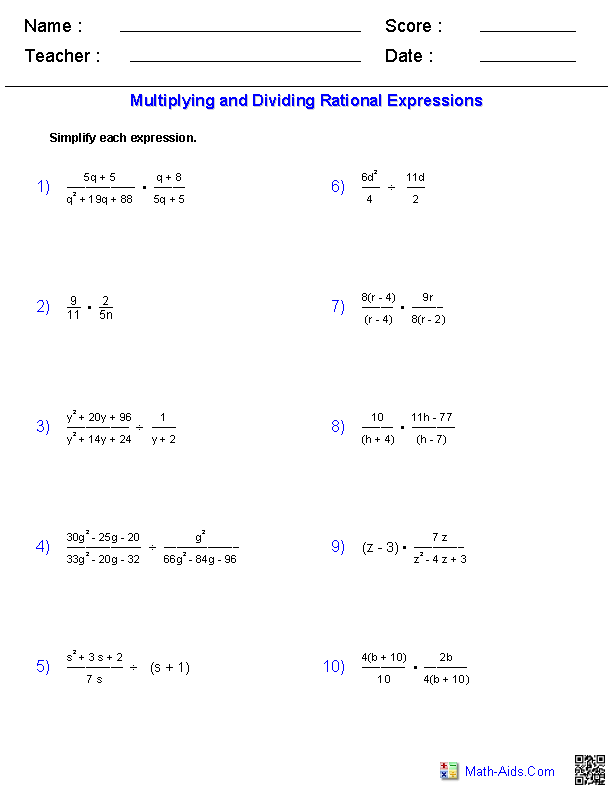
Understanding rational expressions is crucial for students navigating through algebra, as these expressions form the backbone of many higher-level math concepts. This blog post will delve deep into simplifying and solving rational expressions, providing a comprehensive guide for students and educators alike. We'll explore step-by-step methods, offer tips on common mistakes to avoid, and provide examples to solidify understanding.
What are Rational Expressions?

A rational expression is essentially a fraction where both the numerator and the denominator are polynomials. Here are some key points about rational expressions:
- They can represent real-life scenarios such as rates, ratios, and proportions.
- Understanding how to manipulate these expressions is fundamental in calculus, engineering, and more advanced mathematical fields.

Let’s start with the basics of simplifying these expressions.
Simplifying Rational Expressions

Simplifying rational expressions involves reducing them to their simplest form by factoring and canceling common factors:
1. Factor the Numerator and Denominator
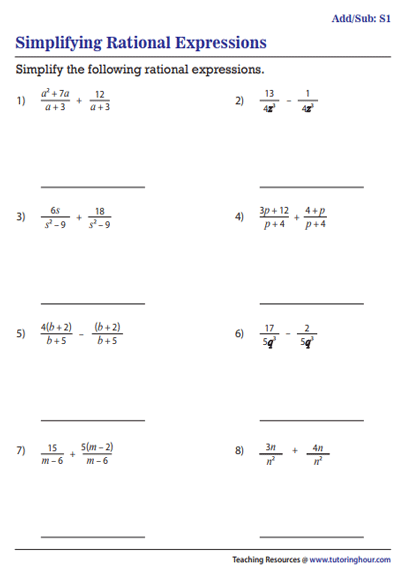
First, you factor both the top and the bottom polynomials completely:
- If the numerator is x2 + 5x + 6, it can be factored into (x + 2)(x + 3).
- For the denominator, say x2 - 1, you’ll get (x + 1)(x - 1).
2. Identify and Cancel Common Factors

After factoring, identify common factors in the numerator and the denominator. For example:
| Numerator | Denominator | Result |
|---|---|---|
| (x + 2)(x + 3) | (x + 1)(x - 1) | (x + 2)(x + 3) / (x + 1)(x - 1) = (x + 2) / (x - 1) |

3. Handle Undefined Values

Remember that certain values will make the expression undefined due to division by zero:
⚠️ Note: When x = 1, the expression is undefined.
Examples of Simplifying Rational Expressions

Let’s work through some examples to see simplification in action:
Example 1: Simplify (x2 - 9) / (x - 3)

Factoring gives us:
- Numerator: (x - 3)(x + 3)
- Denominator: (x - 3)
Cancelling out (x - 3), the result is (x + 3), but we must exclude x = 3 since it makes the denominator zero.
Example 2: Simplify (2x2 - x - 1) / (x - 1)

Factoring the numerator:
- Numerator: (2x + 1)(x - 1)
- Denominator: (x - 1)
After canceling, we get 2x + 1, again excluding x = 1.
Solving Rational Equations

Beyond simplifying, we often need to solve equations involving rational expressions:
Step-by-Step Method for Solving Rational Equations

- Identify the common denominator to eliminate the fractions.
- Multiply both sides by this denominator, simplifying the equation to a polynomial equation.
- Solve for x and check for extraneous solutions.
Example 3: Solve (x / (x + 3)) = 1 / (x - 1)
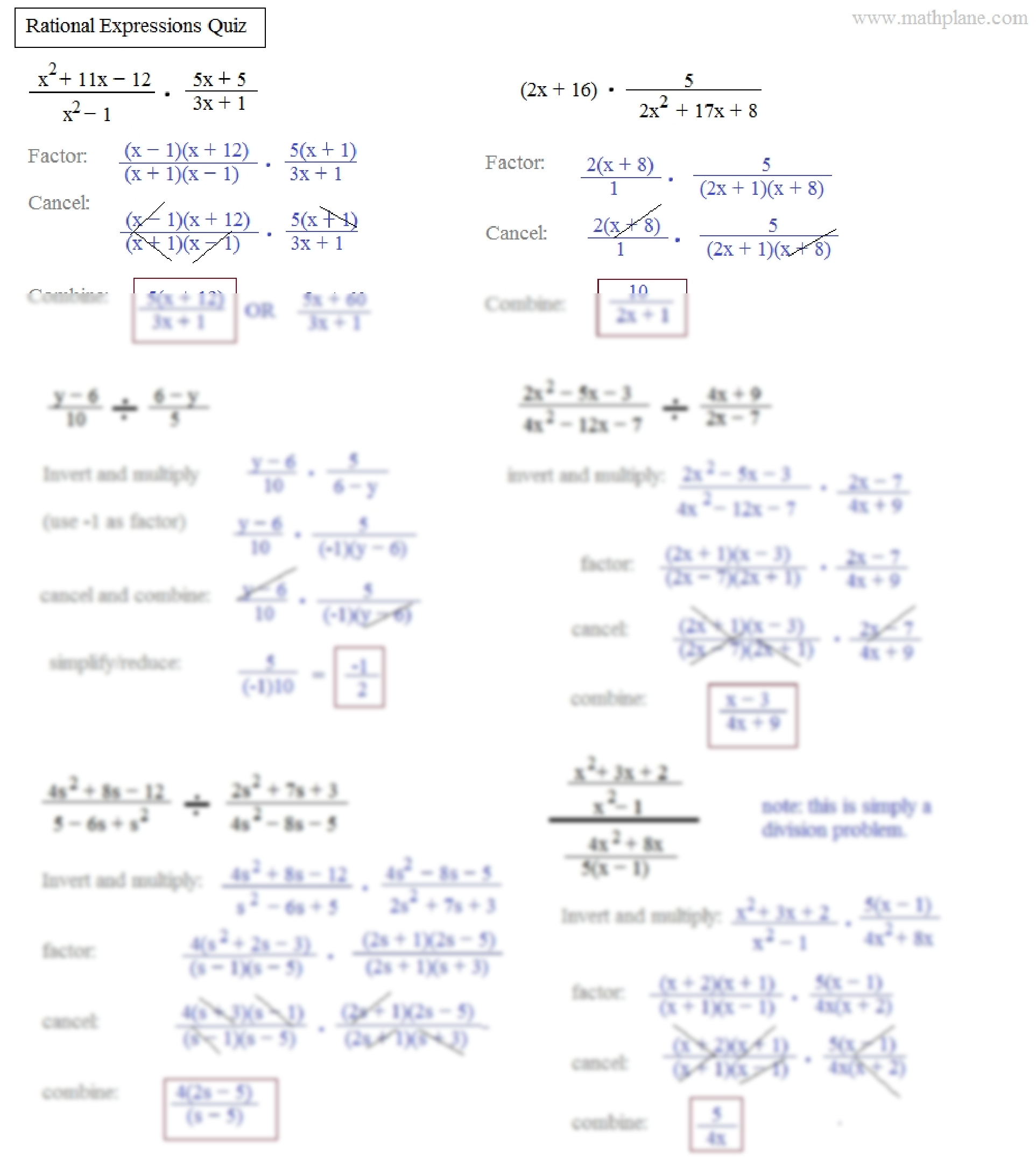
First, find a common denominator:
- x(x - 1) / ((x + 3)(x - 1)) = x(x - 1)
Multiply both sides by (x + 3)(x - 1):
- x = (x + 3) / (x - 1)
This leads to:
- x(x - 1) = x + 3
- x2 - x = x + 3
- x2 - 2x - 3 = 0
Factoring the quadratic:
- (x - 3)(x + 1) = 0
- x = 3 or x = -1
⚠️ Note: We must check for extraneous solutions, but in this case, both solutions are valid as they do not make the original denominators zero.
Pitfalls and Common Mistakes

Here are some common mistakes to avoid:
- Failing to consider undefined values where denominators become zero.
- Cancelling out factors when they are not actually common factors, leading to incorrect simplification.
- Ignoring the possibility of extraneous solutions in equations.
In wrapping up our journey through rational expressions, remember that the key to mastering them lies in practice and understanding the foundational principles. Simplifying rational expressions involves meticulous factoring, careful cancellation, and an awareness of where these expressions are undefined. Solving equations with rational expressions requires methodical steps to eliminate fractions, solve for the variable, and check for extraneous solutions. The ability to manipulate these expressions not only strengthens your algebra skills but also prepares you for advanced mathematical topics. Keep practicing, and the concepts will become clearer, enhancing your problem-solving capabilities in math and beyond.
What makes a rational expression undefined?

+
A rational expression becomes undefined when the denominator equals zero since division by zero is not allowed in mathematics.
How do I know if I’ve cancelled correctly in a rational expression?

+
Ensure you cancel only common factors, not terms. Common factors can be identified by factoring both the numerator and the denominator completely. Always verify by multiplying back the simplified form to see if you obtain the original expression.
What are extraneous solutions?

+
Extraneous solutions are apparent solutions that, when substituted back into the original equation, make it false or undefined. They often arise from operations like squaring or multiplying both sides by an expression that can be zero.