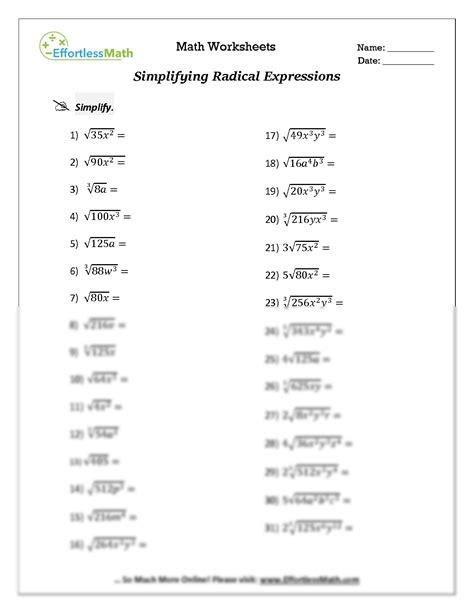
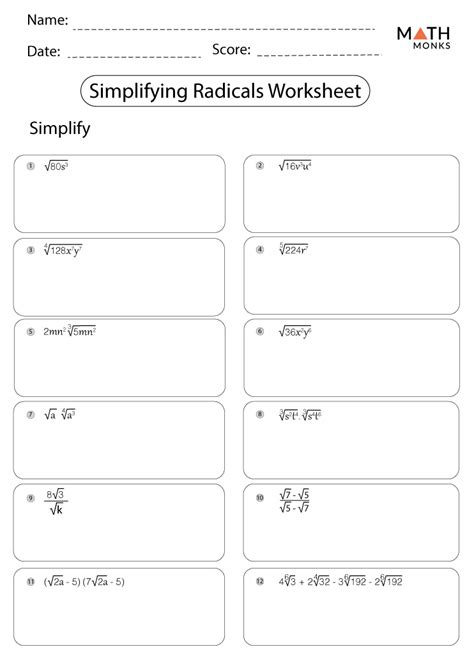
5 Ways to Simplify Radical Expressions

Understanding Radical Expressions

Radical expressions are a fundamental concept in algebra and mathematics, representing the root of a number. Simplifying radical expressions is an essential skill to master, as it helps in solving equations and performing mathematical operations. In this article, we will explore five ways to simplify radical expressions, providing a clear understanding of the process and its applications.
Method 1: Factoring Out Perfect Squares

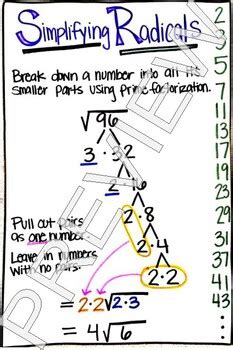
One of the simplest methods to simplify radical expressions is by factoring out perfect squares. A perfect square is a number that can be expressed as the square of an integer, such as 4, 9, or 16. When a perfect square is present under the radical sign, it can be factored out, simplifying the expression.
For example, consider the expression √16. This can be simplified by factoring out the perfect square 16:
√16 = √(4 × 4) = 4
Similarly, consider the expression √(x^2 + 4). This can be simplified by factoring out the perfect square x^2:
√(x^2 + 4) = √(x^2 + 2^2) = √(x + 2)^2 = x + 2
📝 Note: When factoring out perfect squares, make sure to check if the resulting expression is still in simplest form.
Method 2: Using the Product Rule

The product rule is a fundamental rule in algebra that states the product of two radical expressions can be simplified by multiplying the radicands (the numbers under the radical sign) and then taking the square root of the result.
For example, consider the expression √(4x) × √(9y). Using the product rule, we can simplify this expression as follows:
√(4x) × √(9y) = √(4x × 9y) = √(36xy) = 6√(xy)
📝 Note: When using the product rule, make sure to multiply the radicands correctly and simplify the resulting expression.
Method 3: Using the Quotient Rule

The quotient rule is another fundamental rule in algebra that states the quotient of two radical expressions can be simplified by dividing the radicands (the numbers under the radical sign) and then taking the square root of the result.
For example, consider the expression √(16x) ÷ √(4y). Using the quotient rule, we can simplify this expression as follows:
√(16x) ÷ √(4y) = √(16x ÷ 4y) = √(4x/y) = 2√(x/y)
📝 Note: When using the quotient rule, make sure to divide the radicands correctly and simplify the resulting expression.
Method 4: Simplifying Rational Exponents

Rational exponents are a type of exponent that can be expressed as a fraction. Simplifying rational exponents can help in simplifying radical expressions. To simplify rational exponents, we can use the following rules:
- (a^m)^n = a^(m×n)
- a^(m/n) = (a^m)^(1/n)
For example, consider the expression (2x)^3⁄2. Using the rules for rational exponents, we can simplify this expression as follows:
(2x)^3⁄2 = (2x)^(3×1/2) = 2^(3⁄2) × x^3⁄2 = 2√(2) × x√(x)
📝 Note: When simplifying rational exponents, make sure to apply the rules correctly and simplify the resulting expression.
Method 5: Using the Conjugate
The conjugate of a radical expression is an expression that has the same terms but opposite signs. Using the conjugate can help in simplifying radical expressions by eliminating the radical sign.
For example, consider the expression √(x + 2) + √(x - 2). Using the conjugate, we can simplify this expression as follows:
√(x + 2) + √(x - 2) = (√(x + 2) + √(x - 2)) × (√(x + 2) - √(x - 2)) / (√(x + 2) - √(x - 2))
= ((x + 2) - (x - 2)) / (√(x + 2) - √(x - 2))
= 4 / (√(x + 2) - √(x - 2))
📝 Note: When using the conjugate, make sure to apply the rules correctly and simplify the resulting expression.
In conclusion, simplifying radical expressions is an essential skill in algebra and mathematics. By using the methods outlined above, such as factoring out perfect squares, using the product rule and quotient rule, simplifying rational exponents, and using the conjugate, we can simplify radical expressions and solve equations. By mastering these skills, we can better understand and work with radical expressions in various mathematical contexts.
What is a radical expression?

+
A radical expression is a mathematical expression that represents the root of a number. It is denoted by the symbol √ and can be expressed as √(a), where a is the radicand (the number under the radical sign).
How do I simplify radical expressions?
+
There are several ways to simplify radical expressions, including factoring out perfect squares, using the product rule and quotient rule, simplifying rational exponents, and using the conjugate. The specific method used will depend on the form of the radical expression and the context in which it is being used.
What is the product rule for radical expressions?
+
The product rule for radical expressions states that the product of two radical expressions can be simplified by multiplying the radicands (the numbers under the radical sign) and then taking the square root of the result. For example, √(a) × √(b) = √(ab).
What is the quotient rule for radical expressions?

+
The quotient rule for radical expressions states that the quotient of two radical expressions can be simplified by dividing the radicands (the numbers under the radical sign) and then taking the square root of the result. For example, √(a) ÷ √(b) = √(a/b).
Related Terms:
- Radical expressions worksheet pdf
- Radical expressions worksheet with answers
- Simplifying radical expressions worksheet
- printable radicals worksheets
- radical expression worksheet pdf
- worksheet on simplifying radical expressions



