5 Unique Pythagorean Theorem Word Problems to Solve

Pythagorean Theorem, a fundamental principle in geometry, states that in a right-angled triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides. Expressed as an equation, it is a2 + b2 = c2, where c represents the hypotenuse while a and b are the other two sides. Understanding this theorem not only aids in mathematical problem-solving but also has numerous practical applications. Here, we will explore five unique word problems that require the application of this timeless theorem, providing not only a solution but also insights into various real-life scenarios where the Pythagorean Theorem can be applied.
Problem 1: The Farmer's Field

A farmer wants to add an electric fence to a part of his rectangular field. The field measures 45 feet by 30 feet, but he only needs to fence three sides because the existing house serves as the fourth side. Here's how the Pythagorean Theorem helps:
- Calculate the diagonal distance across the rectangle to ensure the fence posts are aligned correctly.
- The diagonal will serve as the length of the fence on the house side.
- Using the Pythagorean Theorem: 452 + 302 = c2, where c is the diagonal.
- Solving for c:
- 2025 + 900 = c2
- 2925 = c2
- c = √2925 ≈ 54.1 feet
🌾 Note: This problem demonstrates how the Pythagorean Theorem can be used in real-life agriculture scenarios to optimize fencing and resource use.
Problem 2: The Ladder Against the Wall
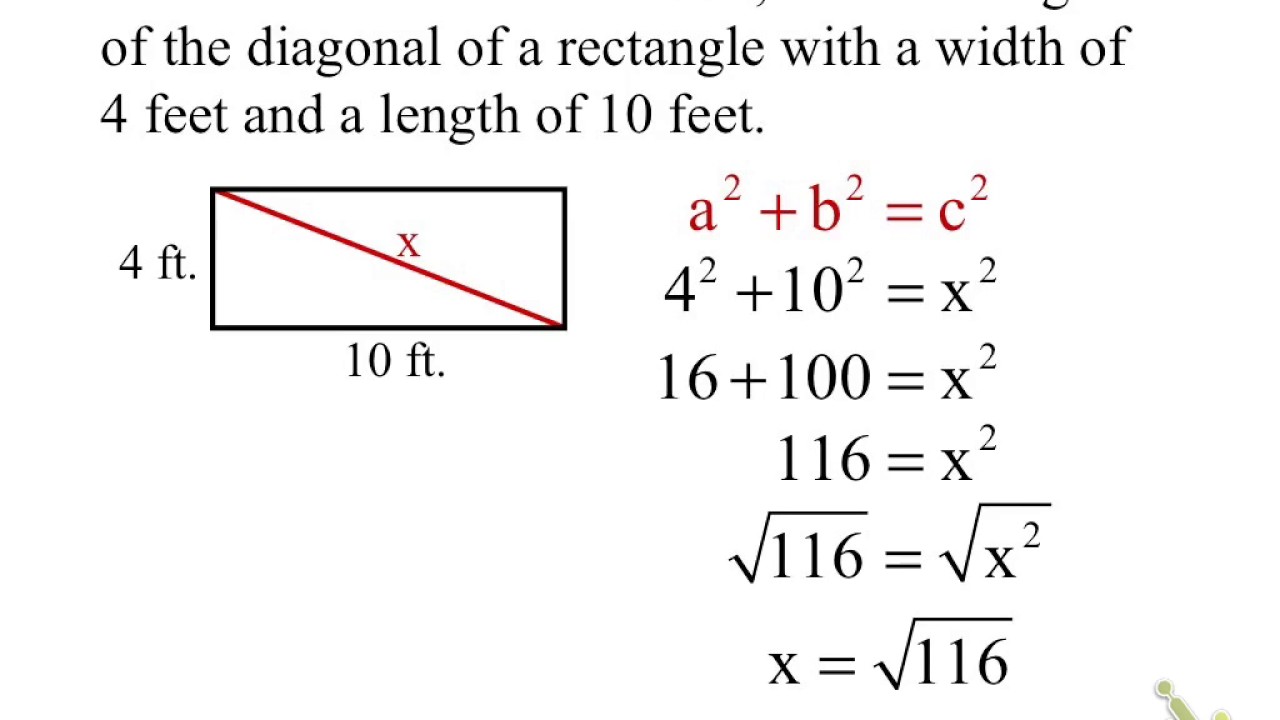
A homeowner wants to replace a lightbulb in an outdoor floodlight. She uses an 8-meter ladder, resting against the wall at a point 7.5 meters above the ground. She needs to determine the base of the ladder from the wall to ensure it is stable:
- Let the ladder, the wall, and the ground form a right-angled triangle.
- The ladder length (hypotenuse) is 8 meters.
- The height from the ground to the light is 7.5 meters.
- Using the Pythagorean Theorem:
- a2 + 7.52 = 82
- a2 + 56.25 = 64
- a2 = 7.75
- a = √7.75 ≈ 2.78 meters
🔍 Note: This application showcases the theorem's utility in construction and safety assessments.
Problem 3: Sizing Up A TV

An interior decorator needs to recommend a TV for a space where the dimensions of the display area are 72 inches wide and 40 inches tall. The Pythagorean Theorem helps in:
- Finding the diagonal measurement of the TV screen.
- Let width (a) be 72 inches and height (b) be 40 inches.
- Using the theorem:
- a2 + b2 = c2
- 722 + 402 = c2
- 5184 + 1600 = c2
- c2 = 6784
- c = √6784 ≈ 82.4 inches (measured diagonally)
📺 Note: The Pythagorean Theorem assists in making informed decisions in home decor and electronics, ensuring that products fit the intended space.
Problem 4: The GPS Distance Between Points

A hiker wants to know the shortest distance between two points on a hiking trail map:
- The coordinates of point A are (0,0) and point B are (3,4).
- Using the distance formula, which is derived from the Pythagorean Theorem:
- d = √((x2 - x1)2 + (y2 - y1)2)
- d = √((3 - 0)2 + (4 - 0)2)
- d = √(9 + 16)
- d = √25
- d = 5 kilometers
🌐 Note: This problem illustrates how the Pythagorean Theorem can extend into three-dimensional space for navigation purposes.
Problem 5: Creating A Custom Ramp
A shopkeeper needs to build a ramp for accessibility, knowing the height of the shop's threshold is 12 inches. She wants the ramp to have a 1:12 ratio for ease of use, as recommended by accessibility standards:
- The length of the ramp is given by the formula:
- Length = Height / Slope
- Length = 12 / (1/12)
- Length = 144 inches
- Now, to find the horizontal run:
- a2 + b2 = 1442
- a2 + 144 = 20736
- a2 = 20592
- a = √20592 ≈ 143.4 inches
♿️ Note: Accessibility is crucial, and the Pythagorean Theorem helps in designing inclusive infrastructure.
Summing up, these unique applications of the Pythagorean Theorem not only make for intriguing word problems but also reflect real-world scenarios where geometry plays a pivotal role. Whether it’s in agriculture, construction, home decor, navigation, or accessibility design, understanding how to apply this theorem can lead to efficient problem-solving and better decision-making. The elegance of the theorem lies not just in its mathematical beauty but in its versatility across various fields, highlighting the seamless connection between theory and practice.
Why is the Pythagorean Theorem important?

+
The Pythagorean Theorem is vital in understanding and solving problems involving right-angled triangles, which are common in construction, design, navigation, and engineering.
Can the Pythagorean Theorem be applied to non-right angled triangles?

+
Not directly. However, the Law of Cosines, which generalizes the Pythagorean Theorem, can be used for any triangle.
How does the Pythagorean Theorem relate to the Pythagorean Triples?

+
Pythagorean Triples are sets of three positive integers (a, b, c) that satisfy the theorem’s equation. They simplify calculations and provide pre-known right triangle side lengths.