Free Pythagorean Theorem Worksheet: Math Fun for All

Introduction to the Pythagorean Theorem

The Pythagorean Theorem is a cornerstone of geometry, named after the ancient Greek mathematician Pythagoras. It provides a fundamental relationship between the three sides of a right triangle. Simply put, it states that in any right-angled triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of squares of the other two sides. This principle is often represented with the equation:
c2 = a2 + b2Where a and b are the lengths of the triangle's legs, and c is the length of the hypotenuse. Understanding and applying this theorem can not only help in solving geometric problems but also in various real-world applications where right triangles are involved.

Why Learn the Pythagorean Theorem?

The Pythagorean Theorem isn't just an abstract concept; it has numerous practical applications:
- Construction: Builders and architects use it to ensure buildings are squared accurately.
- Navigation: Pilots and sailors apply it to navigate using latitude and longitude.
- Art and Design: Artists and graphic designers use it to create perfect right angles in their work.
- Everyday Measurement: From simple furniture assembly to complex engineering projects, the theorem aids in accurate measurements.
Putting the Pythagorean Theorem to Work with Worksheets

Worksheets are an excellent tool for mastering the Pythagorean Theorem. They provide hands-on practice, which is crucial for internalizing mathematical concepts. Here's how to make the most of Pythagorean Theorem worksheets:
1. Understand the Basics

Before diving into worksheets, ensure you grasp:
- What the theorem means.
- How to identify the hypotenuse and legs of a right triangle.
- The concept of squaring numbers and finding square roots.
2. Start Simple

Begin with worksheets that have straightforward problems, where two sides are given, and you need to find the third:
| Side 'a' | Side 'b' | Side 'c' (Hypotenuse) |
|---|---|---|
| 3 | 4 | ? |
| ? | 5 | 13 |
| 8 | ? | 17 |

3. Move to Word Problems

As you become comfortable, progress to problems set in real-world contexts:
Olivia has a ladder that is 15 feet long. She places it against a wall so that the bottom is 9 feet away from the base of the wall. How high on the wall does the ladder touch?
Use the theorem to solve: c2 = 152 - 92 = 144, hence c = 12 feet.
📝 Note: Remember to label the sides correctly when dealing with word problems. Always identify 'a', 'b', and 'c' in your equation.
4. Mix it Up with Variations

Some worksheets might introduce challenges like:
- Finding the hypotenuse when only one leg is known.
- Determining if a triangle is a right triangle using the theorem.
- Working with isosceles right triangles where legs are equal.
Enhancing Learning with Visual Aids

Visual aids can significantly boost comprehension when learning the Pythagorean Theorem:

1. Use Geoboards

Geoboards allow students to create right triangles physically, helping them see the relationship between the sides:
Create a right triangle on the geoboard with sides 3 and 4 units long. Use pegs to show where the hypotenuse would be, and measure it. Does it equal 5 units?
2. Interactive Software

Apps and online platforms provide interactive ways to manipulate triangles:
- GeoGebra: Allows real-time adjustments of sides to see how the theorem works.
- Desmos: Offers a graphing calculator where you can plot triangles and calculate using the theorem.
3. Physical Models
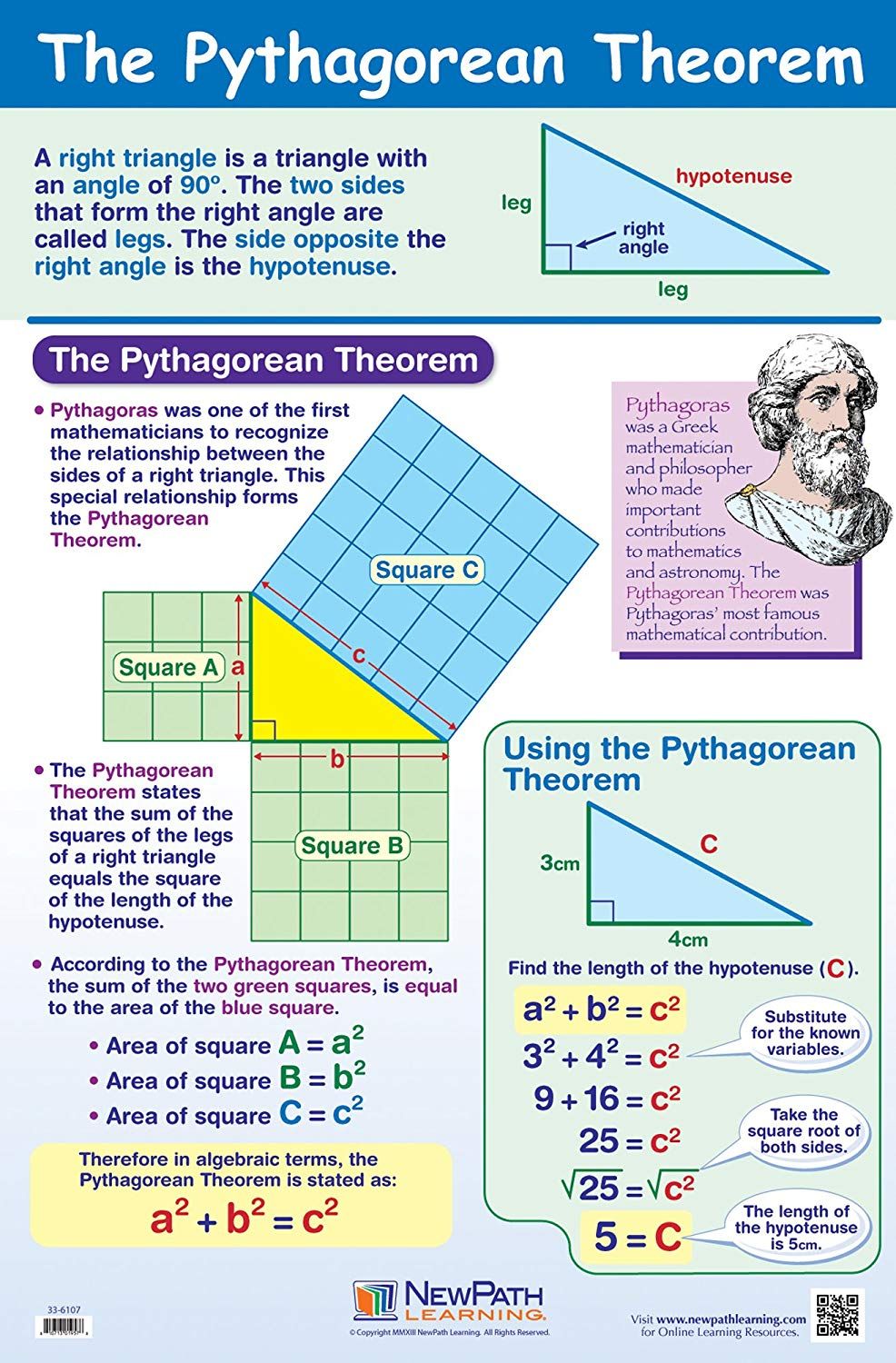
Physical models or cut-out shapes can help visualize the squares:
Cut out squares with areas corresponding to the legs of a right triangle. Overlay these on top of a square representing the hypotenuse. They should fit perfectly, demonstrating the theorem visually.
These aids not only make learning more engaging but also cater to different learning styles.
Application and Extension

Once familiar with the theorem, explore its extensions and applications:
1. Pythagorean Triples

Learn about sets of integers like {3, 4, 5} or {5, 12, 13} that form right triangles:
| Side 'a' | Side 'b' | Hypotenuse 'c' |
|---|---|---|
| 3 | 4 | 5 |
| 5 | 12 | 13 |
| 8 | 15 | 17 |
2. Pythagorean Theorem in 3D

Extend the theorem to 3-dimensional shapes like rectangular prisms:
A rectangular box has a diagonal of 15 cm, with dimensions of 9 cm by 12 cm by x cm. Find x using the 3D extension of the Pythagorean Theorem.
To solve, use:
x = √(152 - 92 - 122)This exploration into 3D space showcases the theorem's versatility.
Recap of Key Learnings

We've traversed the world of the Pythagorean Theorem, from its basic tenets to its applications:
- Understanding the theorem's foundational equation and its proof.
- Applying the theorem to solve for unknown lengths in right triangles.
- Using it in practical scenarios through word problems and visual aids.
- Exploring extensions like Pythagorean triples and 3D applications.
By engaging with worksheets, visual aids, and real-world problems, you've not only learned a mathematical principle but also gained skills in logical reasoning and problem-solving.
What is the Pythagorean Theorem?

+
The Pythagorean Theorem states that in a right-angled triangle, the square of the length of the hypotenuse © is equal to the sum of squares of the other two sides (a and b). It’s represented by the equation: c2 = a2 + b2.
How do I know if a triangle is a right triangle?
+You can use the Pythagorean Theorem to check if a triangle is a right triangle. If c2 = a2 + b2, where ‘c’ is the longest side, then it is a right triangle. If c2 < a2 + b2, it’s an acute triangle, and if c2 > a2 + b2, it’s an obtuse triangle.
Can the Pythagorean Theorem be used with non-right triangles?
+No, the Pythagorean Theorem applies specifically to right triangles. For other triangles, different formulas, like the Law of Cosines, are used to relate side lengths and angles.