5 Quick Answers to Pythagorean Theorem Worksheet Problems

Understanding the Pythagorean Theorem: The Pythagorean theorem is a fundamental principle in geometry that states in a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the other two sides. This can be expressed as a2 + b2 = c2. Here's how you can quickly solve problems related to this theorem:
The Basics of the Pythagorean Theorem


Before diving into complex problems, it’s crucial to understand:
- What a right triangle is: A triangle where one of the angles measures 90 degrees.
- Identifying the hypotenuse: The longest side, directly opposite the right angle.
- Using the formula: Plug in the known values for a and b or c into the theorem equation to find the missing side.
Problem 1: Finding the Hypotenuse
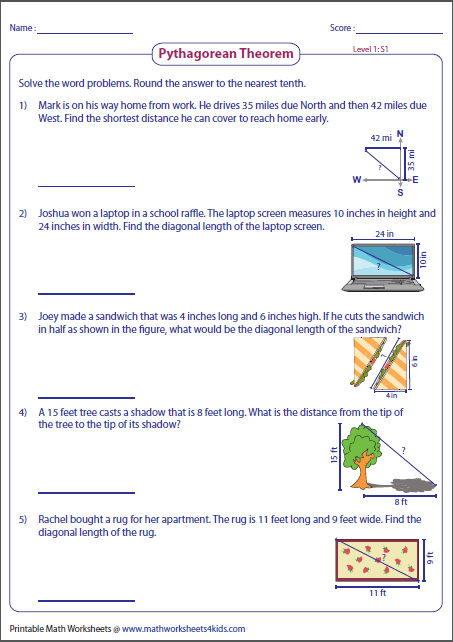

Let’s consider a triangle where a = 3 and b = 4:
- Square a to get 32 = 9.
- Square b to get 42 = 16.
- Add the results: 9 + 16 = 25.
- Take the square root of 25 to find c: √25 = 5.
So, the hypotenuse is 5 units.
Problem 2: Solving for One of the Legs


If we know c = 13 and a = 5, we need to find b:
- Square c to get 132 = 169.
- Square a to get 52 = 25.
- Subtract a2 from c2: 169 - 25 = 144.
- Take the square root of 144 to find b: √144 = 12.
The other leg, b, measures 12 units.
Problem 3: Determining if a Triangle is Right


Given sides 6, 8, and 10, we can check if this forms a right triangle:
- Identify the potential hypotenuse (the largest side): 10.
- Calculate 62 + 82 = 36 + 64 = 100.
- Since 100 = 102, this triangle does indeed satisfy the Pythagorean theorem, confirming it is a right triangle.
Problem 4: Real-World Application

Suppose you need to find the length of a ladder to reach the top of a wall 10 feet high from 8 feet away:
- The wall and the ground form a right triangle.
- Let a = 8 (distance from the wall), b = 10 (height of the wall), and c the ladder’s length.
- Use the formula: 82 + 102 = c2, which gives 64 + 100 = c2, leading to 164 = c2.
- Thus, c = √164 ≈ 12.8 feet.
Problem 5: Triples and Patterns

The Pythagorean triples, such as 3-4-5 or 5-12-13, make solving problems easier. These sets:
- Follow the pattern where a = m2 - n2, b = 2mn, and c = m2 + n2.
- Here, m and n are positive integers where m > n.
- To find a triple, choose m and n such that they’re coprime (i.e., they have no common divisor other than 1).
Notes on Solving Pythagorean Theorem Problems

When approaching these problems:
🖌️ Note: Always identify which side is the hypotenuse first, as this affects how you use the formula.
🕵️ Note: Triples can be scaled up or down by multiplying all sides by the same factor.
Summing Up: The Pythagorean theorem is a versatile tool in mathematics, with its applications spanning from simple geometry problems to real-world scenarios like architecture, engineering, and surveying. By mastering the theorem, you can not only solve for unknown sides in right triangles but also identify right triangles from given side lengths. Understanding these principles opens up a world of practical problem-solving.
What is the Pythagorean Theorem?

+
The Pythagorean Theorem states that in a right triangle, the square of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the other two sides, expressed as a2 + b2 = c2.
How do you know if a triangle is right angled using the Pythagorean theorem?

+
If the square of the longest side equals the sum of the squares of the other two sides, the triangle is right angled. For example, if a = 3, b = 4, and c = 5, then 32 + 42 = 9 + 16 = 25, which equals 52.
Can the Pythagorean Theorem be used in non-right triangles?

+
No, the theorem applies only to right triangles. For other triangles, you’d need different methods like the Law of Cosines.
What are Pythagorean triples?

+
Pythagorean triples are sets of three positive integers a, b, and c such that a2 + b2 = c2. Common examples include (3, 4, 5) and (5, 12, 13).
How can I find the length of the ladder needed to reach a point on a wall?

+
Use the Pythagorean Theorem if you know the height of the wall and the distance from the wall to where the ladder will rest. For example, with a wall height of 10 feet and distance of 8 feet, calculate as shown in Problem 4.



