Pythagorean Theorem Worksheet: Engaging Math Practice

Discovering the beauty of geometry can be a fun and insightful experience, especially with the right tools in hand. One of the fundamental principles in geometry, the Pythagorean Theorem, provides an excellent opportunity for students to engage with mathematics in a practical manner. This post will introduce an engaging Pythagorean Theorem worksheet designed to help students apply and understand this theorem through various exercises, ensuring a solid grasp on this essential mathematical concept.
Understanding the Pythagorean Theorem

The Pythagorean Theorem is a cornerstone of geometry. It states that in a right-angled triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the other two sides. This can be mathematically expressed as:
a2 + b2 = c2
Where a and b are the lengths of the legs of the triangle, and c is the length of the hypotenuse.
The Importance of Practice

Practice is key in mastering any concept in mathematics, and the Pythagorean Theorem is no exception. By engaging with problems, students can:
- Develop a deeper understanding of how the theorem applies in real-world scenarios.
- Improve their calculation skills, particularly with square roots and squares.
- Gain confidence in manipulating equations.
The Pythagorean Theorem Worksheet
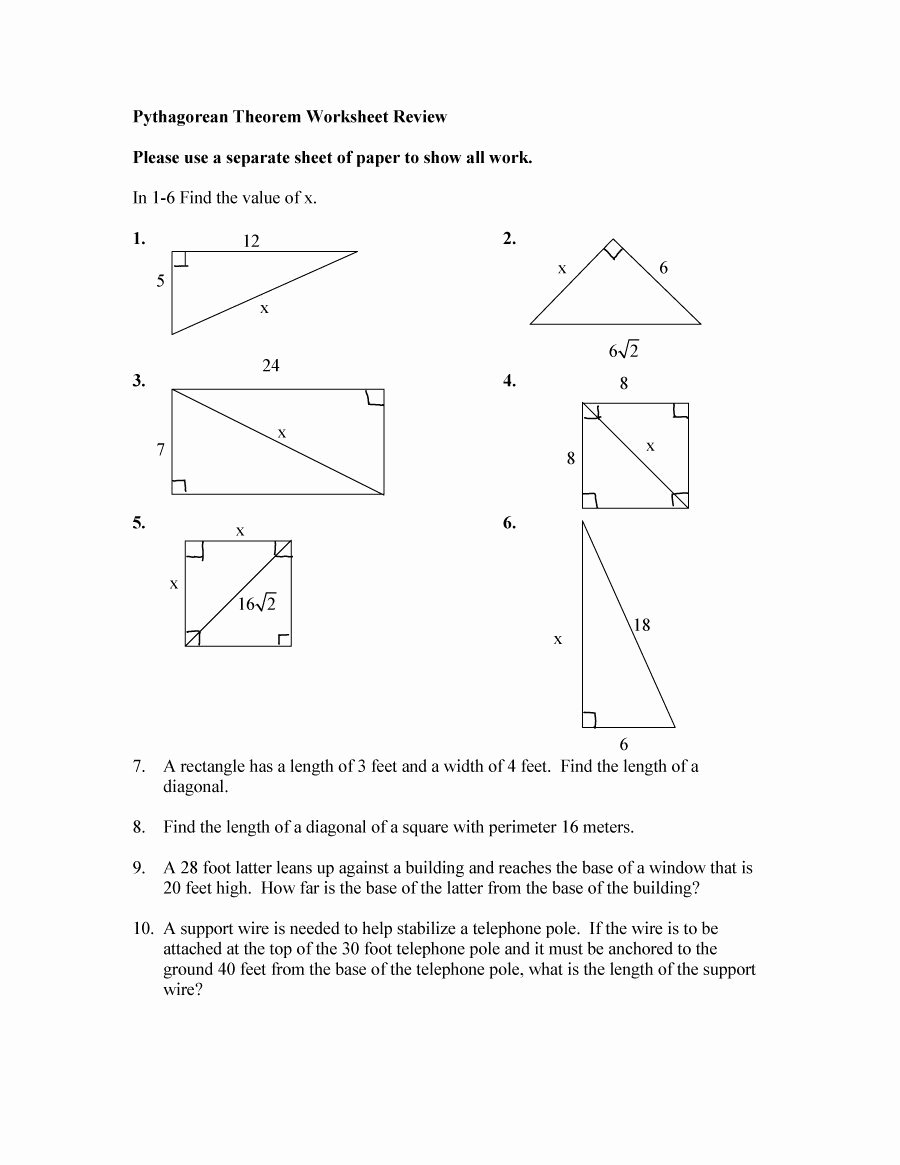
Our Pythagorean Theorem Worksheet is designed to cover various aspects of the theorem, ensuring a comprehensive learning experience:
- Basic Problems: Simple, straightforward problems to familiarize students with the theorem.
- Word Problems: Real-life scenarios where students must apply the theorem to solve practical problems.
- Proof and Variations: Tasks that encourage students to understand why the theorem works and explore its variations.
Basic Problems


This section provides ten problems where students calculate the missing length in a right-angled triangle. Here, students will:
- Learn to identify which side is the hypotenuse.
- Practice setting up and solving the Pythagorean equation.
Word Problems

In real-world scenarios, the Pythagorean Theorem helps solve problems involving right triangles. Here are some examples:
- A ladder leaning against a wall to find the height it can reach.
- Finding the diagonal distance across a rectangular field.
Students will:
- Interpret and extract relevant information from word problems.
- Apply the theorem to practical situations.
Proof and Variations

Understanding why the Pythagorean Theorem works can be as enriching as knowing how to use it. This section:
- Introduces different proofs of the theorem.
- Explores related geometric relationships and theorems.
📝 Note: Encourage students to visualize the theorem using physical objects like squared paper or grids to see how it physically holds true.
How to Use This Worksheet Effectively

To make the most out of this worksheet:
- Start with a brief review of the theorem and its formula.
- Proceed from basic to more complex problems gradually.
- Encourage group work or peer review to foster discussion and learning.
- Use the proof section as a classroom activity or homework assignment for deeper exploration.
Incorporating this worksheet into your teaching strategy not only reinforces the understanding of the Pythagorean Theorem but also makes learning geometry enjoyable and relatable. By engaging with different problem types, students solidify their knowledge and learn how mathematics applies to the world around them.
The exploration of the Pythagorean Theorem through various methods of application and proof offers students a chance to see mathematics as both a precise science and a tool for discovery. By providing a mix of calculation practice, real-world application, and theoretical understanding, this worksheet serves as a comprehensive tool for deepening students' comprehension of one of geometry's fundamental principles. Let this worksheet be a stepping stone for students to explore the vast world of geometric shapes and their intrinsic beauty.
What is the Pythagorean Theorem?

+
The Pythagorean Theorem states that in a right-angled triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the other two sides, expressed as a2 + b2 = c2.
Why is the Pythagorean Theorem important?

+
It’s fundamental in geometry and has numerous real-world applications, from navigation to architecture, engineering, and even in art.
How can I make learning the Pythagorean Theorem fun for students?

+
Using activities like constructing models, solving puzzles or crosswords, and applying the theorem in games or real-life scenarios can make learning enjoyable.