7 Fun Pythagorean Theorem Worksheets for Students

In the realm of mathematics, few concepts are as celebrated and universally recognized as the Pythagorean Theorem. This theorem, which relates the lengths of the sides of a right triangle, is not just a cornerstone of geometry but also an engaging topic for educational exploration. Here, we delve into seven creative and enjoyable worksheets designed to make learning the Pythagorean Theorem an interactive and memorable experience for students.
Worksheet 1: Introducing the Theorem


The first worksheet is dedicated to laying the foundation. Here, students are introduced to the theorem through:
- Definitions of key terms like hypotenuse, legs, and right triangle.
- A visual representation of the theorem using a simple right triangle.
- Simplistic problems to apply the theorem directly, like calculating the length of the hypotenuse when the legs are given.
📚 Note: Ensuring students understand the basic concept before proceeding is crucial for mastering more complex applications later on.
Worksheet 2: Proof and Verification


Verification of the Pythagorean Theorem can be an exciting challenge:
- Students are provided with various geometric proofs, from graphical to algebraic.
- They're encouraged to verify the theorem themselves through cut-and-paste activities or logical reasoning.
Worksheet 3: Real-World Applications


Making the theorem relevant is key to engagement:
- Questions involve calculating distances in real-life scenarios, like determining the shortest path for a dog walker.
- Students explore how the theorem applies to architecture, navigation, and computer graphics.
🌍 Note: Demonstrating how mathematical concepts apply in everyday life can significantly increase student interest.
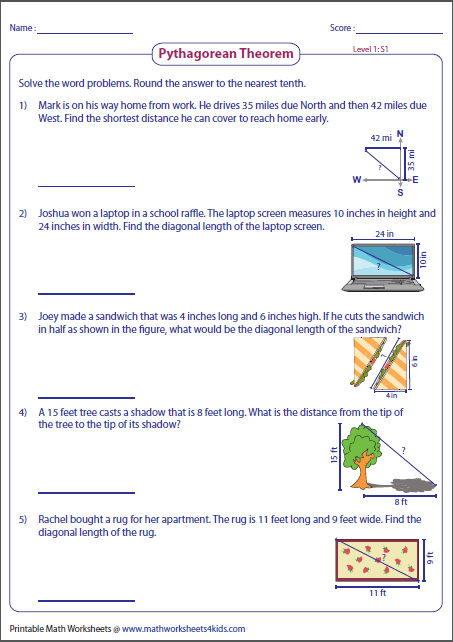
Worksheet 4: Word Problems


Engaging word problems foster problem-solving skills:
- Scenarios requiring students to set up the equation themselves.
- Complex situations where the theorem isn't initially obvious, promoting critical thinking.
Worksheet 5: Pythagorean Triples


Discovering Pythagorean triples:
- Worksheet designed for finding or verifying triples (3, 4, 5).
- Students explore common triples, their properties, and how to generate more.
Worksheet 6: Pythagorean Theorem in 3D

Extending into three dimensions:
- Problems involve calculating the length of a diagonal in a rectangular box or distance between points in space.
📐 Note: Transitioning from 2D to 3D applications can broaden students' mathematical understanding.
Worksheet 7: Creating Your Own Proofs


Encouraging creativity in proof:
- Students are prompted to devise and illustrate their own proofs.
- This worksheet assesses both creativity and comprehension of the theorem.
By journeying through these worksheets, students not only deepen their understanding of the Pythagorean Theorem but also appreciate its versatility in problem-solving. The strategic design of these activities promotes logical thinking, creative problem-solving, and real-world application, making the theorem an engaging and relevant topic. This comprehensive approach ensures that learning is both enjoyable and effective.
What are Pythagorean triples, and why are they important?

+
Pythagorean triples are sets of three whole numbers (a, b, c) where a² + b² = c². They’re important because they provide solutions to the Pythagorean Theorem without any calculation needed, making them useful in both education and practical applications.
Can the Pythagorean Theorem be used for non-right triangles?

+
Yes, though it’s most straightforward with right triangles. For non-right triangles, use trigonometric formulas or Law of Cosines, which also incorporates the Pythagorean Theorem.
How can I make teaching the Pythagorean Theorem engaging?

+
Incorporate visual aids, real-life scenarios, interactive worksheets, and challenge students to create their own proofs or find unique applications.