5 Ways to Master Pythagorean Theorem Worksheets

Exploring the Pythagorean Theorem

The Pythagorean Theorem is a fundamental principle in mathematics, offering insights into the relationships between the sides of a right-angled triangle. This theorem, dating back to ancient Greece, has not only stood the test of time but also finds applications in various fields like engineering, architecture, and even modern-day gaming. For students, mastering the Pythagorean Theorem is often a gateway to more advanced mathematical concepts. Here’s how you can become proficient with Pythagorean Theorem worksheets:
1. Understanding the Basics

Before diving into the exercises, understanding the core theorem is crucial:
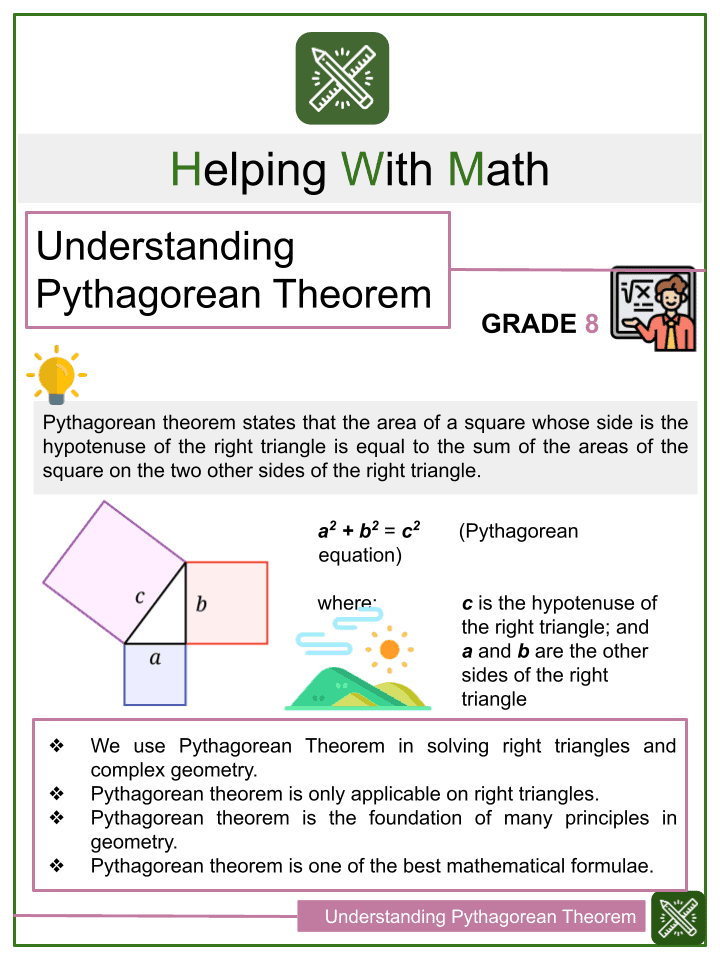
- Formula: a² + b² = c² where a and b are the legs of the triangle, and c is the hypotenuse.
- Proof: There are several proofs, with the most common being through dissection or algebraic methods.

📚 Note: Understanding the proofs is not mandatory for most worksheet applications but can provide deeper insight.
2. Selecting the Right Worksheets

Choosing appropriate worksheets can significantly impact your learning:
- Difficulty Levels: Ensure the worksheets match your skill level, progressing from basic to complex problems.
- Thematic Worksheets: Real-world applications or puzzles can make learning more engaging.
- Mix of Problem Types: Include word problems, multiple-choice, true/false, and algebraic proof questions.
3. Practice with Variety

The key to mastering any mathematical theorem lies in repetitive practice:
| Worksheet Type | Description |
|---|---|
| Basic Problems | Finding the hypotenuse when legs are given. |
| Inverse Problems | Finding a leg when the hypotenuse and one leg are known. |
| Word Problems | Applying the theorem in real-life scenarios. |
| Proof Verification | Checking if a triangle is right-angled using the theorem. |

4. Incorporating Technology and Interactive Tools

Modern tools can enhance your understanding:
- Interactive Apps: Use apps that simulate real-world problems or 3D visualization.
- Online Calculators: Practice verifying your manual calculations.
- Videos and Tutorials: Watch explanations of tricky problems or proofs for a different perspective.
5. Review and Reflection

After each session:
- Revise: Go over common mistakes and learn from them.
- Reflect: Understand where the theorem applies beyond worksheets.
- Set Goals: Plan what aspects you need to improve for the next session.
By following these steps, you'll not only solve Pythagorean Theorem problems with ease but also understand its broader implications and applications. Remember, mastery comes with practice, patience, and persistent curiosity.
What are the best types of worksheets for beginners?

+
For beginners, worksheets with basic problems focusing on calculating the hypotenuse using the Pythagorean formula are ideal.
Can I use the Pythagorean Theorem in real life?

+
Absolutely! You can use it in construction, surveying, navigation, and even in designing computer graphics or animations.
How often should I practice?
+
Daily practice, even for 15-20 minutes, can significantly enhance your proficiency with the theorem.
Do I need to memorize the proof of the Pythagorean Theorem?

+
While not mandatory, understanding a proof can deepen your appreciation and application of the theorem.
How do I know if I’ve mastered the Pythagorean Theorem?

+
If you can solve various problem types quickly, apply it in real-life scenarios, and explain it to others, you’re on your way to mastery!
Summing up, the journey to mastering the Pythagorean Theorem through worksheets involves understanding the basic principles, selecting tailored worksheets, practicing with a range of problems, using technology, and constant review. This approach not only prepares you for mathematical challenges but also enriches your understanding of this timeless theorem, enhancing your problem-solving skills across various contexts.