Mastering Segment Relationships Proofs Worksheet Guide

Mastering the art of geometric proofs is a fundamental skill for students in high school or advanced math classes. Among the various types of proofs, segment relationship proofs hold a special place because they provide insight into how different segments of geometric figures interact. This guide will walk you through the process of understanding and mastering segment relationships proofs, equipping you with the tools to tackle complex geometry problems effectively.
The Fundamentals of Segment Relationship Proofs
Geometric proofs often require a combination of understanding geometric principles and logical reasoning. Segment relationship proofs focus on how segments within or between figures relate to each other, which can include:
- Equal segment lengths
- Midpoints
- Perpendicular bisectors
- Parallels
- Angle bisectors
To start, here are some key concepts you need to grasp:
Understanding Congruent Segments
In geometry, if two line segments have the same length, they are congruent. This is often the cornerstone of segment relationship proofs where you’ll frequently need to prove segments are equal in length or show that they are not.
The Role of Midpoints

A midpoint is a point on a line segment that divides it into two equal parts. Recognizing and using midpoints in proofs helps establish relationships like:
- Proving that a line is a median in a triangle
- Determining properties of parallelograms
Perpendicular Bisectors

A line that bisects a segment at its midpoint and is also perpendicular to it plays a significant role in proofs involving triangles, circles, and polygons:
- Equidistant points
- Circumcenter
- Angle relationships
Using Parallel Lines

When lines are parallel, their relationships with segments can lead to proving:
- Transversal properties
- Proportional segments
- Angle congruences
Angle Bisectors
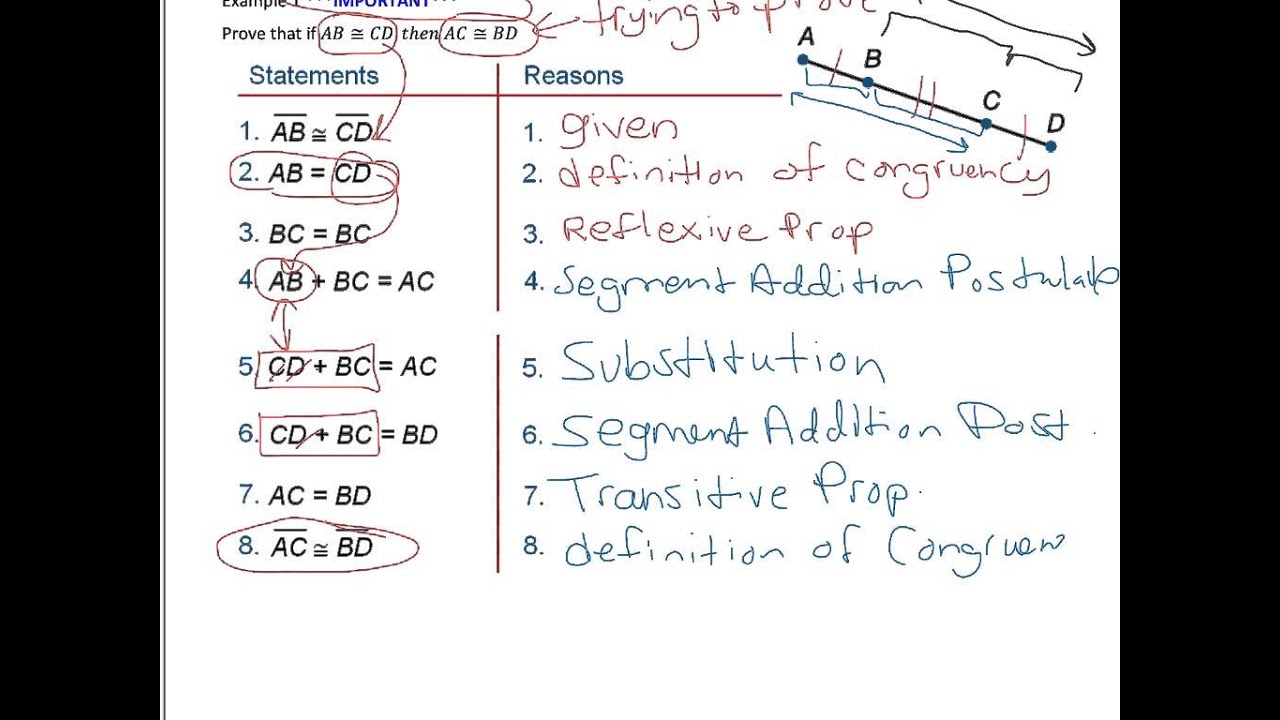
Angle bisectors can help in:
- Proving segment relationships via triangle congruences or similarity
- Using the Angle Bisector Theorem
Strategies for Segment Relationship Proofs

Here are some strategies to navigate through segment relationship proofs:
Step-by-Step Analysis

Begin by identifying what you know from the given information:
- List all given statements.
- Mark diagrams with the given information.
- Identify what you need to prove.
Then, work systematically from the known towards the unknown:
- Look for immediate consequences from the given statements.
- Use definitions, postulates, and theorems to build your proof.
Use of Geometric Theorems

Here’s a non-exhaustive list of relevant theorems:
| Theorem/Postulate | Description |
|---|---|
| Congruent Segment Postulate | Two segments are congruent if their lengths are equal. |
| Angle Bisector Theorem | The angle bisector of an angle in a triangle divides the opposite side into segments that are proportional to the adjacent sides. |
| Midsegment Theorem | The midsegment of a triangle (connecting the midpoints of two sides) is parallel to the third side and half as long. |
| Perpendicular Bisector Theorem | Any point on the perpendicular bisector of a segment is equidistant from the endpoints of the segment. |
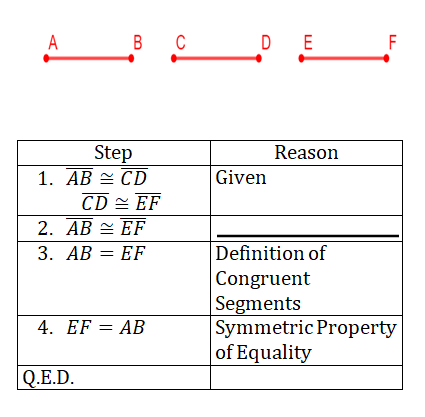
Proof Techniques
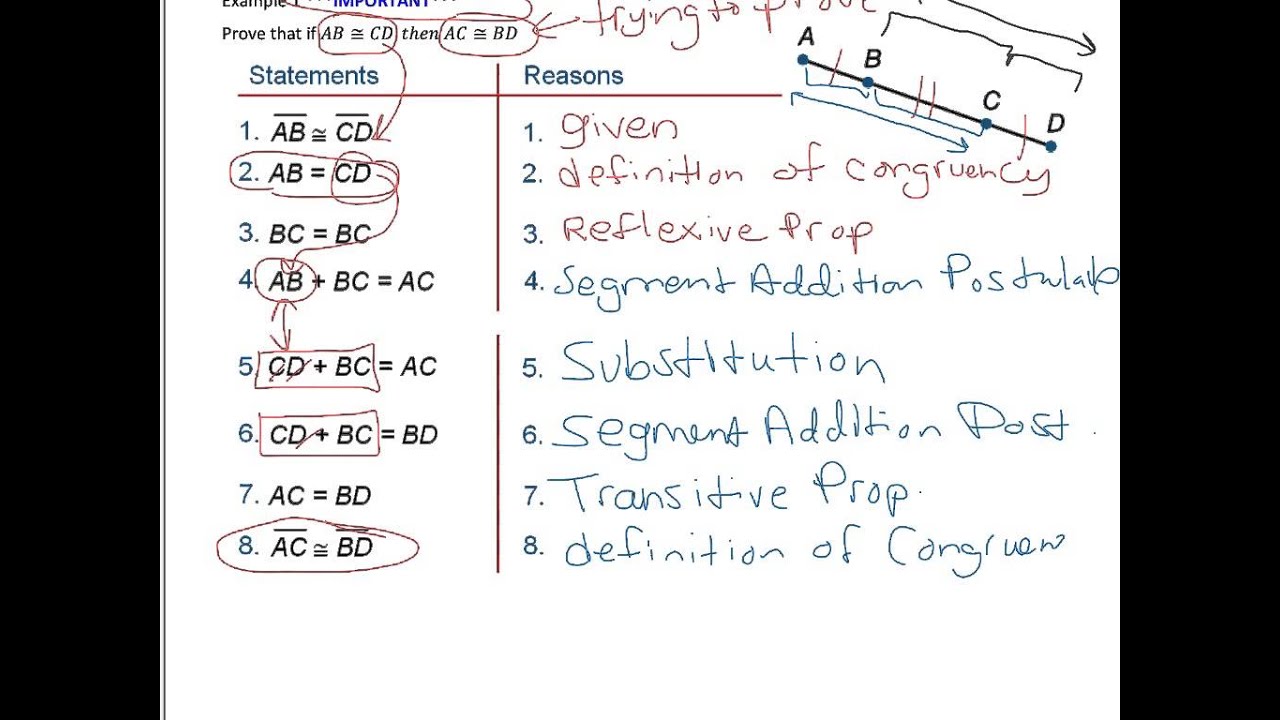
Here are some common proof techniques:
- Direct Proof: Starting with what is given and working directly towards what you want to prove.
- Indirect Proof (Proof by Contradiction): Assuming the opposite of what you want to prove and showing that leads to a contradiction.
- Two-Column Proofs: A structured format showing statements and reasons in parallel columns.
- Paragraph Proofs: Writing proofs in paragraph form, explaining each step in the narrative.
Tips for Completing Segment Relationships Proofs

Here are some practical tips to make your proof process smoother:
- Draw a Detailed Diagram: Make sure your diagram reflects all given information, including angles, segment lengths, or special markings.
- Use Color Coding: Highlight different segments or relationships in different colors to visually distinguish them.
- Label: Name points, segments, and angles clearly to avoid confusion.
- Remember Definitions: Keep basic geometric definitions at hand, especially those related to segments and angles.
- Utilize Theorems: Know your theorems and how they can be applied in different scenarios.
⚠️ Note: Avoid jumping to conclusions without a clear chain of reasoning. Every step must be justified by a definition, postulate, or theorem.
As you work through your proofs, keep these points in mind:
Keep it Logical

Every step in your proof should logically follow from the previous steps. Jumping to conclusions or making assumptions can lead to errors.
Be Precise with Language

Use precise language. Instead of saying, “This line bisects this segment,” say, “Line l bisects segment AB at point M.”
Think about the Converse
Often, geometric statements have a converse. If a theorem says “If X, then Y,” sometimes you might need to prove “If Y, then X” or its converse.
Integrating Segment Relationships in Real-World Applications

Understanding segment relationships isn't just for passing geometry tests; it has real-world applications:
- Architecture: Proving that a structure's design elements are proportionally correct or symmetrical.
- Engineering: Ensuring precision in machine parts or structural stability in bridge designs.
- Computer Graphics: Creating realistic 3D models by ensuring accurate relationships between segments and angles.
- Surveying: Determining property lines and measurements with exactness.
💡 Note: The concepts of segment relationships are also crucial in other fields like physics, where forces or vectors in different directions need to be resolved into components.
In concluding, mastering segment relationships in proofs not only enhances your problem-solving skills but also deepens your appreciation for the structured beauty of geometry. By understanding these relationships, you can deduce properties of geometric figures, solve complex problems, and apply these principles in various real-world scenarios. Keep practicing, leveraging the strategies outlined here, and watch as your ability to unravel geometric puzzles grows.
Why are segment relationship proofs important?
+Segment relationship proofs are important because they help develop logical thinking, precision in argumentation, and an understanding of how geometric elements interact. These proofs are foundational in building a robust knowledge of geometry that can be applied in various practical contexts.
How can I improve my proof-writing skills?
+Regular practice is key. Work through different types of proofs, review your mistakes, and try to understand why certain steps are necessary. Study the theorems and their converses, use different proof formats, and read proofs by others to see varied approaches.
What are some common mistakes to avoid in segment relationship proofs?
+Common mistakes include assuming what needs to be proved, skipping steps without proper justification, misinterpreting diagrams, and not using definitions correctly. Always ensure each step logically follows from the previous one and is supported by definitions, postulates, or theorems.