Master Proving Parallel Lines: Engaging Worksheet with Answers

Discovering the concept of parallel lines not only enhances one's understanding of geometry but also aids in solving real-world architectural and design problems. Parallel lines, by definition, run in the same direction and never intersect, which makes them a fascinating subject in mathematical study. This blog post is dedicated to a comprehensive exploration of master proving parallel lines, providing an engaging worksheet with answers to enrich your learning experience.
Understanding Parallel Lines

Before diving into the worksheet, let’s grasp the basics:
- Parallel Lines: Two lines in a plane that do not intersect or touch at any point, no matter how far extended.
- Properties: They have the same slope and are equidistant from each other at every point along their length.
- Importance in Geometry: Parallel lines are fundamental in many geometric theorems and are crucial for understanding more complex geometric structures.
Proving Lines Parallel

To prove that two lines are parallel, several theorems or postulates can be utilized:
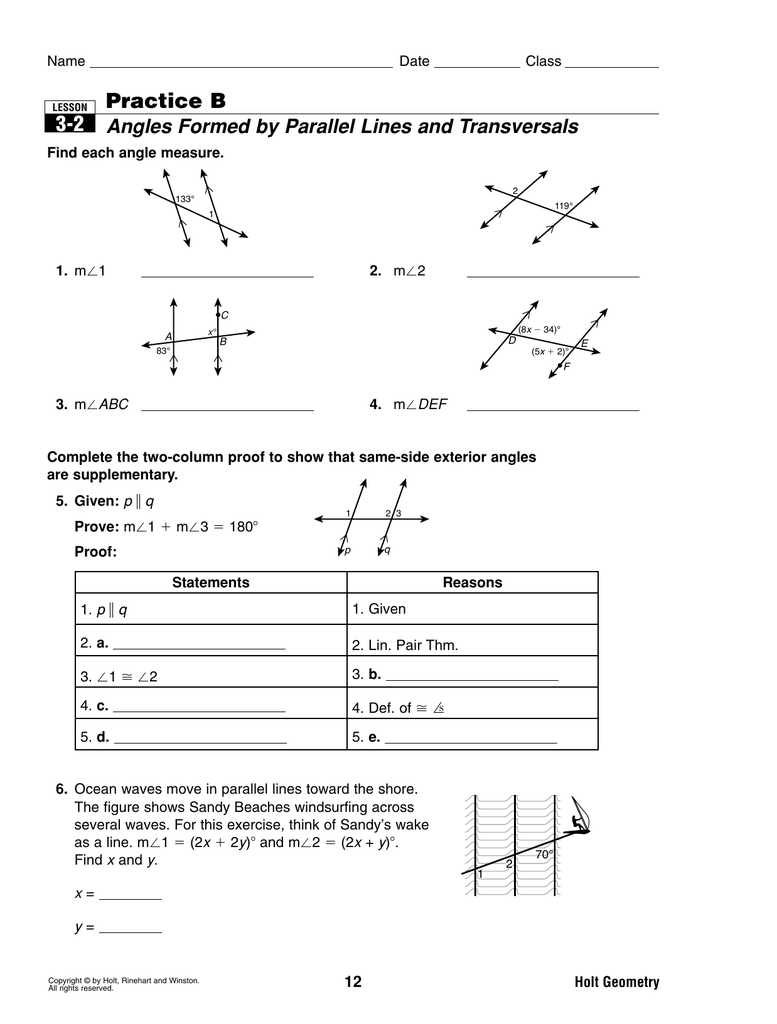
- The Corresponding Angles Postulate: If two lines are cut by a transversal and the corresponding angles are congruent, the lines are parallel.
- The Alternate Interior Angles Theorem: If the alternate interior angles between two lines are equal, then the lines are parallel.
- The Consecutive Interior Angles Theorem: Also known as the Co-interior or Same-Side Interior Angles Theorem. If the sum of the consecutive interior angles is 180 degrees, the lines are parallel.
Worksheet on Proving Parallel Lines

Here’s an engaging worksheet to help solidify your understanding of parallel lines:
| Problem | Proof |
|---|---|
| 1. Given: ∠3 ≅ ∠7, prove that lines l and m are parallel. |
Proof:
|
| 2. Given: ∠2 ≅ ∠8, prove that lines l and m are parallel. |
Proof:
|
| 3. Given: m∠4 + m∠6 = 180°, prove that lines l and m are parallel. |
Proof:
|

📝 Note: The key to mastering these proofs is recognizing which angles relate to parallel lines through transversals. Understanding the angles formed by transversals can make solving these proofs much easier.
Challenges and Advanced Concepts
Once you’ve tackled the basic proofs, here are some challenges to deepen your understanding:
- Parallel Lines in 3D: Proving parallel lines in three-dimensional space using vector methods.
- Slopes and Coordinates: Using the slope-intercept form of a line to determine parallelism in coordinate geometry.
- Proof by Contradiction: Proving lines parallel by assuming they are not and finding a contradiction.
🔍 Note: These advanced concepts can be quite challenging but offer a deeper understanding of how parallel lines interact with different mathematical contexts.
Understanding and proving parallel lines is not just an academic exercise; it has real-world applications in architecture, engineering, and design. By mastering these proofs, you not only enhance your geometric knowledge but also develop critical thinking and logical reasoning skills.
What are parallel lines?

+
Parallel lines are lines in a plane that do not intersect or cross each other at any point, meaning they always remain the same distance apart.
How do you prove lines are parallel?
+
Lines can be proven parallel through several methods like the Corresponding Angles Postulate, Alternate Interior Angles Theorem, or Consecutive Interior Angles Theorem when cut by a transversal.
Can you use algebra to show lines are parallel?

+
Yes, in coordinate geometry, if the slopes of two lines are equal, they are parallel, assuming they are not the same line (different y-intercepts).