Proportions Worksheet Answers: 5 Key Solutions Unveiled

The topic of proportions can be challenging yet interesting for many students. Understanding how to answer questions related to ratios and proportions effectively requires a firm grasp of the underlying mathematical concepts. In this extensive guide, we will explore five key solutions to common proportion problems that appear frequently in worksheets and quizzes. Each solution will delve into various techniques and common pitfalls, offering clear steps to navigate these mathematical scenarios.
Proportion Basics

To begin with, let’s clarify what a proportion is. A proportion is an equation that states that two ratios are equivalent. When dealing with proportions, the critical skill is to determine whether two ratios are proportional to each other, which can be done by cross-multiplying.
- Example: If the ratio of apples to oranges is 3:4, and we want to find out how many oranges we have if there are 6 apples, we set up the proportion like this: 3⁄4 = 6/x.
Key Solution #1: Cross Multiplication

Cross multiplication is a fundamental technique when solving for unknown quantities in a proportion.
- Write the proportion as a fraction equation.
- Multiply diagonally.
- Solve for the unknown value.
🍏 Note: Cross multiplication works because if two fractions are equal, then their cross products are also equal.
Key Solution #2: Rate Problem Proportions

Rate problems involve finding the speed, cost, or productivity per unit. Here’s how to solve these:
- Set up the ratio of the known quantities in one column, and the ratio of the unknown quantities in another.
- Use the method of cross-multiplication to find the missing quantity.
| Speed (Miles per Hour) | Time (Hours) | Distance (Miles) |
|---|---|---|
| 30 | 2 | 60 |
| x | 3 | 90 |

⏱️ Note: This method not only applies to speed but also to cost or work rate problems.
Key Solution #3: Using a Proportion Chart
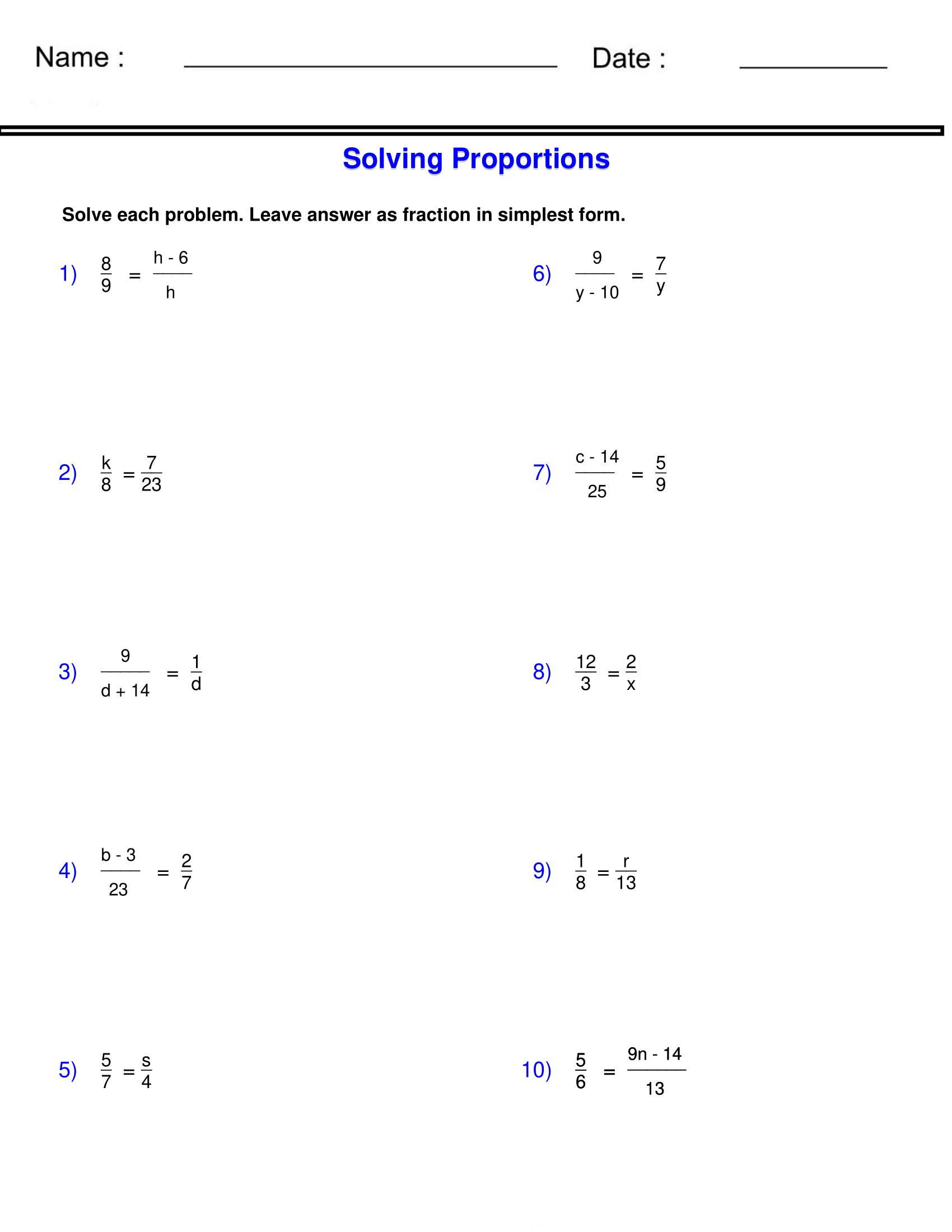
Sometimes, using a chart or diagram can help visualize proportions:
- List out the quantities you know and those you need to find.
- Draw arrows or lines to connect equivalent ratios.
- Calculate to find the missing value.

Key Solution #4: Inverse Proportions

Not all proportions are directly related; some are inversely proportional. Here’s how to address them:
- Identify the relationship (if one increases, the other decreases).
- Set up the proportion with the formula xy = k, where k is a constant.
- Solve for one variable when the other is known.
Key Solution #5: Proportions in Geometry

Proportions often appear in geometric problems like similar triangles or scale drawings:
- Identify the corresponding sides in similar figures.
- Set up the proportion between the corresponding sides.
- Solve for the unknown length or scale factor.
📐 Note: Proportions in geometry help in understanding similarity, which is crucial in many areas of math and science.
In this extensive guide, we've walked through five critical solutions for solving proportions effectively. Each method has its advantages and unique applications, from the basic cross-multiplication to dealing with complex geometric problems. Understanding these techniques not only aids in solving proportion problems but also enhances overall mathematical fluency. Whether you're comparing speeds, working on cost analysis, or dissecting geometric shapes, the principles of proportions remain consistent, providing a versatile tool for various mathematical applications.
What is the difference between a direct and inverse proportion?

+
In a direct proportion, when one quantity increases, the other also increases at the same rate. In contrast, with an inverse proportion, when one quantity increases, the other decreases.
Why do we use cross multiplication in proportions?

+
Cross multiplication simplifies the process of determining if two ratios are equal. If a/b = c/d, then a×d = b×c.
Can proportions help in real-life applications?

+
Absolutely! Proportions are used in cooking to scale recipes, in finance for cost calculations, in engineering for scaling models, and in many other areas where ratios are involved.