Prime Factoring Worksheet: Master Math Skills Easily
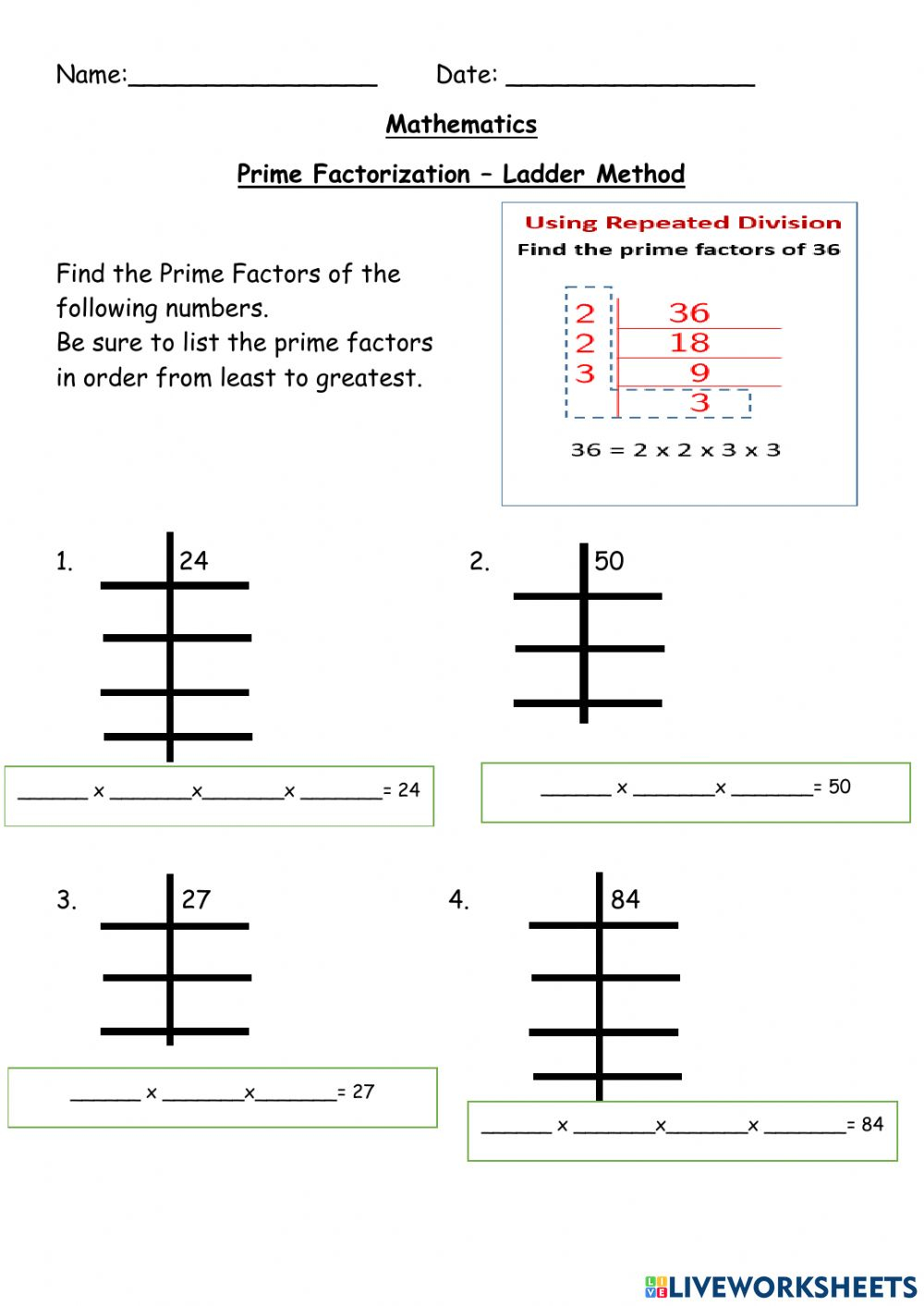
Prime factorization is a fundamental concept in arithmetic that breaks down a number into its prime factors. This process not only enhances your understanding of numbers but also lays the groundwork for various mathematical operations, including solving algebraic equations and simplifying fractions. If you're eager to master prime factoring, this comprehensive worksheet and guide will walk you through the essential steps and techniques to do so effectively.
What is Prime Factorization?


Prime factorization involves expressing a number as a product of its prime factors. A prime number is a whole number greater than one that can only be divided by itself and 1 without a remainder. Here’s a simple example to understand:
- The prime factors of 12 are 2 x 2 x 3.
Prime factorization helps in simplifying numbers, finding the greatest common divisor, and other number theory problems.
How to Prime Factorize

1. Understand the Concept

Before you start, ensure you know what a prime number is. The numbers 2, 3, 5, 7, 11, and so forth are all prime numbers.
2. Use the Division Method

This method involves continuously dividing the number by the smallest prime number that divides it evenly:
- Start with the smallest prime number (2), and divide the target number by it as many times as possible.
- Move to the next smallest prime number (3, then 5, etc.), and repeat the process.
- Record each prime factor in multiplication form.
📚 Note: If you divide by 2 repeatedly, remember to count how many times you’ve divided before moving to the next prime.
3. Use Factor Trees or Lattices

An alternative method is the factor tree, where you:
- Start with the number at the top.
- Break it down into two factors, then continue until all factors are prime.
This visual representation can help in understanding the factorization process.
Practice with Examples

Let’s work through a couple of examples to cement your understanding:
Example 1: Factorize 100

Using the division method:
- 100 ÷ 2 = 50 (so we have one 2)
- 50 ÷ 2 = 25 (so we have another 2)
- 25 ÷ 5 = 5 (so we have one 5)
- 5 ÷ 5 = 1 (so we have another 5)
Therefore, the prime factorization of 100 is 2 × 2 × 5 × 5 or 22 × 52.
Example 2: Factorize 48

Using a factor tree:
| 48 | ||||
| 24 | 2 | |||
| 12 | 2 | 2 | ||
| 6 | 2 | 2 | 2 | |
| 3 | 2 | 2 | 2 | 3 |

The prime factorization is 2 × 2 × 2 × 2 × 3 or 24 × 3.
Prime Factorization Tips and Techniques

To become proficient in prime factorization, consider these tips:
- Memorize small prime numbers: This helps you to quickly identify if a number is divisible.
- Know divisibility rules: For example, a number is divisible by 3 if the sum of its digits is divisible by 3.
- Use factors for efficiency: Sometimes, using known factors can speed up the process. If you know that 15 is a factor of 30, then you don’t need to divide by smaller primes repeatedly.
- Practice regularly: The more you practice, the more intuitive the process will become.
In wrapping up our journey through prime factorization, remember that understanding and practicing these methods will not only help you in solving math problems but also enrich your mathematical insight. The ability to break down numbers into their prime factors is invaluable in algebra, number theory, and even in daily life when dealing with calculations and patterns. Keep in mind that proficiency comes with practice, so continue to factorize numbers and challenge yourself with more complex examples to sharpen your skills.
Why is prime factorization useful?

+
Prime factorization is essential for simplifying fractions, finding the greatest common divisor (GCD), and solving certain types of algebraic equations. It also helps in understanding the nature of numbers and their properties.
Can all numbers be prime factorized?

+
Yes, every integer greater than 1 can be expressed as a product of prime numbers in a unique way, except for the number itself if it’s prime.
What are some challenges in prime factorization?

+
Large numbers can make prime factorization cumbersome, especially for numbers that don’t have small prime factors. This is where computational algorithms like the Quadratic Sieve or the General Number Field Sieve become necessary for efficiency.