Master Congruent Triangles with Our Easy Practice Worksheet

Congruent triangles form the bedrock of many geometric proofs and real-world applications, providing the groundwork for understanding shapes, measurements, and symmetry. For those stepping into the world of mathematics or refining their skills, mastering the congruence of triangles is essential. This blog post dives deep into the concept of congruent triangles, offering not only a robust understanding of what they entail but also providing practical methods to identify, prove, and apply these geometric figures in various contexts.
Congruent Triangles Defined

Two triangles are considered congruent if they have the same size and shape. This means all their corresponding angles are equal, and their corresponding sides are of identical length. However, proving triangle congruence typically involves showing that enough parts of the triangles are the same. Here are the five major methods for proving triangle congruence:
- Side-Side-Side (SSS) Congruence Postulate: If all three sides of one triangle are congruent to three sides of another triangle, then the triangles are congruent.
- Side-Angle-Side (SAS) Congruence Postulate: If two sides and the included angle of one triangle are congruent to two sides and the included angle of another triangle, the triangles are congruent.
- Angle-Side-Angle (ASA) Congruence Postulate: If two angles and the included side of one triangle are congruent to two angles and the included side of another triangle, then the triangles are congruent.
- Angle-Angle-Side (AAS) Congruence Theorem: If two angles and a non-included side of one triangle are congruent to two angles and the corresponding non-included side of another triangle, then the triangles are congruent.
- Hypotenuse-Leg (HL) Congruence Theorem: This applies to right triangles only. If the hypotenuse and a leg of one right triangle are congruent to the hypotenuse and a leg of another right triangle, the triangles are congruent.
🔍 Note: The congruence criteria listed above are useful tools for identifying congruent triangles but remember that the order of letters in the acronyms (e.g., SAS, ASA) matters; it represents how the congruent parts are arranged in relation to each other.
How to Identify Congruent Triangles?
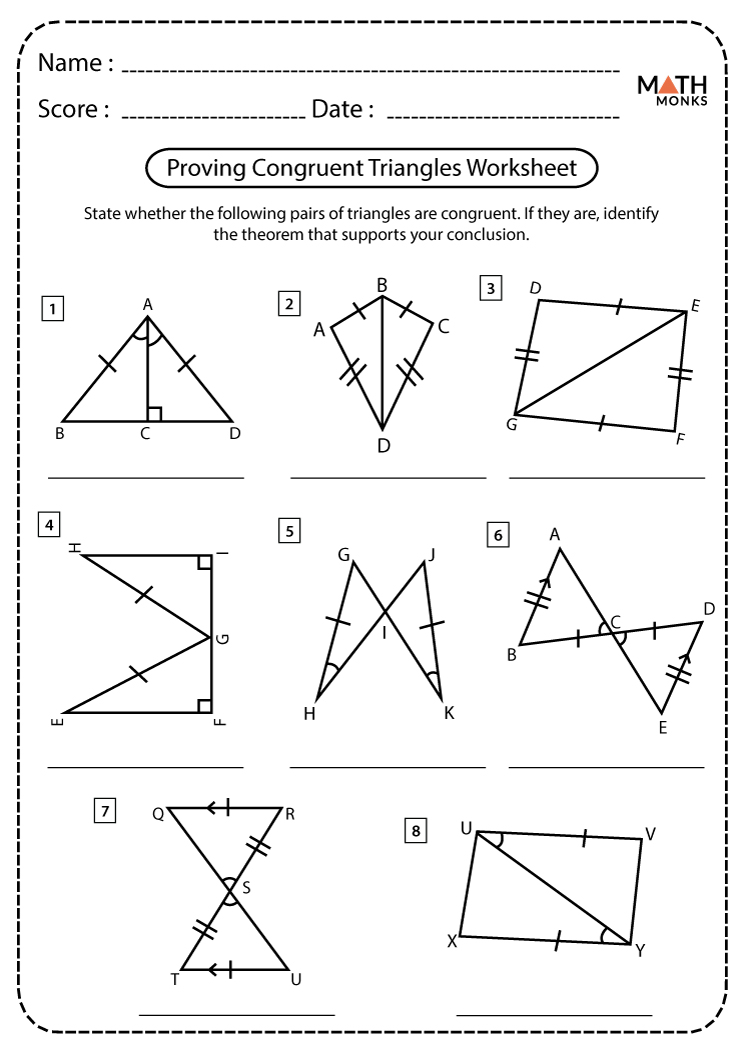
Identifying congruent triangles can be an intuitive process, but here are steps you can follow to ensure accuracy:
- Understand the Postulates: Familiarize yourself with the congruence postulates and theorems listed above.
- Compare Corresponding Parts: Identify which sides or angles in one triangle could correspond to those in the other. Use labels, if provided, or assume logical correspondence based on the diagram.
- Check for Congruence Conditions: Once corresponding parts are identified, check if they meet any of the congruence criteria mentioned earlier.
- Use Congruence Symbols: If the triangles are congruent, use the congruence symbol (≅) to denote the relationship.
Proving Congruence

To prove that two triangles are congruent, you often need to provide a formal proof. Here’s a simple example using the SAS Congruence Postulate:
- Given: Triangle ABC with AB = DE, AC = DF, and ∠BAC = ∠EDF.
- Prove: ∆ABC ≅ ∆DEF
The proof would proceed as follows:
- State the given information.
- Use the SAS Congruence Postulate to assert that the triangles are congruent.
- Write the formal statement: Since AB = DE, AC = DF, and ∠BAC = ∠EDF, by the SAS Congruence Postulate, ∆ABC ≅ ∆DEF.
This kind of proof is fundamental in geometry because it’s both rigorous and universally applicable to countless problems.
Real-World Applications of Congruent Triangles

Congruent triangles aren’t just for textbooks; they have numerous real-world applications:
- Architecture: Ensuring that symmetrical elements are congruent ensures aesthetic balance and structural integrity.
- Manufacturing: From car parts to electronics, congruent shapes ensure consistency in product assembly.
- Navigation: In surveyor triangulation, establishing congruent triangles helps in accurately determining distances and positions.
- Physics: In calculating forces and torque in systems, congruent triangles play a role in force diagrams.
🔧 Note: Congruent triangles can simplify complex systems by breaking them down into simpler, calculable segments.
Visualizing Congruent Triangles

Creating visual aids like diagrams can greatly help in understanding and teaching congruent triangles. Here’s a simple table to illustrate how congruent parts are matched:
| Triangle ABC | Congruent Part | Triangle DEF |
|---|---|---|
| AB | ≅ | DE |
| AC | ≅ | DF |
| BC | ≅ | EF |
| ∠BAC | ≅ | ∠EDF |
| ∠ABC | ≅ | ∠DEF |
| ∠ACB | ≅ | ∠DFE |

With a strong foundation in understanding and applying the principles of congruent triangles, you'll find that many geometric puzzles and real-world problems become not only solvable but also comprehensible. Congruent triangles open doors to further exploration in geometry, trigonometry, and beyond, making them a pivotal topic in mathematical education.
What does it mean when two triangles are congruent?

+
When two triangles are congruent, it means that all corresponding sides and angles of the two triangles are equal. This implies that they have the same shape and size, and one triangle can be rotated, flipped, or slid to fit exactly over the other.
Can I prove triangle congruence with only two corresponding sides equal?
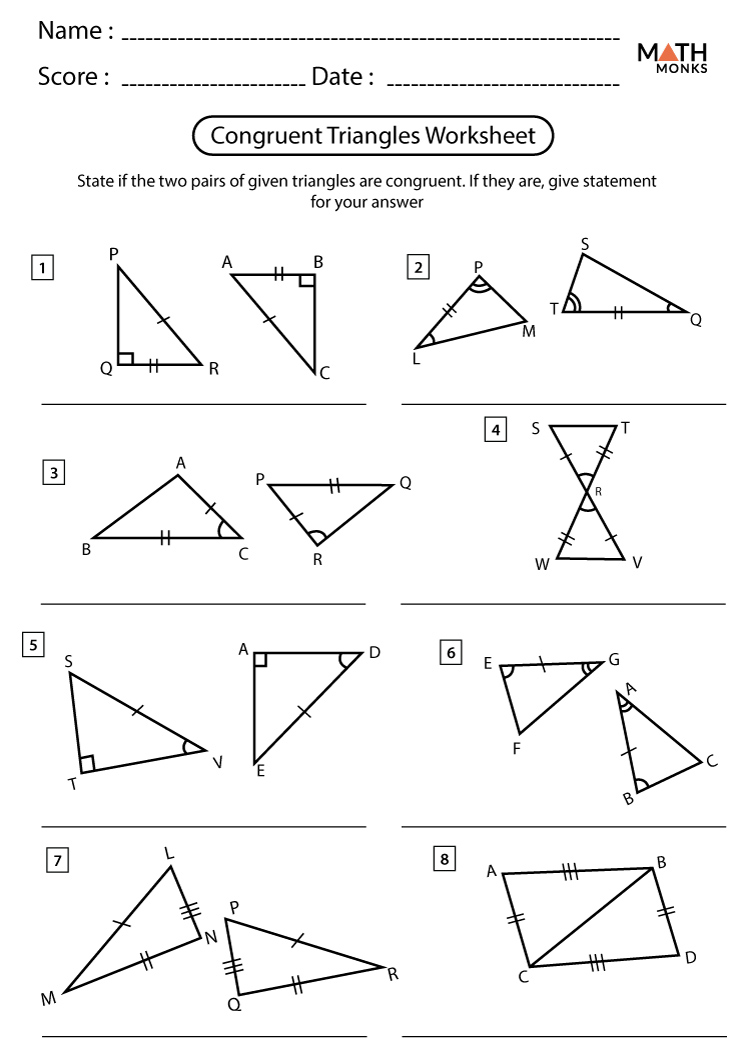
+
Having two corresponding sides equal is not sufficient to prove congruence unless you have additional information like the included angle (SAS) or the angles around those sides (AAS or ASA).
How does the concept of congruent triangles apply to real-world scenarios?

+
Congruent triangles are essential in fields like architecture, manufacturing, and navigation where precision and consistency are key. For example, in architecture, congruent triangles ensure symmetrical design elements.
What are some common mistakes when identifying congruent triangles?

+
Common errors include assuming triangles are congruent when only two sides are equal without checking angles, or misapplying congruence postulates, such as using AAS when the side information is not sufficient.