Kinetic and Potential Energy Worksheet Answers Revealed

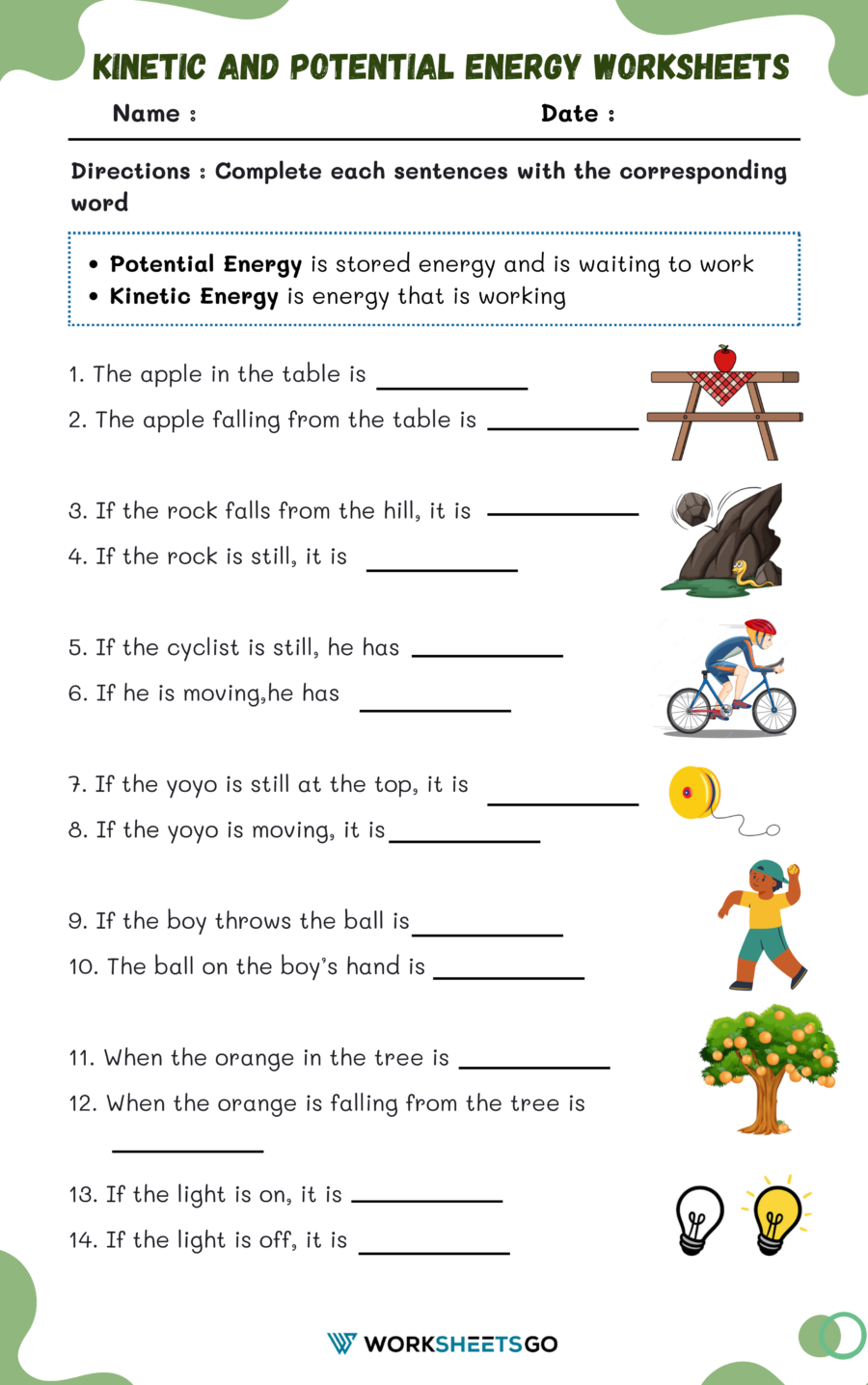
Understanding the concepts of kinetic and potential energy is crucial for anyone studying physics or simply interested in how energy behaves in different systems. This blog post aims to delve deep into these energies, providing kinetic and potential energy worksheet answers alongside detailed explanations to enhance comprehension. Whether you are a student, teacher, or enthusiast, this guide will equip you with the knowledge needed to grasp these foundational physics principles.
Understanding Kinetic Energy

Kinetic energy is the energy of motion. Any object that is moving has kinetic energy, which can be expressed by the equation:
\[ KE = \frac{1}{2}mv^2 \]
- m represents the mass of the object in kilograms (kg).
- v represents the velocity of the object in meters per second (m/s).
Here are some key points to remember:
- The kinetic energy of an object increases exponentially with its velocity. This means doubling the speed will quadruple the kinetic energy.
- Work done on an object increases its kinetic energy.
- Kinetic energy depends solely on the mass and speed of an object, not its direction or type of motion.
Examples and Worksheet Questions

Let's look at some common problems from kinetic energy worksheets:
Question 1:
Calculate the kinetic energy of a 10 kg object moving at 5 m/s.
Answer:
\[ KE = \frac{1}{2} \times 10 \times 5^2 \]
\[ KE = 125 J \]
Question 2:
An object has a kinetic energy of 200 J. If its mass is 5 kg, what is its velocity?
Answer:
\[ 200 = \frac{1}{2} \times 5 \times v^2 \]
\[ 200 = 2.5 v^2 \]
\[ v^2 = 80 \]
\[ v = \sqrt{80} \approx 8.944 m/s \]
💡 Note: Remember to check your units to ensure your answer is consistent.
Understanding Potential Energy
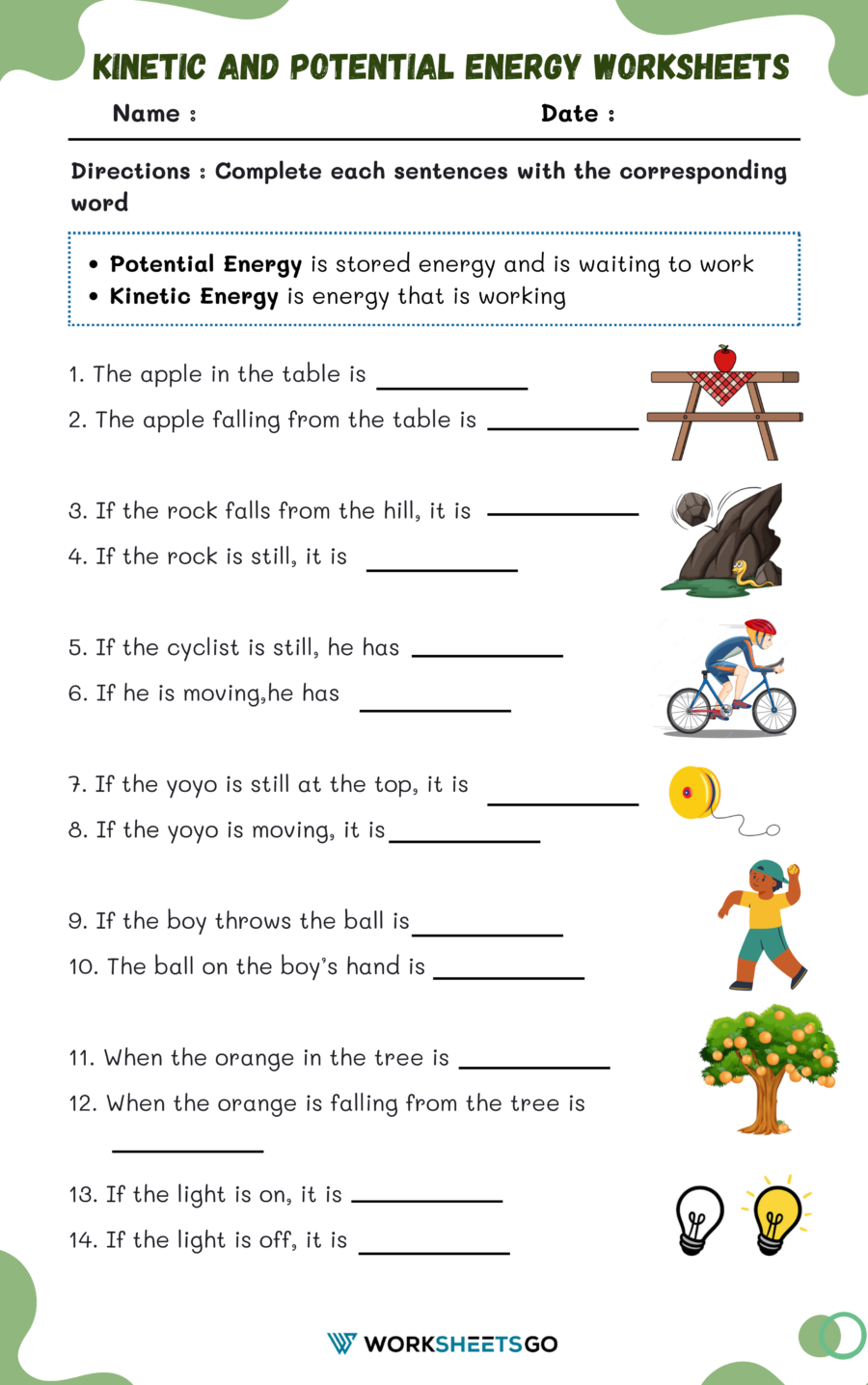
Potential energy is energy that is stored within an object, not in motion. There are several types of potential energy, but we will focus on gravitational potential energy, which is given by:
\[ PE = mgh \]
- m is the mass of the object in kilograms (kg).
- g is the acceleration due to gravity, approximately 9.8 m/s² on Earth.
- h is the height above a reference point in meters (m).
Examples and Worksheet Questions

Question 1:
Find the gravitational potential energy of a 5 kg object at a height of 10 meters from the ground.
Answer:
\[ PE = 5 \times 9.8 \times 10 \]
\[ PE = 490 J \]
Question 2:
An object of unknown mass has a gravitational potential energy of 1000 J at a height of 25 meters. Determine its mass.
Answer:
\[ 1000 = m \times 9.8 \times 25 \]
\[ 1000 = 245m \]
\[ m = \frac{1000}{245} \approx 4.08 kg \]
💡 Note: Gravitational potential energy can also be calculated for objects below ground level or in a vacuum, keeping the reference point the same.
Comparison Between Kinetic and Potential Energy
Both kinetic and potential energy are types of mechanical energy, but they differ in how they manifest:
- Kinetic Energy: Depends on motion, affects objects as they move. It decreases when objects slow down or stop moving.
- Potential Energy: Depends on position or configuration. It increases when an object moves higher or is further away from the ground (in case of gravitational potential energy).
| Energy Type | Definition | Formula |
|---|---|---|
| Kinetic | Energy of motion | \[ \frac{1}{2}mv^2 \] |
| Gravitational Potential | Energy due to height | \[ mgh \] |

The Law of Conservation of Energy

This law states that energy cannot be created or destroyed, only transformed from one form to another. Here's how this plays out in kinetic and potential energy:
- In an ideal, frictionless system, the sum of kinetic and potential energy remains constant (total mechanical energy is conserved).
- Energy can convert from potential to kinetic and vice versa. For example, a pendulum swinging back and forth or a roller coaster moving up and down.
- In real-world scenarios, some kinetic energy might be converted to heat due to friction, reducing the total mechanical energy.
💡 Note: Energy losses due to friction, air resistance, or other forms of work done on or by the system need to be considered for accurate calculations.
After exploring these fundamental concepts, we've covered how to solve common worksheet problems involving kinetic and potential energy, provided definitions, formulas, and an explanation of energy conservation. The distinction between kinetic and potential energy, their calculations, and their interplay in real-life systems give us a comprehensive understanding of how energy behaves in different scenarios. Recognizing these principles helps not only in solving textbook problems but also in understanding everyday physics from sports to space travel.
Remember that while our focus was on kinetic and gravitational potential energy, there are other forms like elastic, chemical, electrical, nuclear, and more. Understanding these energy forms and their interactions can provide an even deeper insight into the physical world around us.
What is the relationship between work and kinetic energy?

+
The relationship between work and kinetic energy is expressed through the work-energy theorem, which states that the net work done on an object is equal to the change in its kinetic energy. If work is done on an object, increasing its speed, its kinetic energy will increase by the same amount. Conversely, if work is done against the object (slowing it down), its kinetic energy decreases.
Can potential energy be negative?
+
Gravitational potential energy can indeed be negative. This occurs when the reference point for zero potential energy is set above the object’s position. For example, if the reference is ground level and an object is underground, its potential energy will be negative since it would require work to bring it back to ground level.
How do kinetic and potential energy relate to the conservation of energy?

+
In an isolated system, the sum of kinetic and potential energies is constant if no non-conservative forces (like friction) do work on the system. This principle is known as the conservation of mechanical energy. As an object moves through a system, energy transfers between kinetic and potential forms but the total mechanical energy remains unchanged unless external forces act upon it.