5 Ways to Master Polygon Similarity Fast

Understanding polygon similarity is a cornerstone in the field of geometry, offering insights into the proportions, congruence, and similarity of shapes. Whether you are a student struggling with geometry homework or an architect designing scale models, mastering polygon similarity can significantly enhance your problem-solving skills and spatial reasoning. Here, we'll explore five effective strategies to quickly grasp the concept of polygon similarity, ensuring that you can confidently tackle any related problem.
1. Identify Corresponding Angles and Sides


The first step towards mastering polygon similarity is to recognize that similar polygons have corresponding angles that are congruent and corresponding sides that are proportional. Here’s how you can approach this:
- List the angles of each polygon in order.
- Compare these lists to find if the angles correspond.
- Check if the sides opposite these angles are proportional. If they are, then the polygons are similar.
For example, in two triangles, if ∠A = ∠D, ∠B = ∠E, and ∠C = ∠F, and sides AB/DE = AC/DF = BC/EF, these triangles are similar.
2. Use the Similar Triangle Theorem


One of the most common polygons you’ll deal with is triangles, and the Similar Triangle Theorem can be your fast track to verifying similarity. This theorem states:
- If two angles of one triangle are congruent to two angles of another triangle, the triangles are similar by the Angle-Angle (AA) criterion.
- If all corresponding sides are proportional, the triangles are similar by the Side-Side-Side (SSS) criterion.
- If two pairs of corresponding sides are proportional and the included angles are congruent, the triangles are similar by the Side-Angle-Side (SAS) criterion.
💡 Note: When applying these criteria, always ensure you’re comparing corresponding parts correctly to avoid common mistakes.
3. Understand Scale Factors and Ratios


The scale factor is a fundamental concept in similarity, where each dimension of one polygon is enlarged or reduced by a constant ratio to match the other. Here’s how to work with scale factors:
- Determine the ratio of one corresponding side to another. This ratio is your scale factor.
- Apply this ratio to all other sides to verify proportionality across the polygons.
- Calculate areas using the square of the scale factor, and volumes using the cube of the scale factor for solids.
For example, if the ratio of side lengths of two similar polygons is 2:3, the area ratio would be 4:9.
4. Use the Geometric Mean and Indirect Measurement
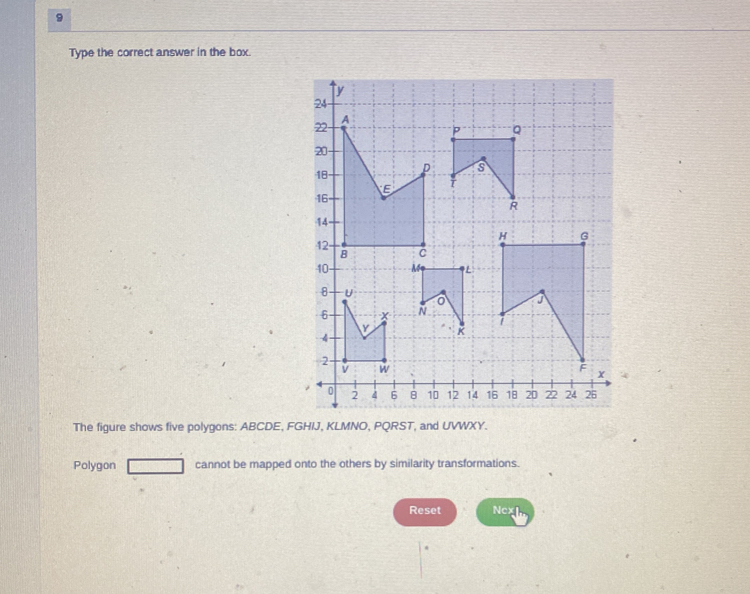

Sometimes, direct measurement isn’t possible or practical. This is where the geometric mean and indirect measurement come into play:
- Understand the Geometric Mean Theorem for similar triangles: The length of the altitude to the hypotenuse of a right triangle is the geometric mean of the lengths of the two segments of the hypotenuse.
- Apply this theorem to solve for unknown sides when heights or other lengths are needed.
For instance, if you have a right triangle with segments a and b along the hypotenuse, and the altitude to the hypotenuse h, then h is the geometric mean of a and b, i.e., h = √(a*b).
5. Practice with Real-World Applications


Applying polygon similarity to real-world scenarios can solidify your understanding:
- Map scaling: Cities often use scale models for planning and development.
- Architecture: Architects use scale drawings to ensure designs are practical and aesthetically pleasing when built at full size.
- Surveying: Land surveyors use similar triangles to calculate distances and heights without physically measuring every part.
- Photography: Understanding how objects scale in photographs can help photographers with perspective correction.
By engaging with these applications, you not only practice geometry but also appreciate its practical implications.
By following these five strategies, you'll gain a robust understanding of polygon similarity, which will not only improve your academic performance but also empower you in practical scenarios where geometry plays a critical role. Remember, practice and application are key. The more you apply these principles, the more intuitive and second nature they become. As you encounter various shapes and proportions in your daily life, you'll find yourself instinctively recognizing patterns of similarity, making geometry an ever-present, helpful tool in your mental toolkit.
What does it mean for two polygons to be similar?

+
Two polygons are similar if they have the same shape but not necessarily the same size. This means corresponding angles are congruent, and the lengths of corresponding sides are proportional.
How can I quickly check if two triangles are similar?

+
Use the AA, SSS, or SAS similarity criteria. If any two of these conditions are met, the triangles are similar:
- AA: If two angles of one triangle are congruent to two angles of another.
- SSS: If all three sides of one triangle are proportional to the sides of the other.
- SAS: If two pairs of sides are proportional, and the included angles are congruent.
Why is understanding polygon similarity important in architecture?

+
In architecture, similarity allows for the creation of scale models. These models help architects visualize the design in a controlled environment, ensure proportions are correct, and communicate design ideas effectively with clients and builders before full-scale construction begins.
Can polygons other than triangles be similar?

+
Yes, all polygons can be similar. For example, two quadrilaterals are similar if their corresponding angles are congruent and their corresponding sides are proportional.