Explore Geometry: Points, Lines, Planes Worksheet Guide

Geometry is a fundamental branch of mathematics that delves into the study of shapes, sizes, properties of space, and the relationships between different shapes. For students, teachers, and enthusiasts, understanding geometry often begins with the basic elements of points, lines, and planes. This guide will explore these geometric entities in detail, providing a comprehensive worksheet guide to facilitate learning and application of these concepts in various contexts.
Understanding Points

A point in geometry is often described as having a location but no size. It is a basic unit of geometry, representing a precise position in space. Here's how to conceptualize and work with points:
- Definition: A point is an exact location in space that has no length, width, or depth.
- Notation: Points are typically labeled with capital letters (e.g., A, B, C).
- Collinear Points: Points that lie on the same straight line.
- Distance: The distance between two points is the shortest path between them, usually measured using the Euclidean distance formula.

Worksheet Ideas:
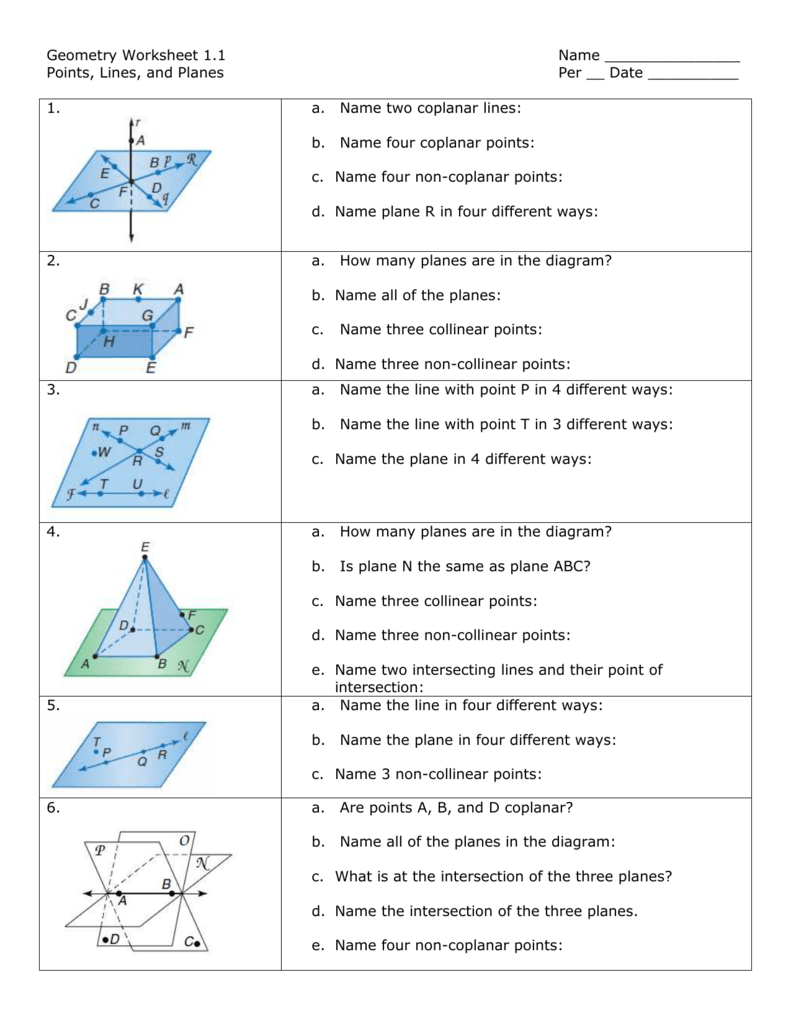
- Identify Points: Show students a diagram with various geometric shapes and ask them to identify and label points.
- Calculate Distance: Provide coordinates for pairs of points and have students calculate the distances between them.
📌 Note: Points can exist in any dimension, from one-dimensional lines to three-dimensional spaces, expanding their utility in geometric studies.
Exploring Lines

A line is an infinitely extending set of points in a single direction without any thickness or width. Here’s a deeper look into lines:
- Definition: A line is a one-dimensional figure that extends infinitely in both directions.
- Notation: Lines are named with letters (e.g., line AB, where A and B are points on the line) or with a single lowercase letter like "l".
- Parallel Lines: Lines that never intersect.
- Intersecting Lines: Lines that cross at one point.
- Perpendicular Lines: Lines that intersect at right angles (90°).

Worksheet Ideas:
- Identify Line Types: Include diagrams where students must identify parallel, intersecting, and perpendicular lines.
- Determine Slope: Given two points, have students find the slope of the line passing through them.
| Type of Lines | Characteristics |
|---|---|
| Parallel | Never intersect, have equal slopes |
| Intersecting | Meet at a single point |
| Perpendicular | Intersect at right angles (90°) |
📌 Note: Lines can be used to form shapes like triangles and quadrilaterals, which are further studied in geometry.
Understanding Planes

A plane in geometry is a flat, two-dimensional surface that extends infinitely in all directions. Here's what you need to know:
- Definition: A plane is an infinitely extending flat surface where thickness is zero.
- Notation: Planes are often named by three points lying on it or with a single uppercase letter.
- Properties: Planes have area but no volume; they can intersect with lines and other planes.
- Co-planar Points: Points that lie in the same plane.

Worksheet Ideas:
- Identify Planes: Provide diagrams with points and lines, asking students to identify the plane or planes they lie on.
- Determine Intersections: Include problems where students must determine how lines or planes intersect.
By working through these exercises, students not only grasp the concepts but also understand how these entities interact in geometric spaces.
The journey through geometric exploration has now brought us to the core elements of geometry - points, lines, and planes. Each of these elements has its unique characteristics and interplay that forms the foundation of spatial understanding. From the precise location of points to the infinite extension of lines and the flat expanse of planes, these basic components allow us to explore and analyze complex shapes and spaces. By utilizing worksheets focused on these elements, we enhance our visualization and analytical skills, making geometry not just a subject of study but a tool for understanding the world around us. We've covered definitions, notations, properties, and practical exercises, preparing you to delve deeper into the world of geometry or to teach these concepts effectively.
How do you define a point in geometry?

+
A point in geometry is defined as an exact location in space with no dimension; it has no length, width, or depth.
What are collinear points?

+
Collinear points are points that lie on the same straight line.
Can you have intersecting lines without an intersection point?

+
No, intersecting lines by definition meet at least at one point. If lines are parallel, they do not intersect.
What is the importance of planes in geometry?

+
Planes are fundamental in geometry as they provide the foundation for many geometric shapes, represent flat surfaces, and are crucial in understanding 3D space.