Master Point Slope Form with Our Engaging Practice Worksheet

The point slope form of a line's equation is an essential tool in algebra, used to represent a linear relationship with distinct clarity. Understanding and mastering this form can significantly aid in solving numerous mathematical problems, particularly those involving lines on a graph. This blog post will delve into the intricacies of the point slope form, explain why it's useful, and provide a practice worksheet designed to cement your understanding.
What is Point Slope Form?

The point slope form of a line's equation is given by:
y - y₁ = m(x - x₁)
- m: The slope of the line
- (x₁, y₁): A specific point on the line
📝 Note: This form is particularly useful when you know both a point on the line and its slope.
Why Use Point Slope Form?

Point slope form offers several advantages:
- Direct Calculation: It directly uses the slope and a point, making the computation straightforward.
- Versatility: Easily converted to other forms like slope-intercept or standard form.
- Intuitive: Clearly shows the relationship between the slope and a point, which can be intuitive for students learning about lines.
Steps to Find the Equation Using Point Slope Form
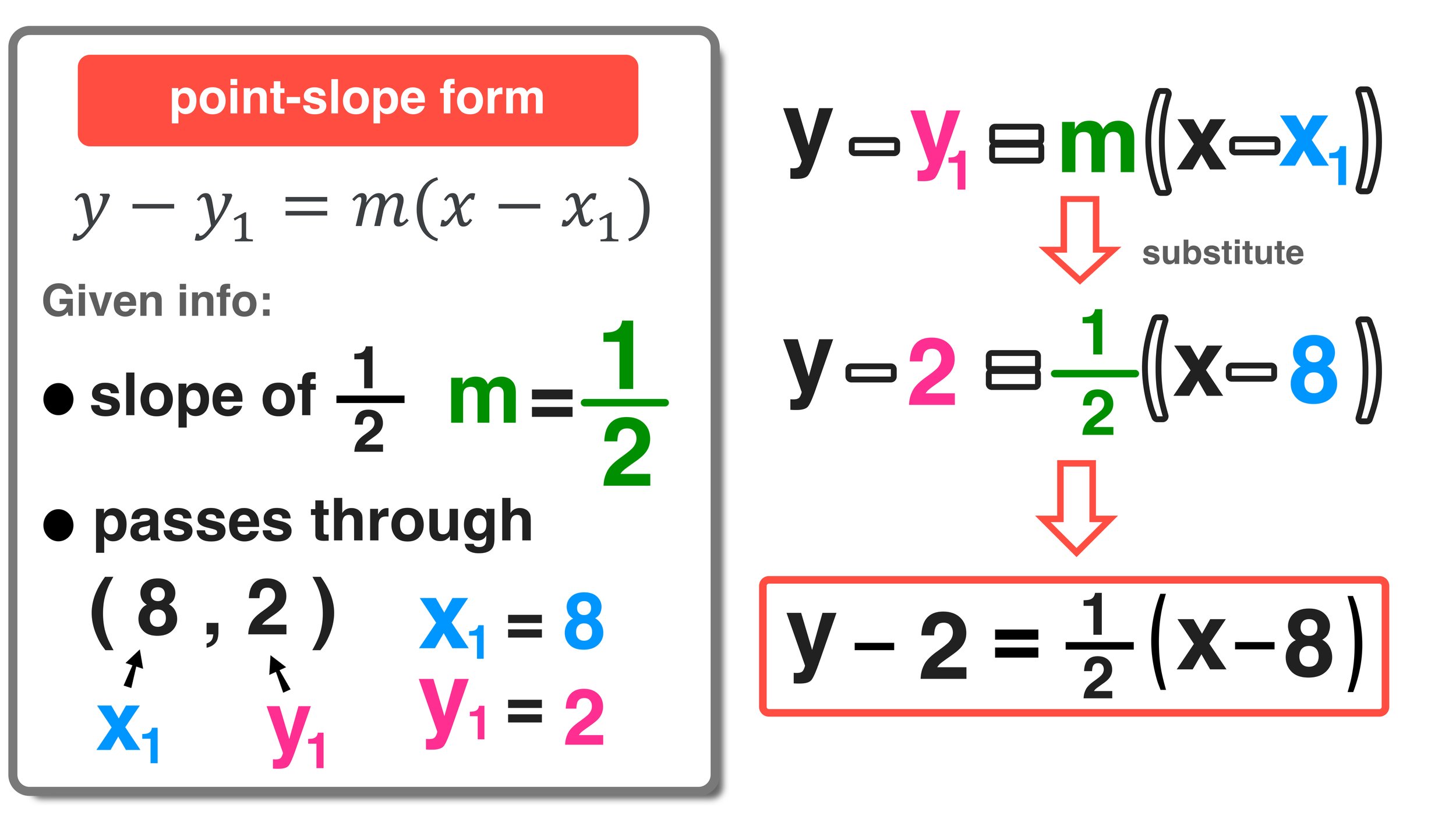
Here's how you can determine the equation of a line using the point slope form:
- Identify Slope (m): Use the change in y divided by the change in x.
- Choose a Point: You need one point on the line, (x₁, y₁).
- Substitute: Substitute m, x₁, and y₁ into the equation y - y₁ = m(x - x₁).
- Simplify: If necessary, simplify the equation to represent the line.
📝 Note: Ensuring you work with the correct slope is crucial for accurate results.
Practice Worksheet

To better understand point slope form, here is a practice worksheet with exercises:
| Problem | Solution |
|---|---|
| Find the equation of the line with slope 2 passing through (1, 3). | y - 3 = 2(x - 1) y - 3 = 2x - 2 y = 2x + 1 |
| A line with a slope of -1/3 that passes through (3, -2). | y - (-2) = -1/3(x - 3) y + 2 = -1/3x + 1 y = -1/3x - 1 |

Common Mistakes to Avoid

Here are some frequent errors to steer clear of when working with point slope form:
- Negative Signs: Forgetting to account for negative values when substituting into the equation.
- Incorrect Slope Calculation: Calculating or interpreting the slope incorrectly.
- Algebraic Errors: Making mistakes in the algebraic manipulation, like distribution or simplification.
⚠️ Note: Always double-check your calculations and be mindful of sign changes.
Advanced Applications

Point slope form isn't just for basic line equations. Here are some advanced applications:
- Tangents to Curves: Useful in calculus when finding the equation of the tangent line to a curve at a specific point.
- Linear Approximations: In approximation techniques like Newton's method or linearization of non-linear functions.
- Perpendicular/Skew Lines: Determining equations of perpendicular or skew lines in 3D space.
Ultimately, point slope form provides a straightforward way to represent a line's equation when you know a point on the line and its slope. Whether you're learning algebra or applying it in higher mathematics, this form is indispensable. Remember, with practice, converting between different forms of linear equations and understanding their graphical and numerical interpretations becomes second nature.
The practice provided in this worksheet not only reinforces your grasp on point slope form but also prepares you for real-world applications where precision and understanding are key. Keep practicing, refine your methods, and ensure that your calculations are correct. This blog has shown how point slope form can be easily worked with, offering you a toolset that is both flexible and powerful in the world of linear equations.
When should I use point slope form over slope-intercept form?

+
Use point slope form when you know a point on the line and the slope. It’s less intuitive for direct graphing but very useful for calculations involving a specific point.
Can I use point slope form in 3D space?
+
Yes, point slope form can extend to three dimensions for lines in space. You’d use two points to define a line, although the equation becomes more complex.
What if I only know two points, not the slope?

+
Calculate the slope using the two points. Then, you can use any one of the points along with this calculated slope to find the equation using point slope form.