5 Piecewise Function Worksheet Answers Explained

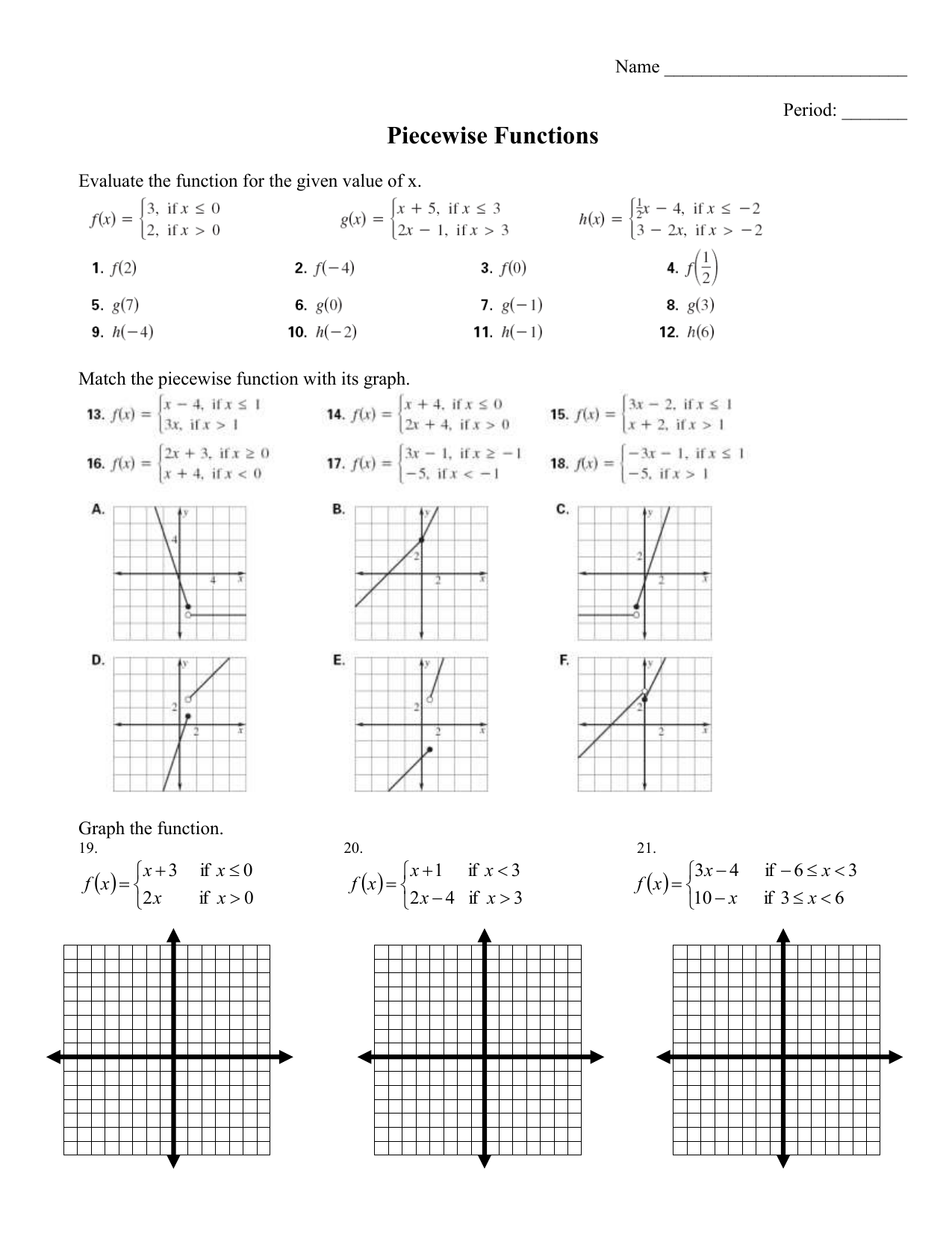
In mathematics, piecewise functions are a way to describe functions that behave differently on different intervals of their domain. These functions are essential for students to understand complex behaviors of mathematical models, from daily life scenarios to engineering applications. This blog post aims to provide detailed explanations for answers on a typical 5 Piecewise Function Worksheet. Here's how we'll approach these functions:

Understanding Piecewise Functions

Piecewise functions are functions that are defined by multiple sub-functions, each applying to a specific interval of the input. Here’s a brief overview of how they work:

• Domain Segmentation: The function’s domain is divided into intervals, and each interval has its own rule (or sub-function).
• Conditional Application: Each sub-function applies only when the input value falls within the given interval.
• Continuity and Discontinuities: At the boundaries of the intervals, piecewise functions can either be continuous or exhibit jumps or breaks.

Sample Problems Explained

Problem 1:

Given the piecewise function:

\[ f(x) = \begin{cases} x^2 + 2 & \text{if } x \leq -1 \\ -2x - 3 & \text{if } -1 < x \leq 3 \\ x + 1 & \text{if } x > 3 \\ \end{cases} \]

Determine the value of f(x) at x = -1, x = 2, and x = 3.5.

📝 Note: Pay attention to the intervals and the respective functions applied at the boundaries.

• At x = -1: Since x ≤ -1, we use the function f(x) = x^2 + 2. So, f(-1) = (-1)^2 + 2 = 3.
• At x = 2: Since -1 < x ≤ 3, we use f(x) = -2x - 3. Thus, f(2) = -2(2) - 3 = -7.
• At x = 3.5: x > 3, so we use f(x) = x + 1. Hence, f(3.5) = 3.5 + 1 = 4.5.

Problem 2:

Consider the function:

\[ g(x) = \begin{cases} \sqrt{4 - x^2} & \text{if } x \leq 0 \\ x^2 + x - 1 & \text{if } 0 < x \leq 2 \\ 4x - 7 & \text{if } x > 2 \\ \end{cases} \]

Find g(0), g(1), and g(2).

• At x = 0: The condition is met for both x ≤ 0 and 0 < x ≤ 2. However, we use the first applicable function since there's no right continuity. So, g(0) = sqrt(4 - 0) = 2.
• At x = 1: Using g(x) = x^2 + x - 1, g(1) = 1^2 + 1 - 1 = 1.
• At x = 2: This is where the interval boundary lies, so we apply g(x) = x^2 + x - 1. g(2) = 2^2 + 2 - 1 = 5.

Problem 3:

Given:

\[ h(x) = \begin{cases} 5 - 2x & \text{if } x < 2 \\ x & \text{if } x \geq 2 \\ \end{cases} \]

Sketch the function and find the value of h(x) at x = 1.5, x = 2, and x = 3.

📝 Note: Observe the continuity of the function at x = 2.

• At x = 1.5: Here, x < 2, so h(1.5) = 5 - 2(1.5) = 2.
• At x = 2: Both functions are applicable, but we take the left-sided limit since x = 2 is on the boundary. So, h(2) = 5 - 2(2) = 1.
• At x = 3: x ≥ 2, so h(3) = 3.

Problem 4:

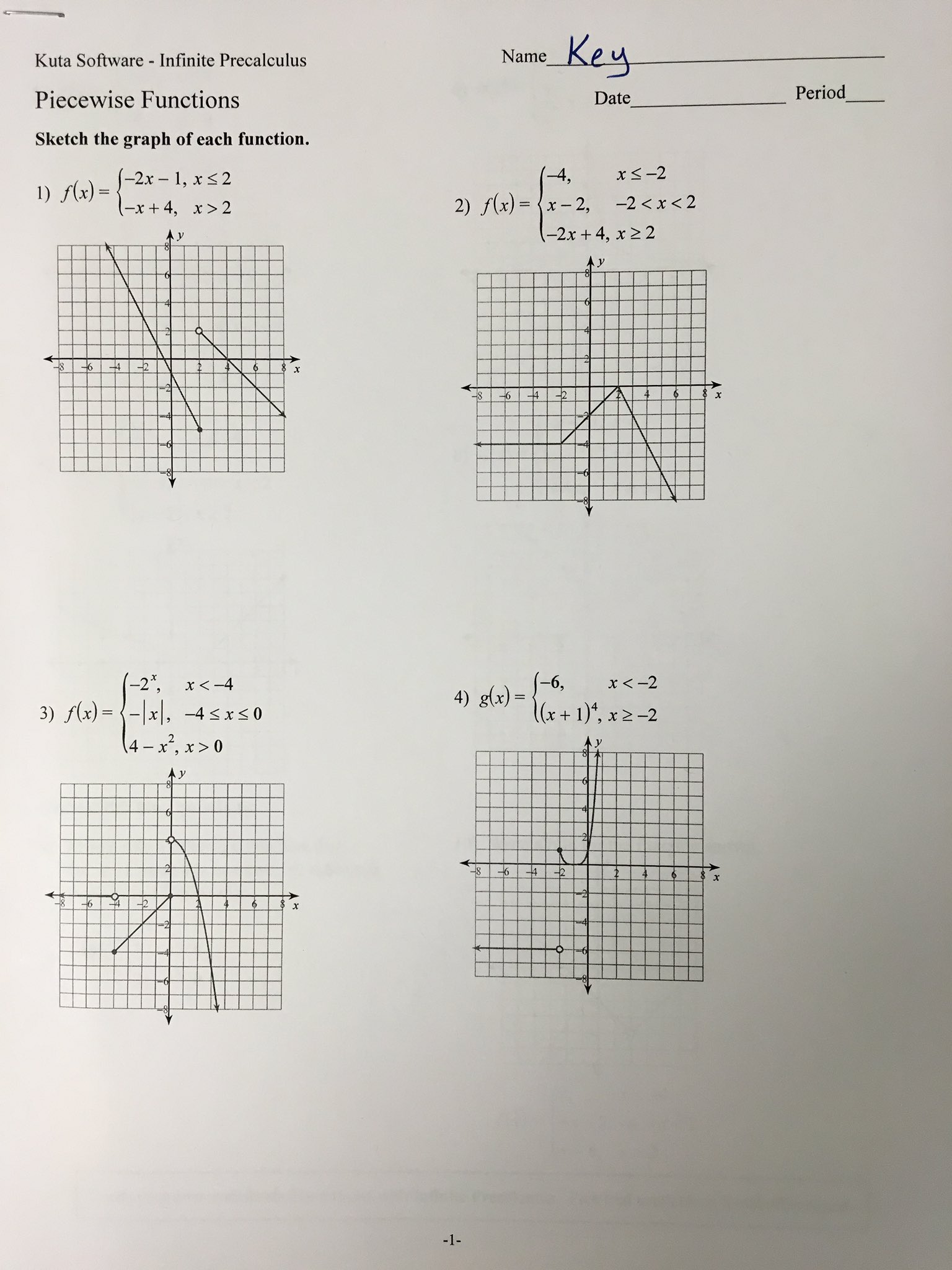
Explore this piecewise function:

\[ k(x) = \begin{cases} x + 1 & \text{if } x \leq -2 \\ \frac{1}{x} & \text{if } -2 < x < 2 \\ x^2 - 3 & \text{if } x \geq 2 \\ \end{cases} \]

Evaluate k(x) for x = -2, x = -1, and x = 2.

• At x = -2: Here, x ≤ -2, so k(-2) = -2 + 1 = -1.
• At x = -1: Since -2 < x < 2, k(-1) = 1/-1 = -1.
• At x = 2: This is on the boundary, so we use k(x) = x^2 - 3, resulting in k(2) = 4 - 3 = 1.

Problem 5:

Analyze the following:

\[ f(x) = \begin{cases} x^2 - 3x & \text{if } x < 1 \\ |4 - x| & \text{if } 1 \leq x < 4 \\ x - 1 & \text{if } x \geq 4 \\ \end{cases} \]

Determine the function values at x = 1, x = 3, and x = 4.5.

• At x = 1: Since x < 1 is also true, but by convention, we use the upper bound if inclusive, so f(1) = |4 - 1| = 3.
• At x = 3: This falls in the second interval, so f(3) = |4 - 3| = 1.
• At x = 4.5: x ≥ 4, thus f(4.5) = 4.5 - 1 = 3.5.

In this extensive exploration of piecewise functions, we've covered how to evaluate functions at specific points, understand domain segmentation, and recognize when continuity or discontinuities occur. These problems illustrate how piecewise functions can model different behaviors in various mathematical and real-world contexts.