5 Must-Know Tips for Mastering Piecewise Functions
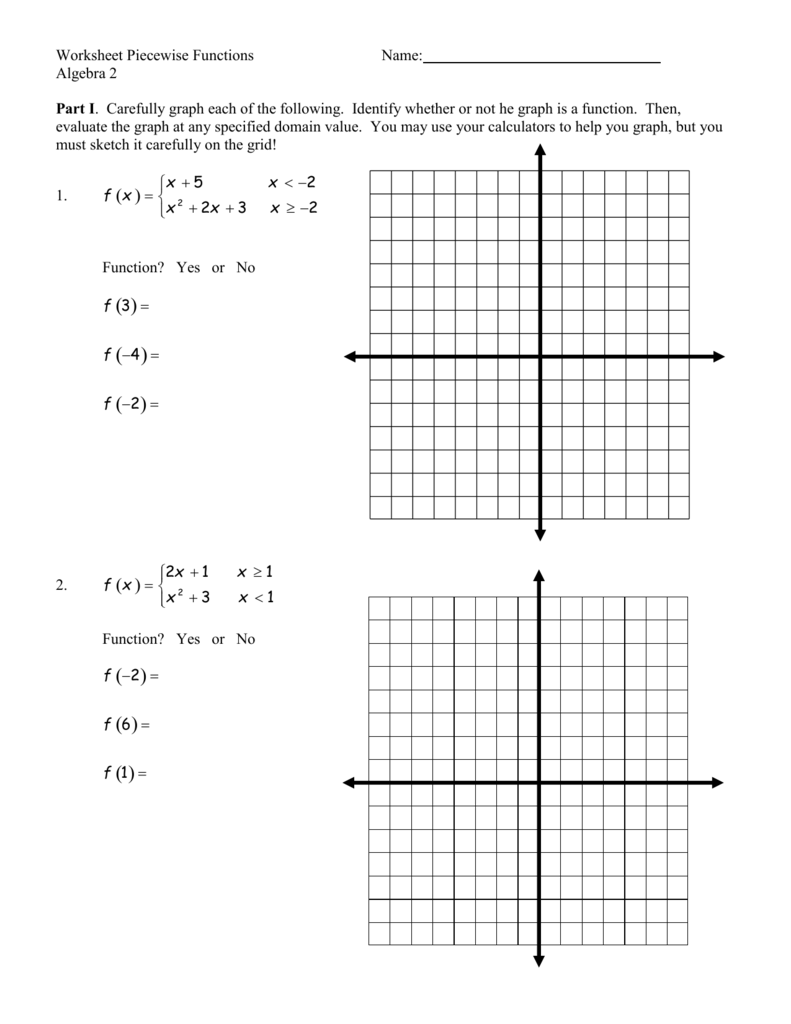
Piecewise functions can appear daunting at first glance. Their segmented nature challenges our typical mathematical intuition, offering unique hurdles in calculus and algebra. However, mastering piecewise functions is essential for students and professionals alike, as they provide a powerful tool for modeling real-world phenomena with distinct behavioral changes. Let's delve into five critical tips that will guide you towards fluency in understanding and utilizing piecewise functions.
1. Understanding the Definition and Domain

Before diving into calculations, ensure a clear grasp of what piecewise functions represent. They are functions composed of several sub-functions, each defined over a specific interval of the domain. Here’s how you can achieve this:
- Identify the Domains: Each segment of a piecewise function has its domain. For example, f(x) = { x, if x ≤ 0 ; x2, if x > 0 }, which means x takes on different values depending on whether it is less than or equal to zero or greater than zero.
- Understand Discontinuities: Recognize where the function is continuous and where there might be jumps or removable discontinuities.
💡 Note: Each sub-function should be consistent within its domain to make the piecewise function meaningful. Any contradictions or overlapping definitions need careful attention.
2. Visualizing Piecewise Functions

Graphing these functions provides a visual representation that can clarify their behavior. Here’s how you can proceed:
- Plot Each Piece: Graph each sub-function separately over its respective interval. For the example above, plot the line y = x for x ≤ 0 and the parabola y = x2 for x > 0.
- Identify Key Points: Points of intersection between different segments, also known as breakpoints or transition points, need careful plotting.
📝 Note: When plotting piecewise functions, use different colors or linestyles to distinguish between segments for clarity.
3. Evaluating Piecewise Functions
To evaluate a piecewise function at a specific value of x, follow these steps:
- Determine the Domain: Find the interval where the given x value falls.
- Apply the Correct Formula: Once the domain is known, use the corresponding function to evaluate x.
🔎 Note: Special attention must be paid at the boundary points where the domain changes to avoid confusion or mistakes.
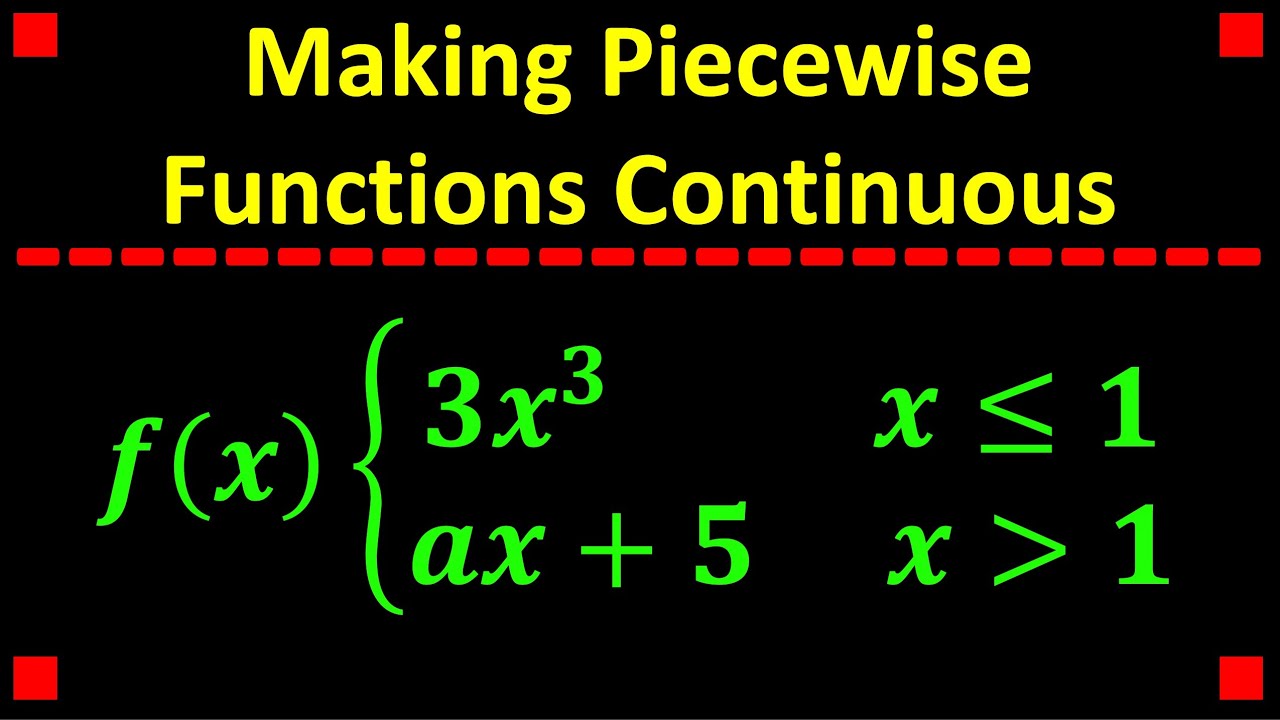
4. Piecewise Derivatives

When taking derivatives of piecewise functions:
- Derive Each Segment: Apply derivative rules to each segment separately.
- Check Continuity: Ensure that the derivative function is continuous at the breakpoints. If not, you might need to address the discontinuities.
- Explore Right and Left Hand Derivatives: At transition points, calculating left-hand and right-hand derivatives can help identify the behavior of the function at those points.
💡 Note: When the function's derivative has a jump at a point, this indicates a cusp or vertex in the original function.
5. Applications and Examples

Piecewise functions are not merely academic constructs; they’re prevalent in real-world applications:
| Application | Example |
|---|---|
| Tax Systems | Different tax rates based on income brackets. |
| Electronic Circuit Switching | Signals may behave differently in different voltage ranges. |
| Graphs in Finance | Options pricing where the payoff function changes at specific price points. |

🔎 Note: Real-world applications often require careful modeling to ensure that the function accurately captures the behavior of the system or process being modeled.
By exploring these tips, one not only becomes adept at handling piecewise functions but also gains insights into their practical relevance. Understanding piecewise functions equips you with the ability to model complex systems, predict behaviors, and analyze real-world data where conditions or states change based on thresholds. These functions are not just a part of theoretical math; they reflect the segmented, conditional nature of many phenomena in our lives, from economics to physics, from signal processing to social sciences.
What makes piecewise functions useful?

+
Piecewise functions allow modeling different behaviors in different domains, which is particularly useful in applications where conditions change based on thresholds or boundaries.
How do I know if my piecewise function is continuous?

+
A piecewise function is continuous if, at each transition point (where the function changes), the left-hand and right-hand limits approach the same value as the function value at that point.
Can piecewise functions have more than two segments?

+
Yes, piecewise functions can be made up of as many segments as needed to accurately model the behavior or data. Each segment can have a different function form or be defined over different intervals.