Perpendicular and Parallel Lines: Worksheet Fun for Geometry Fans
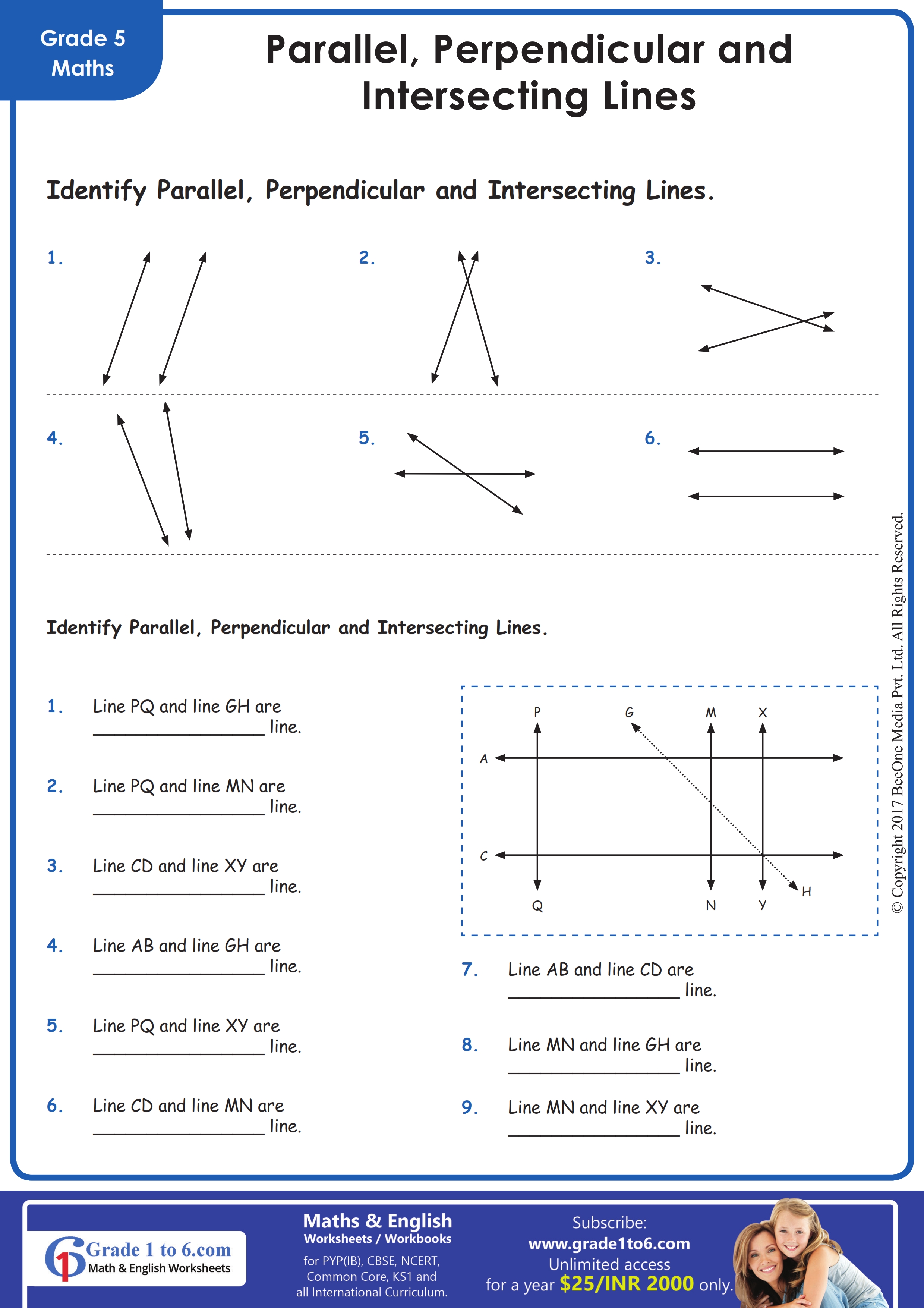
In the dynamic world of geometry, understanding the concepts of perpendicular and parallel lines is fundamental for students. These concepts are not just abstract theories but have real-world applications from architecture to engineering. This blog post dives into an engaging way to learn about these lines through various geometry worksheets.
Understanding Perpendicular Lines


Perpendicular lines are lines that intersect at a right angle (90 degrees). Here’s how you can engage with this concept:
- Draw the Lines: Students can use graph paper to physically draw two lines intersecting at a 90-degree angle.
- Worksheet Activity: Include exercises where students have to determine if given pairs of lines are perpendicular by checking their angle.
Exploring Parallel Lines


Parallel lines are lines on the same plane that never intersect. Here are some fun ways to explore them:
- Railway Tracks Visualization: Ask students to imagine or draw railway tracks, which are perfect examples of parallel lines extending to infinity.
- Worksheet Tasks: Provide worksheets with lines and let students identify parallel lines. Use arrows or distances to clarify.
Combining Perpendicular and Parallel Concepts

Both perpendicular and parallel lines can coexist in interesting ways:
| Type of Lines | Description |
|---|---|
| Perpendicular | Lines that meet at a 90-degree angle |
| Parallel | Lines that do not meet at any point |

Here’s how you can integrate these concepts in learning:
- Grid Navigation: Use a grid to navigate where students move perpendicular to one axis and parallel to another to solve mazes or puzzles.
- Designing Shapes: Engage students in creating shapes where they have to incorporate both parallel and perpendicular lines.
📝 Note: Be mindful of maintaining proper formatting to ensure that the worksheets are both educational and fun.
As you venture further into these geometric concepts, practical applications become evident:
- Architecture: Understanding parallel lines is crucial for designing skyscrapers or even laying out building plans.
- Art and Design: Artists use the concept of perpendicularity to create optical illusions and perspective in their work.
Advanced Worksheets and Challenges

For students ready to take their understanding to the next level:
- Proving Perpendicularity: Introduce worksheets where students prove lines are perpendicular using slope or angle properties.
- Parallel Universe: Create scenarios where students must design a virtual world with parallel streets or pathways.
⚠️ Note: Advanced challenges require a solid foundation in basic geometry concepts, so ensure students have grasped the fundamentals first.
Worksheets that incorporate both visual and analytical elements can significantly enhance understanding:
- Visual Recognition: Exercises that ask students to identify and color perpendicular or parallel lines in complex diagrams.
- Problem Solving: Include problems where students must deduce whether lines are parallel, perpendicular, or neither based on given information.
Final Thoughts

Geometry isn't just about lines and angles; it's about spatial reasoning, problem-solving, and understanding the world around us. Using worksheets to explore perpendicular and parallel lines provides an interactive and effective learning experience. By engaging with these concepts through fun activities, students not only remember the material better but also apply these ideas in various fields and daily life scenarios. Through structured exercises, creative challenges, and real-world applications, students gain a comprehensive understanding that goes beyond the classroom.
Why are perpendicular and parallel lines important?

+
Understanding these concepts is crucial for interpreting blueprints, designing structures, and solving various mathematical problems in physics and engineering.
How can I teach my students to recognize these lines?

+
Use visual aids, practical activities like drawing, and incorporate puzzles that require navigating a grid using perpendicular and parallel movements.
What are some real-world applications of these concepts?

+
From designing roads and buildings to art and graphic design, these lines form the basis of structure and perspective in numerous fields.