Permutations and Combinations Worksheet Answers

Permutations and Combinations: Understanding the Basics
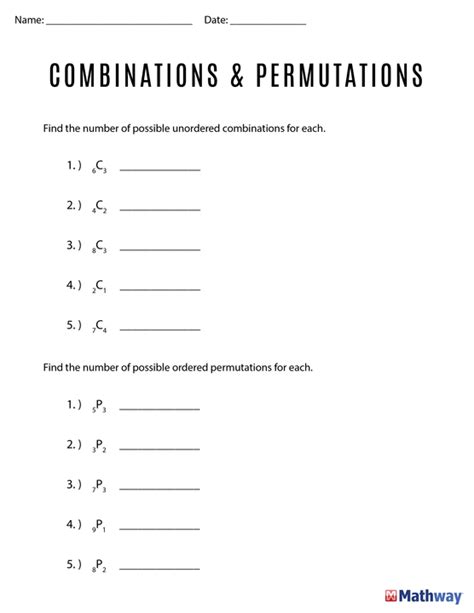
In mathematics, permutations and combinations are two fundamental concepts used to calculate the number of ways to arrange or select objects from a set. These concepts have numerous applications in various fields, including statistics, probability, and computer science. In this article, we will delve into the world of permutations and combinations, exploring their definitions, formulas, and examples.
Permutations: Definition and Formula
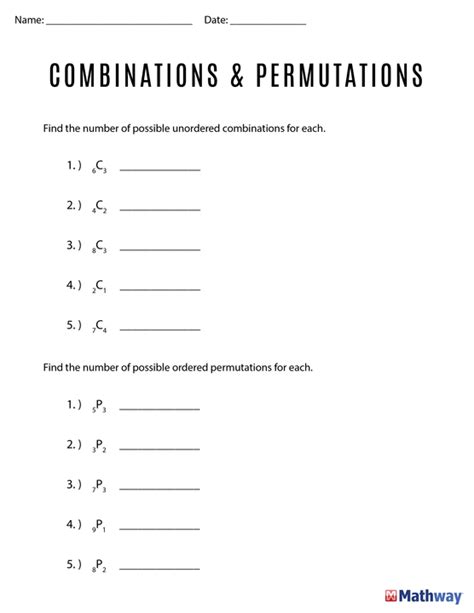
A permutation is an arrangement of objects in a specific order. For example, if we have three letters: A, B, and C, we can arrange them in different ways, such as ABC, ACB, BAC, BCA, CAB, and CBA. The number of permutations of n objects taken r at a time is calculated using the formula:
P(n, r) = n! / (n-r)!
where n! represents the factorial of n (the product of all positive integers up to n).
📝 Note: The factorial of a number is denoted by an exclamation mark (!). For example, 5! = 5 × 4 × 3 × 2 × 1 = 120.
Combinations: Definition and Formula

A combination is a selection of objects from a set, without regard to order. Using the same example as above, if we want to choose two letters from the set {A, B, C}, we can select either AB, AC, or BC. The number of combinations of n objects taken r at a time is calculated using the formula:
C(n, r) = n! / (r!(n-r)!)
Permutations and Combinations: Examples and Solutions

Example 1: Calculate the number of permutations of 5 objects taken 3 at a time.
Solution: P(5, 3) = 5! / (5-3)! = 5! / 2! = (5 × 4 × 3 × 2 × 1) / (2 × 1) = 60
Example 2: Calculate the number of combinations of 7 objects taken 4 at a time.
Solution: C(7, 4) = 7! / (4!(7-4)!) = 7! / (4!3!) = (7 × 6 × 5 × 4 × 3 × 2 × 1) / ((4 × 3 × 2 × 1)(3 × 2 × 1)) = 35
Example 3: A committee of 3 members is to be formed from a group of 8 people. How many different committees can be formed?
Solution: C(8, 3) = 8! / (3!(8-3)!) = 8! / (3!5!) = (8 × 7 × 6 × 5 × 4 × 3 × 2 × 1) / ((3 × 2 × 1)(5 × 4 × 3 × 2 × 1)) = 56
Permutations and Combinations Worksheet Answers

| Question | Solution |
|---|---|
| 1. Calculate P(4, 2) | 12 |
| 2. Calculate C(6, 3) | 20 |
| 3. A set of 10 books is to be arranged on a shelf. How many different arrangements are possible? | 10! = 3628800 |
| 4. A group of 12 students is to be divided into teams of 4. How many different teams can be formed? | C(12, 4) = 495 |
| 5. Calculate P(7, 5) | 2520 |
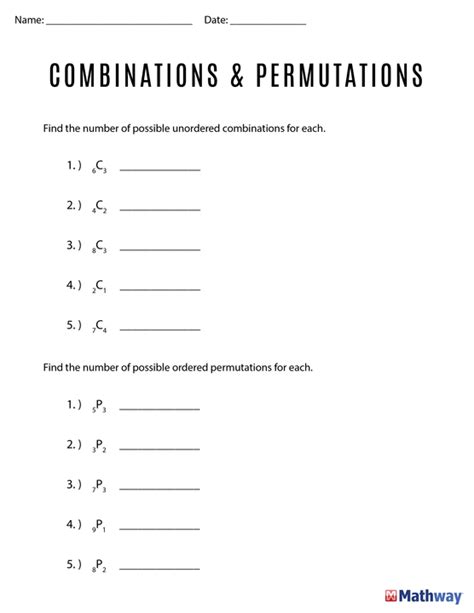
In conclusion, permutations and combinations are fundamental concepts in mathematics that have numerous applications in various fields. By understanding the definitions and formulas, we can calculate the number of ways to arrange or select objects from a set. With practice and persistence, you can master these concepts and become proficient in solving permutation and combination problems.
What is the difference between a permutation and a combination?

+
A permutation is an arrangement of objects in a specific order, while a combination is a selection of objects from a set, without regard to order.
How do I calculate the number of permutations of n objects taken r at a time?

+
The number of permutations of n objects taken r at a time is calculated using the formula P(n, r) = n! / (n-r)!.
How do I calculate the number of combinations of n objects taken r at a time?

+
The number of combinations of n objects taken r at a time is calculated using the formula C(n, r) = n! / (r!(n-r)!).
Related Terms:
- Permutations and Combinations Worksheet pdf
- Permutation Worksheet with Answers pdf
- Simple permutations and combinations Worksheet
- permutation vs combination worksheet answers
- combination worksheet with answers pdf
- combinations questions and answers pdf



