5 Math Tips: Permutation and Combination Worksheet Mastery

Are you trying to conquer the complexities of permutations and combinations in mathematics? Often, these mathematical concepts can seem daunting, but with the right approach, you can not only understand but master them. This guide will walk you through effective methods to improve your skills in solving permutation and combination problems, ensuring you feel confident in tackling any worksheet that comes your way.
The Fundamentals of Permutation and Combination


Before diving into the tips for mastering permutation and combination worksheets, it's vital to understand their basics:
- Permutation: This refers to the arrangement of items where the order is crucial. For example, arranging letters in a word or people in a line.
- Combination: Here, the order does not matter, focusing instead on the selection. For instance, choosing a team or a committee.
Tip 1: Understand the Notation

Permutations and combinations have specific notations:
- nPr stands for the number of permutations of n items taken r at a time.
- nCr represents the number of combinations of n items taken r at a time.
📝 Note: Remember, these notations are not just shorthand; they represent different mathematical operations that determine how you solve problems.
Tip 2: Master the Formulas
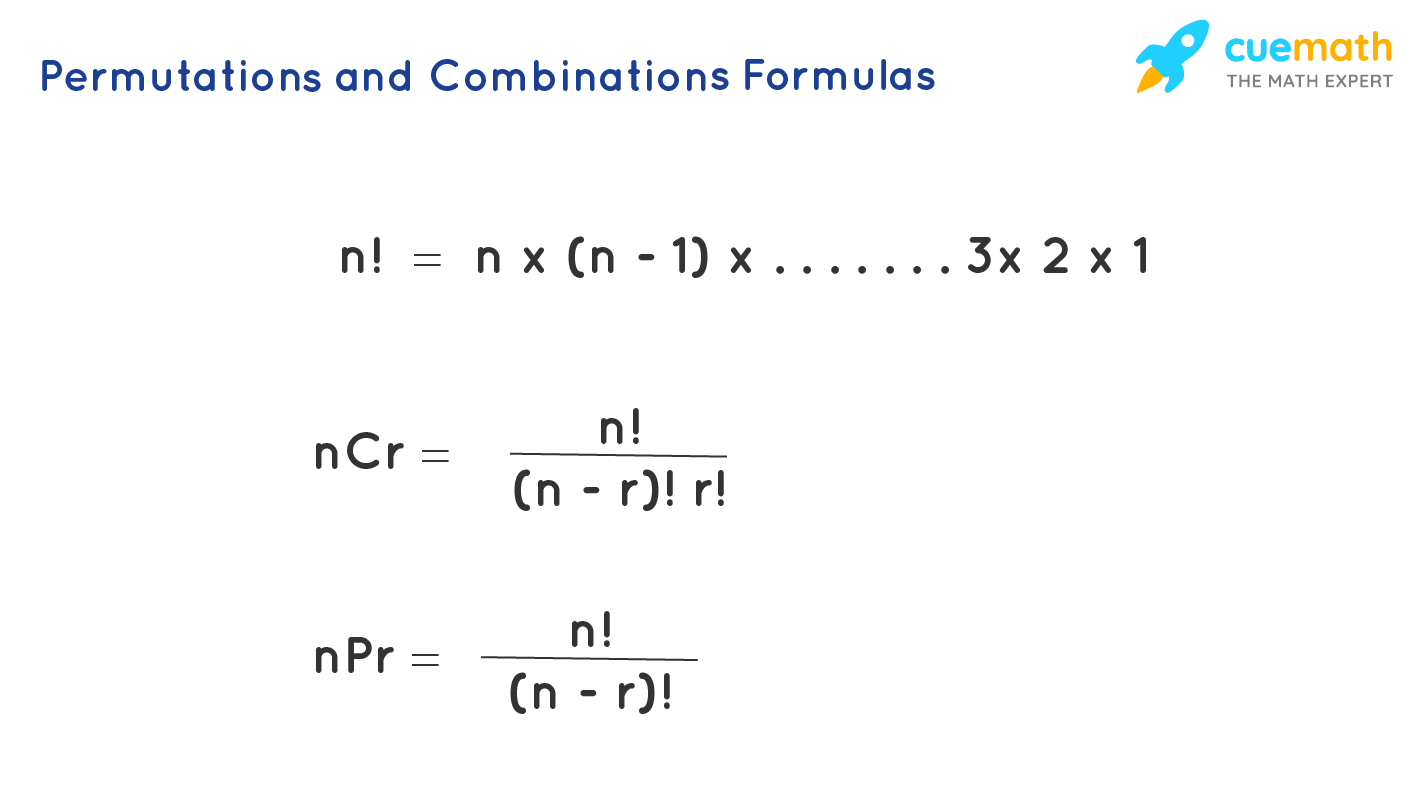
| Formula | Explanation |
|---|---|
| nPr = n!/(n-r)! | Arrangement where order matters |
| nCr = n!/(r!(n-r)!) | Selection where order is not relevant |

Memorizing these formulas isn’t enough; you need to understand their derivation and when to apply them.
Tip 3: Practice with Real-life Examples

Linking mathematical concepts to real-life scenarios can make them more relatable:
- How many ways can you arrange 5 different books on a shelf? (Permutation)
- How many different three-person committees can you form from a group of 8? (Combination)
Tip 4: Use Mnemonics

To help remember the difference between permutations and combinations, think of mnemonics:
- Permutation: Position Matters
- Combination: Can Miss Order
Simple phrases like these can stick in your memory, aiding in quick decision-making during problem-solving.
Tip 5: Work Backwards

Sometimes, it’s beneficial to solve a problem in reverse:
- If you know the answer, find how you could have gotten there by applying permutation or combination rules.
This reverse engineering approach can deepen your understanding and help when you’re stuck.
In summary, mastering permutations and combinations involves understanding their fundamental differences, mastering the relevant formulas, practicing with real-life applications, using mnemonics, and occasionally working problems in reverse. By following these tips, you'll find that what once seemed like a complex mathematical puzzle becomes a manageable and even enjoyable part of your mathematical journey.
What’s the difference between permutation and combination?

+
Permutation deals with arrangements where order matters, while combination involves selections where order does not matter.
How do I know when to use nPr or nCr?

+
Use nPr if the problem involves arrangements or when the order of selection matters. Use nCr if it’s about selecting a subset without regard to order.
Can permutations and combinations overlap in some problems?

+
Yes, some complex problems might require using both concepts, especially in real-world applications where partial order might be significant.