Master Perfect Square Trinomials with Our Worksheet

Learning mathematics can be both challenging and incredibly rewarding, especially when exploring concepts like perfect square trinomials. Perfect square trinomials are algebraic expressions that can be written as the square of a binomial. They hold a pivotal place in simplifying algebraic expressions, factoring, and solving quadratic equations. This detailed guide will take you through the intricacies of perfect square trinomials, helping you master this algebraic concept using our comprehensive Perfect Square Trinomials Worksheet.
The Essence of Perfect Square Trinomials
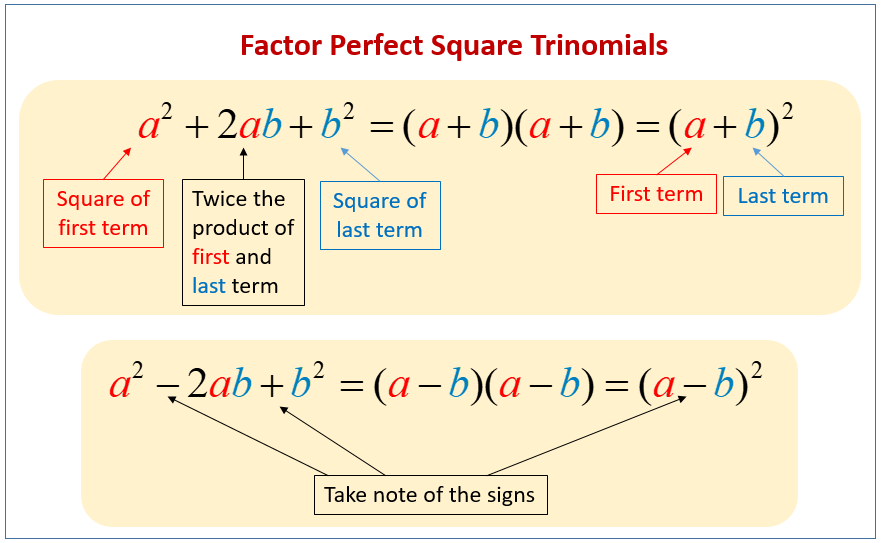
A perfect square trinomial is essentially an expression of the form:
- a² + 2ab + b² = (a + b)²
- a² - 2ab + b² = (a - b)²
Here, a and b are any real numbers. To verify if an expression is indeed a perfect square trinomial, you can observe:
- It's a trinomial (three terms).
- The first and last terms are perfect squares.
- The middle term is double the product of the square roots of the first and last terms.
Unraveling the Mystery with Our Worksheet

Our Perfect Square Trinomials Worksheet is designed to guide you through this algebraic concept with step-by-step exercises:
- Identify Perfect Square Trinomials: The first set of exercises will help you recognize which expressions are perfect square trinomials.
- Factoring Perfect Square Trinomials: You'll learn to factorize perfect square trinomials into the product of two binomials.
- Completing the Square: This section teaches you to transform any quadratic expression into a perfect square trinomial, which is essential for solving quadratic equations.
- Solving Equations: Finally, you'll apply what you've learned to solve equations where one side is a perfect square trinomial.
Step-by-Step Process

1. Identifying Perfect Square Trinomials
To identify a perfect square trinomial, you can:
- Check if the first and last terms are perfect squares.
- Verify if the middle term is twice the product of the square roots of these terms.
2. Factoring Perfect Square Trinomials
The process involves:
- Taking the square root of the first term for the first binomial.
- Taking the square root of the last term for the second binomial.
- Determining if the middle sign is positive or negative to decide between addition or subtraction in the binomial.
Here's an example:
x² + 6x + 9 = (x + 3)²
📝 Note: Remember, the middle term's sign is crucial when factoring. Positive middle terms indicate both binomial terms are added, while negative terms indicate subtraction.
3. Completing the Square
This technique transforms a quadratic expression into a perfect square trinomial. Here are the steps:
- Ensure the coefficient of the quadratic term is 1 (if not, divide the whole equation by that coefficient).
- Take the coefficient of the middle term, divide it by 2, and square it. Add and subtract this value inside the equation.
- Group the perfect square trinomial and the constant term that was just subtracted.
An example:
x² + 10x + 12 = (x + 5)² - 13
4. Solving Equations
Once you've transformed an equation into a perfect square trinomial format, you can:
- Take the square root of both sides to find the solutions for x.
🧠 Note: Remember to consider both the positive and negative roots when solving for x.
The final stages of this journey through our worksheet allow you to practice applying these concepts to solve complex quadratic equations, which is both a practical skill and a demonstration of mastery over the subject.
Conclusion

Mastering perfect square trinomials significantly enhances your ability to work with algebraic expressions, factor, and solve quadratic equations. This guide and our Perfect Square Trinomials Worksheet offer a structured path to understanding and applying this concept effectively. By working through the exercises, you'll gain not only in-depth knowledge but also the confidence to tackle more complex mathematical problems. Remember, practice is key to mastery; use our worksheet to refine your skills, and watch as your proficiency in algebra grows.
How can I determine if an expression is a perfect square trinomial?

+
Check if the first and last terms are perfect squares and if the middle term is twice the product of their square roots.
What are some common errors to avoid when factoring perfect square trinomials?

+
Be careful with the signs in the binomials and ensure you’re taking the correct square roots. Also, avoid mixing up the order of terms when adding or subtracting in the binomials.
How do I solve a quadratic equation after completing the square?

+
Take the square root of both sides, then solve for the variable, keeping in mind to consider both the positive and negative roots.



