5 Essential Percent Problems Solved: Free Worksheet Answers

Welcome to our comprehensive guide on essential percent problems. Whether you're a student looking to master percentage calculations or an adult revisiting math for financial planning, understanding how to work with percentages is incredibly beneficial. In this article, we'll explore five key percentage problems, providing step-by-step solutions along with a free worksheet where you can practice these concepts.
Understanding Percentages

Percentages, denoted by the symbol ‘%’, represent parts per hundred. They are useful in various contexts:
- To compute discounts during sales
- To understand growth rates or interest
- To calculate taxes, tips, or commissions
A good grip on percentages can greatly enhance your ability to make informed decisions in both personal and professional scenarios.
Problem 1: Finding a Percentage of a Number

Let’s start with a basic problem: how do you find 25% of 80?
- Step 1: Convert the percentage to a decimal by dividing by 100. So, 25% = 0.25.
- Step 2: Multiply the decimal by the given number. Here, 0.25 x 80 = 20.
Therefore, 25% of 80 is 20.
Free Worksheet: Finding Percentages

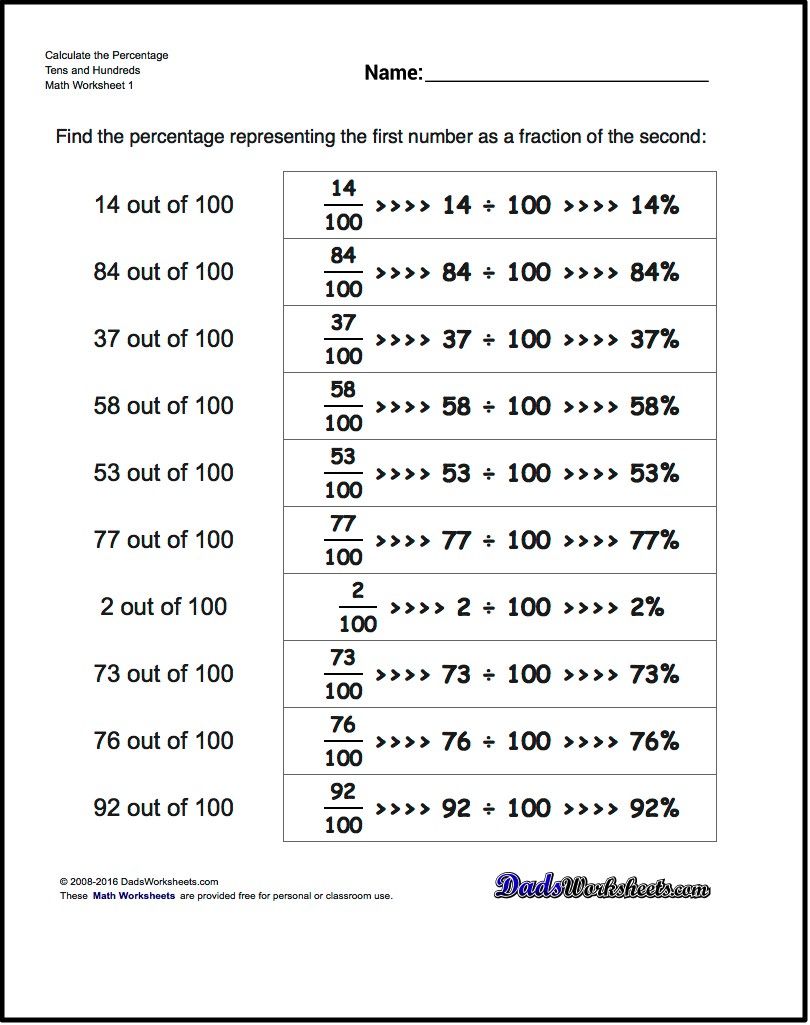
Here’s a simple table with examples to practice finding percentages:
| Percentage | Number | Result |
|---|---|---|
| 10% | 50 | 5 |
| 40% | 150 | 60 |
| 75% | 120 | 90 |

Problem 2: Finding the Whole from a Percentage

Now, let’s reverse the process: If 30 is 60% of a number, what is that number?
- Step 1: Set up the equation. Let x be the unknown whole. 30 = 0.6 * x.
- Step 2: Solve for x by dividing both sides by the decimal value of the percentage: x = 30 / 0.6 = 50.
Hence, 30 is 60% of 50.
Problem 3: Percent Change

Calculating percent change is often used to track changes in price, population, or other metrics over time:
- Step 1: Determine the difference between the new and old values. Let’s say the price went from 100 to 120, so the difference is $20.
- Step 2: Divide this difference by the old value and multiply by 100 to get the percentage. Here, it would be (20 / 100) * 100 = 20%.
The price has increased by 20%.
Problem 4: Percentage Increase and Decrease

Understanding how to increase or decrease a quantity by a percentage is crucial in sales, budgeting, or any scenario involving financial adjustments:
- For a percentage increase: Multiply the original value by (1 + the percentage as a decimal). If a shirt costs 50 and you want to increase its price by 15%, the new price would be <strong>50 * (1 + 0.15) = 57.50.
- For a percentage decrease: Multiply the original value by (1 - the percentage as a decimal). If a 120 item is discounted by 20%, the reduced price is <strong>120 * (1 - 0.20) = 96.
Problem 5: Compound Interest

Compound interest, where interest is added to the principal sum, can significantly increase the amount of money over time:
- Use the compound interest formula: A = P(1 + r/n)^(n*t) where:
- A is the amount of money accumulated after n years, including interest.
- P is the principal amount (the initial amount of money).
- r is the annual interest rate (decimal).
- n is the number of times interest is compounded per year.
- t is the time the money is invested or borrowed for, in years.
- If you invest 1,000 at an annual rate of 5%, compounded quarterly (n=4) for 2 years, the calculation would be:</li> <ul> <li><strong>A = 1000(1 + 0.05/4)^(4*2) = 1103.81
After 2 years, the investment would grow to $1103.81.
💡 Note: Compound interest can make a significant difference in the long term, especially when reinvesting dividends or interest income.
In this extensive guide, we've covered the spectrum of percent problems you might encounter. From finding simple percentages to understanding compound interest, these calculations are indispensable in various aspects of life. Keep practicing with the worksheet provided, and you'll be well-equipped to tackle any percentage calculation with confidence. Understanding these principles not only helps in academic settings but also in making savvy financial decisions. Whether it's calculating a discount, understanding growth rates, or managing investments, percentages are at the core of much of our daily decision-making process.
What is the easiest way to find percentages?

+
The easiest method often involves converting percentages to decimals and then performing multiplication. For instance, to find 20% of 150, convert 20% to 0.20, then multiply: 150 * 0.20 = 30.
Can percentages be more than 100%?
+
Yes, percentages can exceed 100%. This is often seen when dealing with growth rates or comparing quantities where the value increases beyond the original amount. For example, if a population doubles, it’s 200% of its initial size.
How does compound interest differ from simple interest?

+
Simple interest is calculated only on the initial amount (principal) invested. Compound interest, on the other hand, is calculated on the principal plus any accumulated interest. This means that over time, compound interest grows faster as interest is added to interest.
How can knowing percentages help in daily life?

+
Percentages are useful in various everyday scenarios like budgeting, calculating discounts during shopping, understanding nutritional facts on food packaging, and determining investment growth or savings.