Percent Error Worksheet Answers: Boost Your Skills Easily

Understanding and calculating percent error is a fundamental skill in many scientific and analytical fields. Whether you're a student, professional, or just someone curious about how things work, mastering this concept can help you evaluate the precision and accuracy of your experiments or calculations. This blog post will guide you through the process of finding percent error, provide worksheets to practice, and give you detailed solutions to enhance your understanding and improve your skills with ease.
What is Percent Error?

Percent error measures how far off your experimental value is from the actual or accepted value, expressed as a percentage. Here’s the formula:
Percent Error = ((|Experimental Value - Accepted Value|) / Accepted Value) * 100%
Here, the vertical bars denote absolute value, ensuring the result is positive since error can’t be negative in this context.
How to Calculate Percent Error

To calculate percent error, follow these steps:
- Identify the Accepted Value: This is the known or true value you should compare against.
- Identify the Experimental Value: This is your measurement or result from the experiment.
- Calculate the Difference: Subtract the accepted value from the experimental value and take the absolute value of the result.
- Divide the Difference: Divide this absolute difference by the accepted value.
- Multiply by 100: To convert the fraction to a percentage.
Let’s illustrate with an example:
- Accepted Value = 50 meters
- Experimental Value = 55 meters
Percent Error = (|55 - 50| / 50) * 100 = (5 / 50) * 100 = 10%
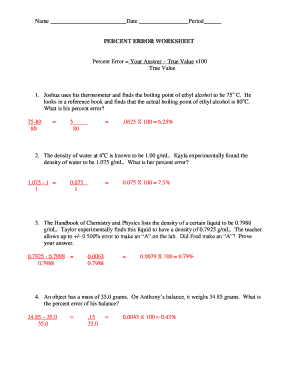
Practice with Percent Error Worksheet

To reinforce your understanding, here is a worksheet to help you calculate percent error. Solve these problems, and then check your answers below:
| Problem | Accepted Value | Experimental Value |
|---|---|---|
| 1 | 20 grams | 22 grams |
| 2 | 100 volts | 95 volts |
| 3 | 25 degrees Celsius | 26 degrees Celsius |
| 4 | 60 seconds | 65 seconds |

Percent Error Worksheet Answers

Here are the solutions for the worksheet:
- Problem 1: ((|22 - 20|) / 20) * 100 = 10%
- Problem 2: ((|100 - 95|) / 100) * 100 = 5%
- Problem 3: ((|25 - 26|) / 25) * 100 = 4%
- Problem 4: ((|65 - 60|) / 60) * 100 = 8.33%
🌟 Note: While calculating, ensure you round your percentage to two decimal places for precision, unless otherwise specified.
By working through these examples, you can start to get a feel for how percent errors vary with the values involved. This practice not only helps in mastering the calculation but also in understanding the significance of the results in real-world applications.
Applications of Percent Error

Percent error has numerous applications in various scientific fields:
- Physics: Measuring the accuracy of experiments.
- Chemistry: Determining the precision of lab work or purity of substances.
- Engineering: Evaluating design tolerances and manufacturing processes.
- Economics: Analyzing discrepancies in financial forecasts versus actual results.
Now, as we wind down our discussion on percent error, we can see that this simple calculation serves as a bridge between theoretical expectations and practical outcomes. It is a tool for critical analysis and a step towards improving accuracy and reliability in various disciplines.
Why is calculating percent error important?
+
Percent error helps assess how closely experimental results match theoretical or known values, which is crucial for refining methods, validating hypotheses, and ensuring reliability in scientific and industrial applications.
Can percent error be more than 100%?

+
Yes, percent error can exceed 100% if the experimental value is significantly off from the accepted value. This indicates a large discrepancy, often requiring revisiting the experimental setup or calculation methods.
What does a negative percent error mean?
+
Since percent error uses absolute value, negative errors don't exist in traditional percent error calculations. However, if you're looking at the sign, a negative could indicate an experimental value less than the accepted value.
This comprehensive exploration of percent error provides you with the tools to evaluate your work’s accuracy and enhance your skills in a variety of scientific and technical fields. By practicing with real-world examples and understanding the implications of your calculations, you’ll find yourself better equipped to handle data with precision and confidence.