Master Percent Composition: Molecular Formula Worksheet Guide

In the fascinating field of chemistry, understanding the percent composition of substances is crucial for various analytical processes. The concept of percent composition tells us the percentage by mass of each element within a compound. This metric is not only fundamental in chemical stoichiometry but also plays a pivotal role in fields like pharmacology, where the purity of compounds can affect drug efficacy, and in food science for nutritional labeling. Here's an in-depth guide to mastering the calculation of percent composition using a molecular formula worksheet.
Understanding Percent Composition

Percent composition is determined by calculating the mass percentage of each element in a chemical compound. The formula to calculate this is:
[ \text{Percent Composition of Element X} = \left( \frac{\text{Atomic Mass of X} \times \text{Number of atoms of X}}{\text{Molecular Mass of Compound}} \right) \times 100\% ]
Here, you calculate the molecular mass by adding the atomic masses of all atoms in the molecule.
Steps to Calculate Percent Composition

- Identify Each Element in the Compound: Write down all the elements present in the compound’s molecular formula.
- Determine the Number of Atoms of Each Element: From the molecular formula, count how many atoms of each element are present.
- Find the Atomic Mass for Each Element: Use a periodic table or standard reference to get the atomic mass for each element.
- Calculate the Molecular Mass: Sum the product of the atomic mass and the number of atoms for each element in the compound.
- Calculate Percent Composition: Apply the formula provided above for each element in the compound.
Example Calculation

Let’s calculate the percent composition for glucose (C6H12O6):
- Carbon: There are 6 carbon atoms. Atomic mass of carbon is approximately 12.01 g/mol. Thus, mass of carbon in glucose = 6 x 12.01 = 72.06 g/mol.
- Hydrogen: There are 12 hydrogen atoms. Atomic mass of hydrogen is 1.008 g/mol. Thus, mass of hydrogen in glucose = 12 x 1.008 = 12.096 g/mol.
- Oxygen: There are 6 oxygen atoms. Atomic mass of oxygen is approximately 16.00 g/mol. Thus, mass of oxygen in glucose = 6 x 16.00 = 96.00 g/mol.
- Molecular Mass of Glucose: 72.06 + 12.096 + 96.00 = 180.156 g/mol.
Now, let's compute the percent composition:
- Carbon: \left(\frac{72.06}{180.156}\right) \times 100 = 40.0\%
- Hydrogen: \left(\frac{12.096}{180.156}\right) \times 100 = 6.71\%
- Oxygen: \left(\frac{96.00}{180.156}\right) \times 100 = 53.29\%
Using a Worksheet to Master Calculations
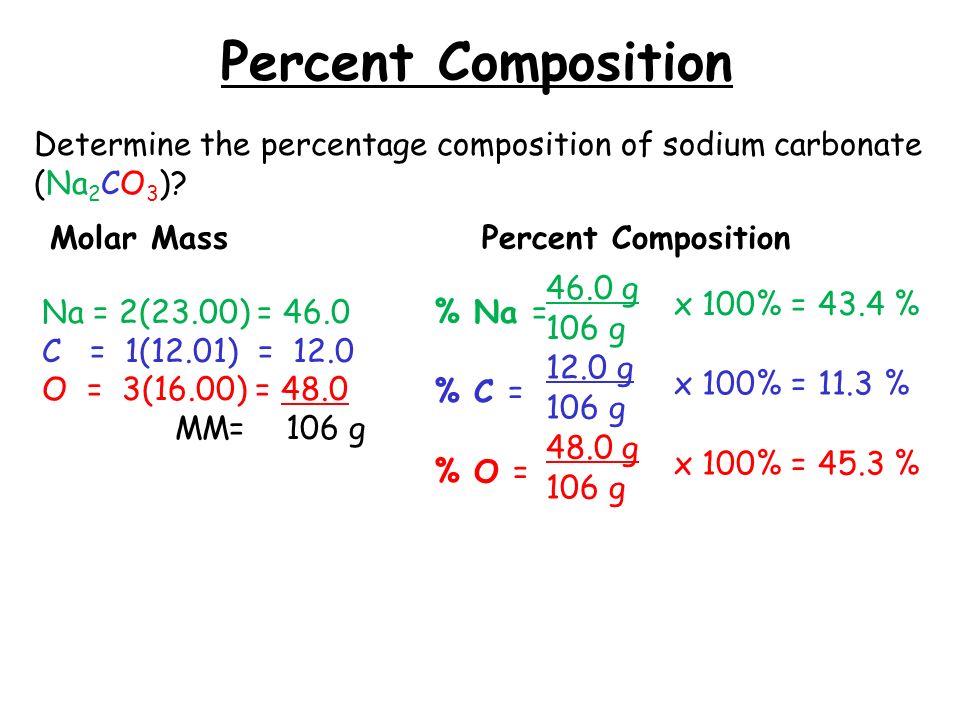
A molecular formula worksheet can serve as an excellent tool for practice:
- List Compounds: Write down several compounds you wish to analyze for percent composition.
- Set Up Your Table: Create a table with columns for Element, Number of Atoms, Atomic Mass, Molecular Mass of Compound, and Percent Composition.
- Calculate: Fill in each row with the calculations for one element at a time. Use the steps listed above to find the percent composition.
| Element | No. of Atoms | Atomic Mass (g/mol) | Molecular Mass of Glucose | Percent Composition |
|---|---|---|---|---|
| Carbon | 6 | 12.01 | 180.156 | 40.0% |
| Hydrogen | 12 | 1.008 | 180.156 | 6.71% |
| Oxygen | 6 | 16.00 | 180.156 | 53.29% |

🔍 Note: Ensure to double-check your calculations for accuracy, especially with units and significant figures to maintain precision.
Why Master Percent Composition?

Mastering percent composition is key to:
- Understanding chemical reactions and stoichiometry.
- Analyzing purity in pharmaceuticals or environmental samples.
- Determining nutritional content in food analysis.
- Forensic analysis where the composition of substances is critical.
Percent composition calculations can provide insight into the structural makeup of compounds, which can be vital for research, quality control, and product development. As you delve deeper into chemistry, these concepts will form the bedrock of more complex analyses and theories.
What is the difference between empirical and molecular formulas?

+
The empirical formula represents the simplest whole-number ratio of atoms in a compound, while the molecular formula shows the actual number of atoms of each element in a molecule. For example, glucose has an empirical formula of CH2O and a molecular formula of C6H12O6.
Can percent composition be used to determine the formula of a compound?

+
Yes, by comparing the percent composition of a compound to the theoretical percent composition calculated from possible molecular formulas, one can deduce or confirm the formula of the compound.
How does percent composition relate to stoichiometry?

+
Percent composition is integral to stoichiometry because it helps determine the amount of reactants and products in a chemical reaction based on the mass of each element present in the compounds involved.