5 PEMDAS Practice Worksheets for Math Mastery

In the realm of mathematics, mastering basic operations and understanding the order in which they should be performed is foundational. One of the key concepts that students often struggle with is PEMDAS - an acronym for Parentheses, Exponents, Multiplication and Division (from left to right), Addition and Subtraction (from left to right). Understanding and applying PEMDAS effectively can dramatically improve a student's ability to solve mathematical problems correctly. In this post, we will explore five PEMDAS practice worksheets designed to enhance students' proficiency with this order of operations.
The Importance of PEMDAS in Mathematics

PEMDAS isn't just a rule; it's a system that ensures consistency in solving mathematical expressions. Here are a few reasons why mastering PEMDAS is crucial:
- Ensures Accuracy: Following PEMDAS helps in obtaining the correct answer consistently, reducing errors.
- Improves Problem-Solving Skills: Understanding the sequence of operations enhances logical thinking and structured problem-solving approaches.
- Prepares for Advanced Math: Knowledge of PEMDAS is fundamental for learning more complex mathematical concepts in algebra, calculus, and beyond.
Worksheet 1: Basics of PEMDAS

The first worksheet focuses on the basics, ensuring students understand how to apply each component of PEMDAS:
| Problem | Solution |
|---|---|
| 3 + (4 * 2) | 3 + 8 = 11 |
| 5 - (8 / 2) + 3 | 5 - 4 + 3 = 4 |
| 2 * (3^2) - 4 | 2 * 9 - 4 = 14 |
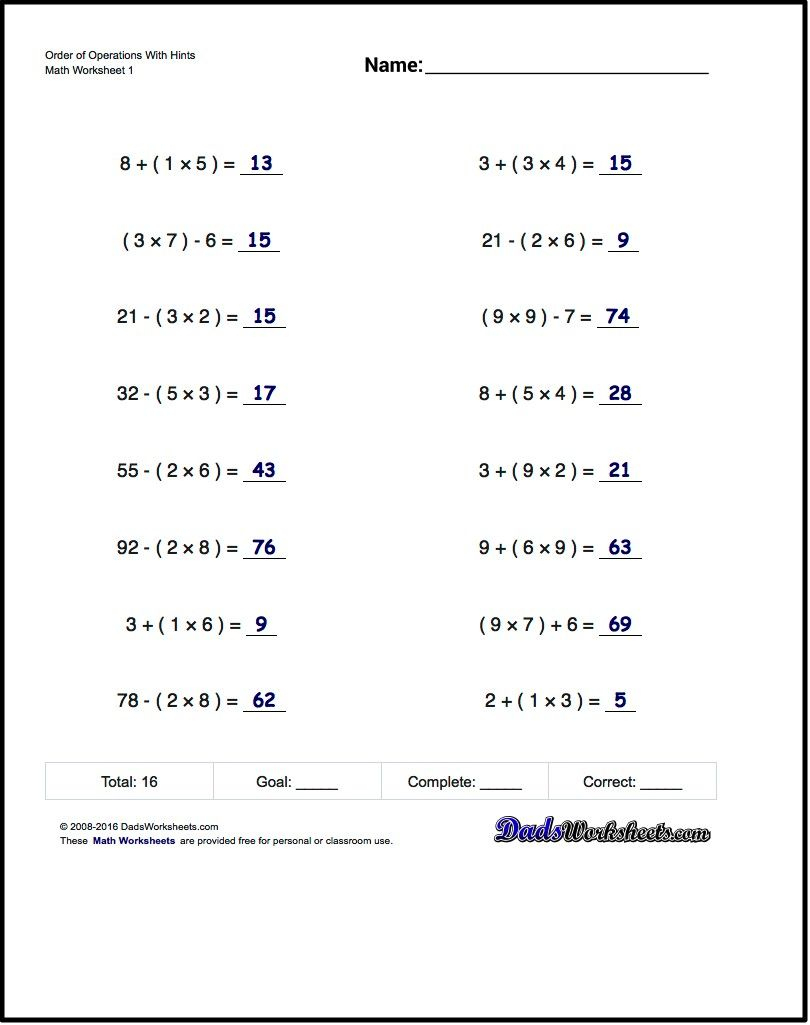
⚠️ Note: This worksheet starts with simple examples to build confidence before moving to more complex problems.
Worksheet 2: Mixed Operations with Parentheses

Here, students will work with multiple operations within parentheses:
- 7 - (2 * 3) + 4 = 7 - 6 + 4 = 5
- (9 + 3) * (7 - 4) = 12 * 3 = 36
- (6 - 3^2) / 3 + 2 = (6 - 9) / 3 + 2 = -1 + 2 = 1
Worksheet 3: Exponents and Order of Operations

Exponents can often be confusing for students. This worksheet isolates them to clarify their precedence:
- 4^2 + 3 * (5 - 1)
- (2 + 3)^2 - 8/2
- 5 * (6 + 4) / (2^3)
Worksheet 4: Multiplication and Division with Parentheses
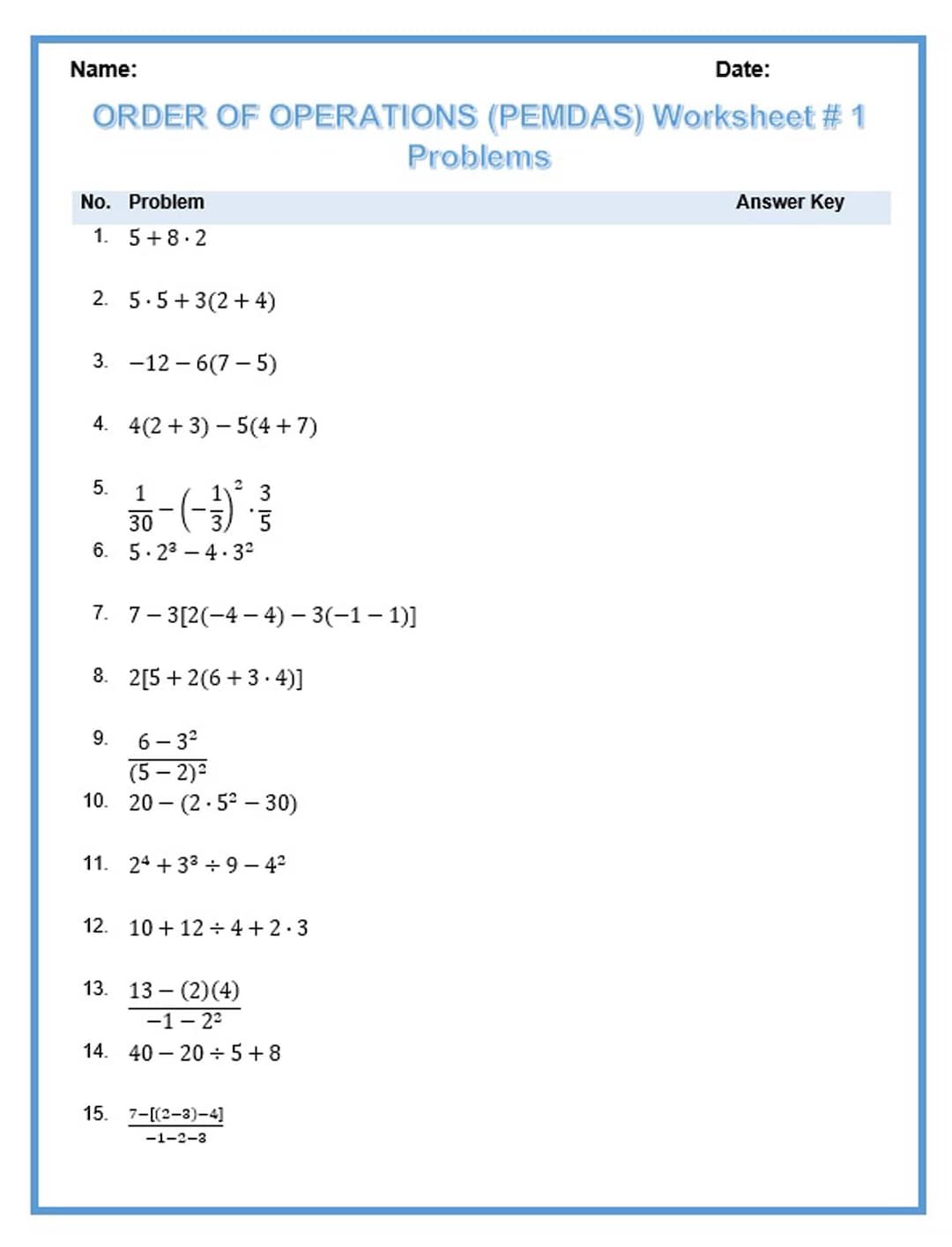
Understanding how to handle division and multiplication within and outside of parentheses:
- (10 + 2) * 6 / 3
- 8 / (4 - 2) * 5
- 24 / (3 * 2) + 6
Worksheet 5: Complex PEMDAS with Fractions

This worksheet introduces fractions to make PEMDAS even more challenging:
| Problem | Solution |
|---|---|
| 2^2 + (3/4 * 8) - 1/2 | 4 + 6 - 0.5 = 9.5 |
| 5 - 1/3 * (2+1) + 1/2 | 5 - 1 + 0.5 = 4.5 |
| (3 + 1/2) / 2 * 4 - 2 | 3.5 / 2 * 4 - 2 = 7 - 2 = 5 |
🌟 Note: These worksheets can be used sequentially or individually based on a student's progress or areas where they need more practice.
Mastering PEMDAS is about understanding the logical flow of mathematical operations. These five worksheets are designed to guide students through the process step by step, from basic to advanced problems, helping to cement their understanding of how and when to apply each operation. With regular practice, students will become more comfortable and quicker in solving mathematical expressions. Moreover, by offering problems with increasing complexity, these worksheets ensure that students are well-prepared for any level of math, from basic arithmetic to more advanced algebraic expressions.
Why is PEMDAS important in mathematics?

+
PEMDAS provides a consistent framework for solving mathematical expressions, ensuring accuracy and clarity in mathematical communication. It’s vital for problem-solving at all levels of mathematics.
Can I skip learning PEMDAS if I’m good at math?

+
Not really. PEMDAS is fundamental for structuring math problems correctly. Even advanced mathematicians use these principles to ensure the validity of their work.
How should I introduce PEMDAS to beginners?

+
Start with simple problems that only require understanding one or two operations at a time, gradually increasing complexity as the student grasps each concept.
What if I still make mistakes using PEMDAS?

+
Practice is key. Use these worksheets regularly, and if errors persist, consider revisiting basic operation rules or seeking additional teaching resources to clarify misunderstandings.
Are there any mnemonic devices or strategies to remember PEMDAS?

+
Yes, for instance, “Please Excuse My Dear Aunt Sally” or using visual aids like trees to represent the order of operations can be helpful.

