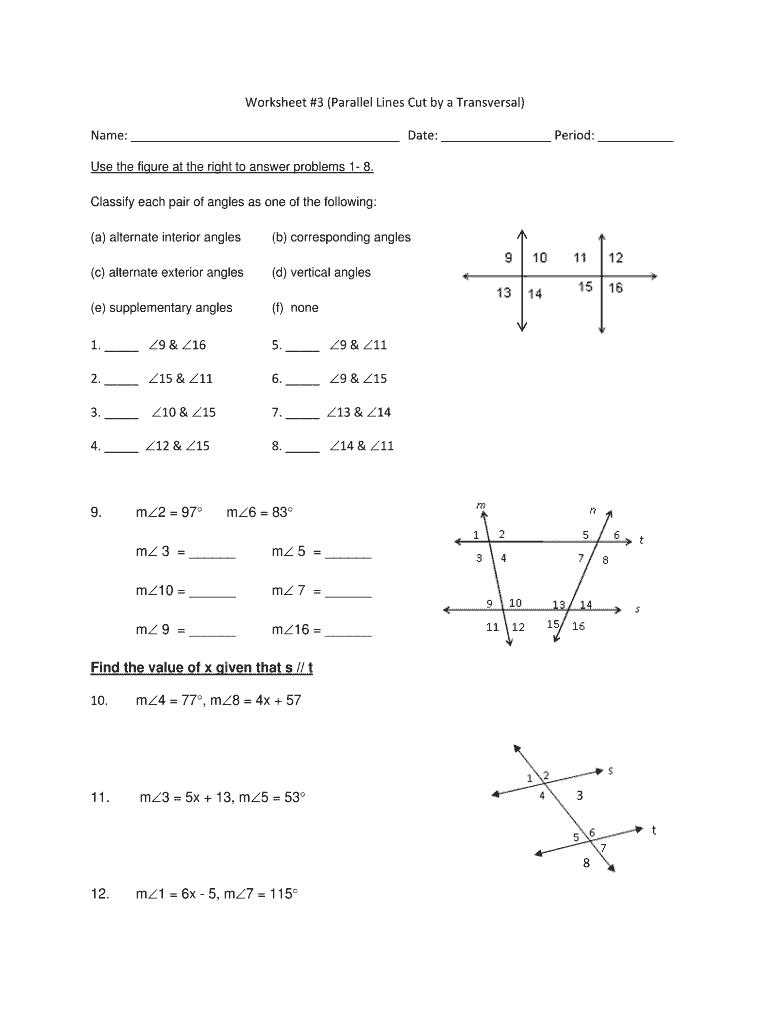
Parallel Lines and Transversals Algebra Worksheet Answers

Understanding the principles of parallel lines and transversals is fundamental in geometry, particularly when preparing for algebra and trigonometry. Students often encounter these concepts in various forms, whether through diagrams, problems, or worksheets, which require a strong grasp of how these lines interact. This post delves into how to approach problems on parallel lines and transversals using algebraic methods, offering detailed solutions and explanations to common problems found in algebra worksheets.
What are Parallel Lines and Transversals?

Parallel lines are two lines in the same plane that do not intersect or touch each other at any point. A transversal is a line that cuts across two or more (often parallel) lines. When a transversal intersects two parallel lines, several angle relationships are created, which are crucial for algebraic proofs and problem-solving:
- Corresponding Angles - Angles in the same relative position at each intersection.
- Alternate Interior Angles - Non-adjacent angles inside the parallel lines on opposite sides of the transversal.
- Alternate Exterior Angles - Angles outside the parallel lines, on opposite sides of the transversal.
- Consecutive Interior Angles (Co-interior Angles) - Pairs of interior angles on the same side of the transversal.
Solving Problems Using Algebra

Let's explore how algebra can be used to solve problems related to these angles:
Example Problem 1

If l1 and l2 are parallel lines and t is a transversal, and if the measure of ∠1 = 5x + 10 and ∠2 = 7x - 2, find the measures of these angles.
| Angles | Expression | Relation |
|---|---|---|
| ∠1 | 5x + 10 | Corresponding to ∠2 |
| ∠2 | 7x - 2 |

Since ∠1 and ∠2 are corresponding angles, they are equal:
5x + 10 = 7x - 2 10 + 2 = 7x - 5x 12 = 2x x = 6
Now substituting back to find the angles:
∠1 = 5(6) + 10 = 40° ∠2 = 7(6) - 2 = 40° ⚠️ Note: This calculation confirms that ∠1 and ∠2 are indeed equal, supporting the property of parallel lines and transversals.
Example Problem 2

Suppose you have angles ∠3 and ∠4, both on the same side of the transversal, and ∠3 = 3y + 15 and ∠4 = 2y + 45. Find the measures of these angles.
∠3 and ∠4 are consecutive interior angles which sum up to 180° when lines are parallel:
(3y + 15) + (2y + 45) = 180 5y + 60 = 180 5y = 120 y = 24
Plugging y back into the equations:
∠3 = 3(24) + 15 = 87° ∠4 = 2(24) + 45 = 93°
FAQ Section

What is the importance of angles formed by parallel lines and a transversal?
+
These angles are essential for understanding geometric properties, spatial relationships, and are crucial in fields like architecture, engineering, and design where precise measurements are needed.
How can algebra help solve geometric problems involving parallel lines?

+
Algebra provides a method to set up and solve equations based on the relationships between angles created by transversals, which is invaluable in proving geometric theorems and solving real-world problems.
Can you give an example of how to find an unknown angle using algebra?

+
Sure, if you have two angles such as ∠1 = 2x + 3 and ∠2 = x + 27, where ∠1 and ∠2 are vertical angles, you would set up the equation:
2x + 3 = x + 27Solve for x, and then find ∠1 and ∠2.
The exploration of parallel lines and transversals through algebraic methods provides a practical and systematic way to solve geometric problems. These skills are not only fundamental for succeeding in algebra but also apply in higher mathematics and real-world applications where precision is paramount. By understanding how to set up and solve these equations, students can confidently approach more complex problems, ensuring a solid foundation in both geometry and algebra.