Parallel and Perpendicular Lines Worksheet Solutions

What Are Parallel and Perpendicular Lines?
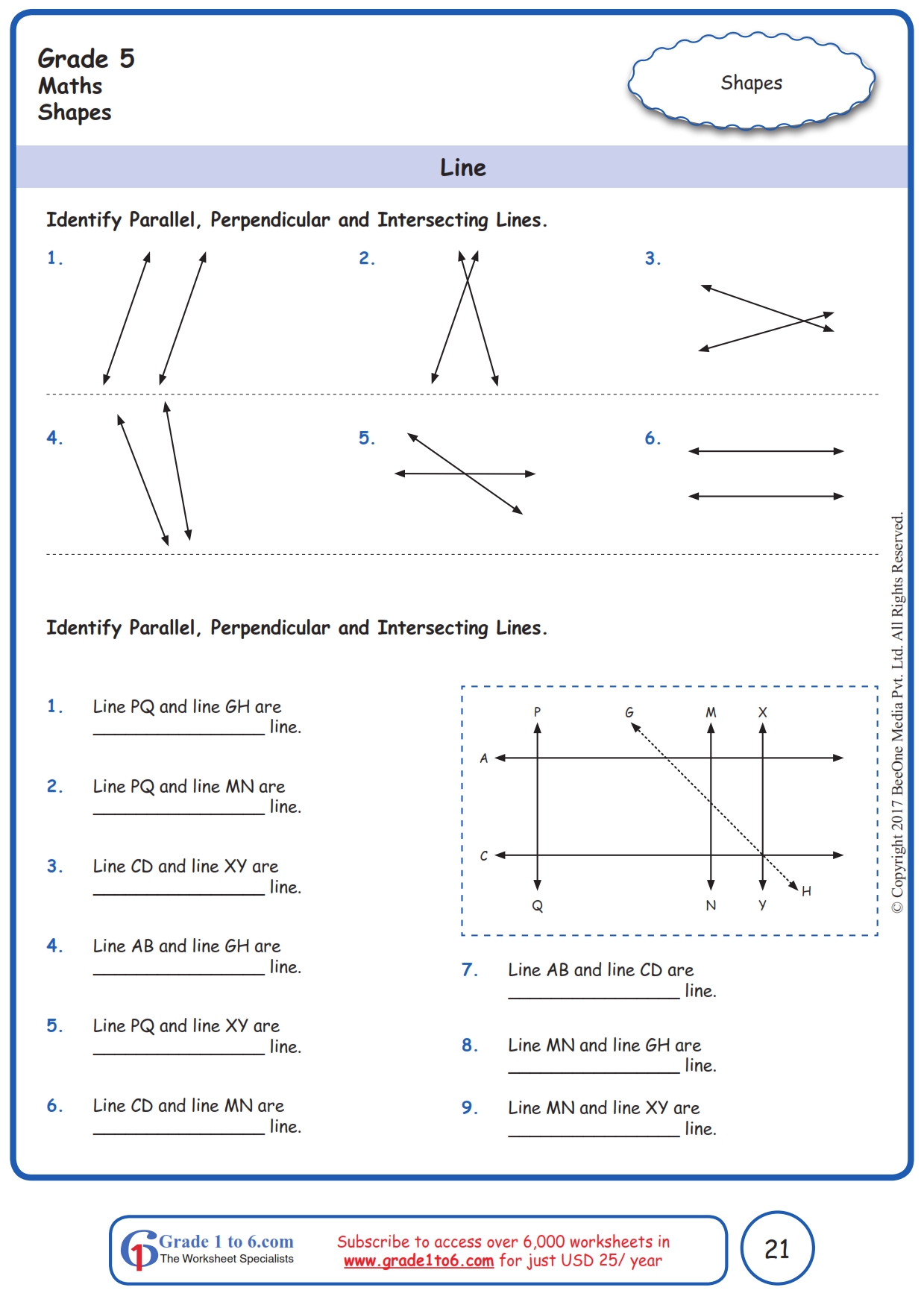
Parallel lines are those that run in the same direction and are equidistant from each other, never meeting at any point. They share the same slope in mathematical terms. Conversely, perpendicular lines intersect at a right angle, forming a 90-degree angle with each other. Their slopes are negative reciprocals of one another.

Understanding Slopes for Parallel and Perpendicular Lines

- Parallel Lines: They have the same slope. If line A has a slope of m, then line B must also have a slope of m to be parallel.
- Perpendicular Lines: The product of their slopes is -1. If line A has a slope of m, then line B, to be perpendicular, must have a slope of -1/m.

Examples of Parallel and Perpendicular Lines

Here are some practical examples to solidify your understanding:
- Parallel Example: Line A with the equation y = 2x + 1 and line B with the equation y = 2x - 3 are parallel because they both have a slope of 2.
- Perpendicular Example: If line A has the equation y = 3x + 2, then a perpendicular line would have a slope of -1⁄3. An equation could be y = -1/3x + 4.
🔍 Note: When graphing parallel or perpendicular lines, ensuring the slope calculations are correct is crucial for accuracy.

Step-by-Step Worksheet Solutions

Let’s delve into some common problems you might encounter on a worksheet:
Finding the Slope of Parallel Lines

Given an equation for line A, find the equation of line B that is parallel and passes through a specific point.
- Identify the slope of the given line.
- Use the slope-intercept form (y = mx + b) to write an equation for the new line, substituting the known point and the slope into the formula.
Example:
- Given Line A: y = 2x + 5
- Line B passes through (1,4)
- The slope of B is the same as A (m = 2)
y = 2x + b 4 = 2(1) + b b = 2 y = 2x + 2
🔍 Note: Always double-check your final equation against the given conditions.
Finding the Equation of Perpendicular Lines

If you have the equation of one line, find the equation of a perpendicular line that passes through a given point:
- Find the slope of the original line.
- Calculate the negative reciprocal for the slope of the new line.
- Substitute the new slope and the given point into the slope-intercept formula to find the y-intercept.
Example:
- Given Line A: y = 3x - 1
- Line B passes through (2, -3)
- The slope of B will be the negative reciprocal of 3, which is -1⁄3.
y = -1/3x + b -3 = -1⁄3(2) + b b = -3 + 2⁄3 = -2⁄3 y = -1/3x - 2⁄3

Determining If Lines Are Parallel or Perpendicular

To determine if given lines are parallel or perpendicular:
- If the slopes are the same, the lines are parallel.
- If the product of their slopes is -1, the lines are perpendicular.
Example:
- Line A: y = 4x - 6
- Line B: y = -4x + 2
- These lines are not parallel because their slopes are different, and not perpendicular because the product of their slopes isn’t -1.
In this final summary, we’ve covered how to identify and work with parallel and perpendicular lines. From understanding the properties of slopes, through finding equations for lines, to the determination of relationships between lines, each step is crucial for mastering this geometric concept.
What is the difference between parallel and perpendicular lines?

+
Parallel lines have the same slope, meaning they never meet. Perpendicular lines have slopes that are negative reciprocals of one another, intersecting at a right angle (90 degrees).
How do you find the slope of a line if it is given in equation form?

+
To find the slope of a line given its equation in slope-intercept form (y = mx + b), you simply look at the coefficient of x (the m value).
Can lines be both parallel and perpendicular at the same time?

+
No, lines cannot be both parallel and perpendicular simultaneously as these are mutually exclusive properties.