5 Ways to Order Real Numbers

Introduction to Real Numbers

Real numbers are a fundamental concept in mathematics, encompassing all rational and irrational numbers. They can be positive, negative, or zero, and are used to represent quantities that can be measured, such as length, weight, or time. Understanding how to order real numbers is crucial for various mathematical operations, including comparisons, calculations, and problem-solving. In this article, we will explore five ways to order real numbers, providing a comprehensive overview of this essential mathematical concept.

Understanding Real Numbers

Before diving into the methods for ordering real numbers, it’s essential to understand the different types of real numbers. Real numbers can be classified into two main categories: rational and irrational numbers. Rational numbers are those that can be expressed as the ratio of two integers, such as fractions or decimals that terminate or repeat. On the other hand, irrational numbers are those that cannot be expressed as a simple fraction, such as the square root of 2 or pi. Both rational and irrational numbers can be positive, negative, or zero, and can be used to represent a wide range of quantities.

Method 1: Using the Number Line

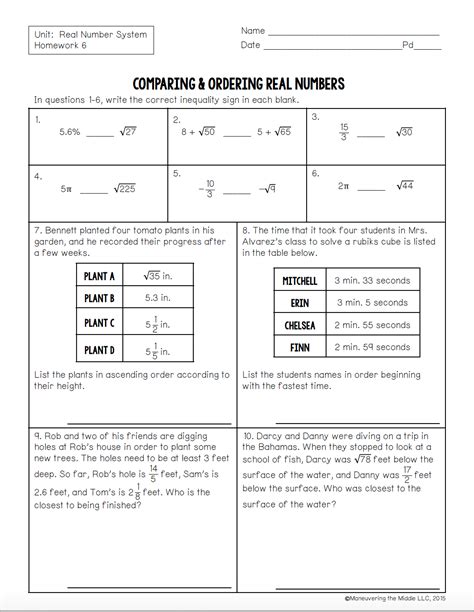
One of the most intuitive ways to order real numbers is by using a number line. A number line is a visual representation of real numbers, with negative numbers to the left and positive numbers to the right. Zero is the reference point, and all other numbers are positioned relative to it. To order real numbers using a number line, simply plot the numbers on the line and compare their positions. The number that is farther to the right is greater, while the number that is farther to the left is smaller. For example, -3 is less than 0, and 2 is greater than 0.

Method 2: Comparing Absolute Values

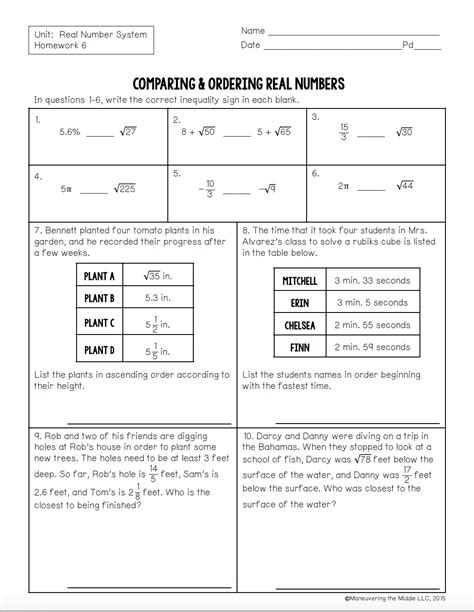
Another way to order real numbers is by comparing their absolute values. The absolute value of a number is its distance from zero, regardless of direction. To compare two real numbers using absolute values, calculate their absolute values and compare the results. The number with the larger absolute value is farther from zero, and is therefore greater. For instance, |5| = 5 and |-3| = 3, so 5 is greater than -3.

Method 3: Using Inequalities

Inequalities are a powerful tool for ordering real numbers. An inequality is a statement that compares two expressions using greater-than, less-than, greater-than-or-equal-to, or less-than-or-equal-to symbols. To order real numbers using inequalities, write the numbers as inequalities and compare the results. For example, 2 > 1 and -3 < 0, so 2 is greater than 1, and -3 is less than 0.

Method 4: Comparing Fractions and Decimals

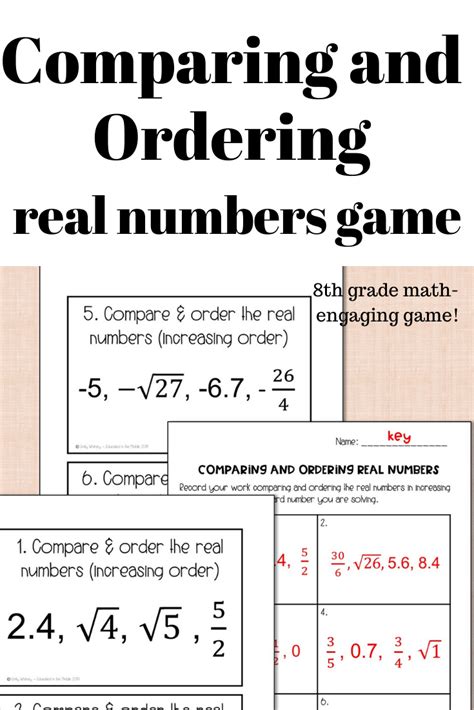
When working with rational numbers, such as fractions or decimals, it’s essential to compare them correctly. To order fractions, compare their numerators and denominators separately. If the numerators are equal, the fraction with the smaller denominator is greater. If the denominators are equal, the fraction with the larger numerator is greater. For decimals, compare the digits from left to right, starting with the most significant digit. The number with the larger digit in the most significant position is greater.

Method 5: Using Algorithms and Formulas

Finally, there are various algorithms and formulas that can be used to order real numbers. For example, the sign algorithm can be used to determine the sign of a product or quotient. If the signs of the factors are the same, the result is positive; otherwise, it’s negative. Another example is the distance formula, which can be used to calculate the distance between two points on a coordinate plane. By using these algorithms and formulas, you can order real numbers in a systematic and efficient way.

📝 Note: When working with real numbers, it's essential to consider the context and the specific problem you're trying to solve. Different methods may be more suitable for different situations, so it's crucial to understand the strengths and limitations of each approach.

In summary, ordering real numbers is a fundamental concept in mathematics, and there are several methods to achieve this. By understanding the different types of real numbers, using number lines, comparing absolute values, using inequalities, comparing fractions and decimals, and applying algorithms and formulas, you can develop a comprehensive understanding of real numbers and their ordering. This knowledge is essential for various mathematical operations and problem-solving, and will serve as a foundation for more advanced mathematical concepts.