Mastering Opposites and Absolute Value: Fun Worksheet

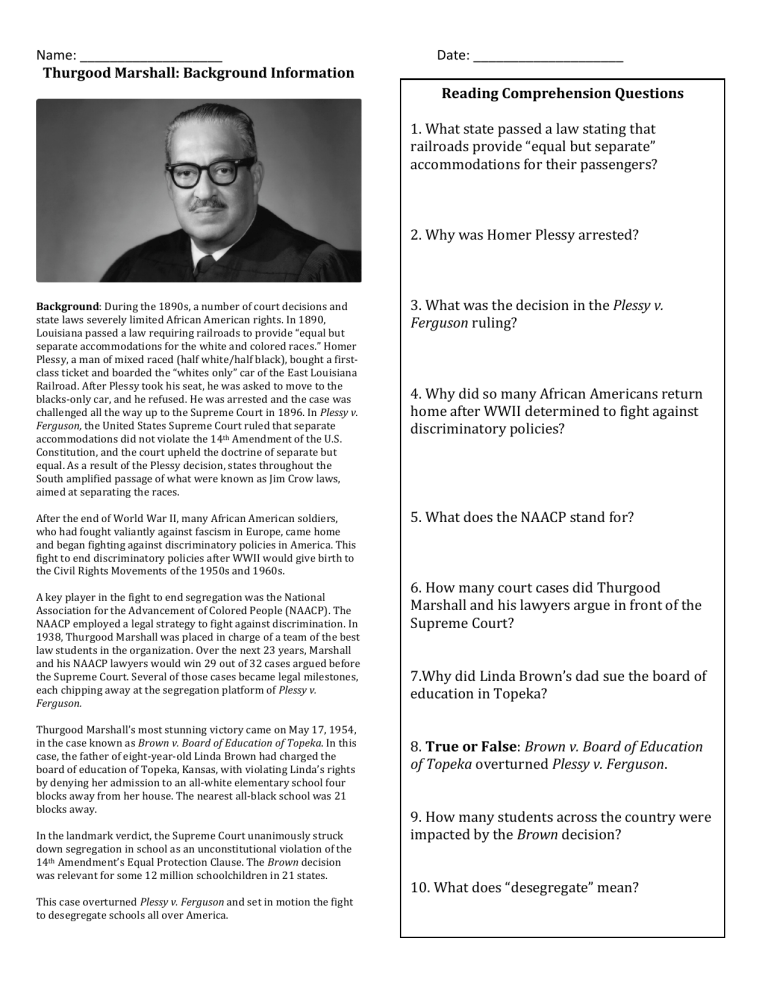
Let's delve into the fascinating world of opposites and absolute value, two integral concepts in mathematics that are not only fun to explore but also crucial for building a strong foundation in algebra and beyond. Whether you're a student encountering these for the first time or a math enthusiast seeking a deeper understanding, this journey through worksheets and real-life applications will enhance your grasp on these mathematical notions.
Understanding Opposites

Opposites, often referred to as additive inverses, are numbers that when added together result in zero. Here’s how they work:
- Every positive number has a negative counterpart, and vice versa. For example, 5 and -5 are opposites because 5 + (-5) = 0.
- Zero is its own opposite, as 0 + 0 = 0.
The Concept of Absolute Value

Absolute value represents the distance of a number from zero on the number line, always expressed as a non-negative number. Here are key points:
- The absolute value of a positive number or zero is itself. E.g., |5| = 5.
- The absolute value of a negative number is its positive counterpart. For instance, |-5| = 5.
How Opposites and Absolute Value Interact

The interplay between opposites and absolute values is where the magic happens:
- The sum of a number and its opposite is zero, aligning with the concept of opposites.
- The distance between a number and its opposite on a number line is twice the absolute value of the number, which introduces the idea of the additive inverse in spatial terms.
Worksheet Ideas

To help students practice, here are some worksheet ideas:
Worksheet for Understanding Opposites

| Number | Opposite | Sum of Opposites |
|---|---|---|
| 3 | -3 | 0 |
| -6 | 6 | 0 |

✨ Note: Use number lines to visually represent the concept of opposites and help students understand the spatial relationship between numbers and their opposites.
Worksheet for Absolute Value

Construct exercises where students find the absolute value of numbers, calculate differences between numbers and zero on a number line, and relate these differences to the absolute value.
Integrating Opposites and Absolute Value in Real Life

Understanding these concepts is not just an academic exercise; they have practical applications:
- Financial Management: Learning to handle debits and credits, where credits are the “opposites” of debits.
- Navigation: Absolute value can help in understanding the shortest distance between two points, irrespective of direction.
Deepening the Concept

For those looking to expand their understanding:
- Group Theory: Opposites align with the concept of inverse elements in group theory, where opposites are elements whose product is the identity element.
- Applications in Physics: Absolute value plays a role in measuring forces, where the magnitude of the force is its absolute value.
In summary, opposites and absolute values are foundational concepts in mathematics that not only help in understanding numbers better but also have significant implications in various fields. From financial planning to advanced physics, the principles of opposites and absolute values offer insights into the structure of numbers and their applications in our everyday lives. By working through worksheets and real-life scenarios, learners can build a robust understanding of these concepts, enhancing their problem-solving skills and deepening their appreciation for mathematics.
Why are opposites important in mathematics?

+
Opposites allow for the concept of zero in number systems, essential for addition and subtraction. They also help in understanding symmetry and balance in algebra and beyond.
How does absolute value relate to distance?

+
The absolute value of a number represents its distance from zero on the number line, providing a measure of magnitude without considering direction.
Can I use opposites and absolute value in problem-solving?

+
Absolutely! These concepts are key in solving equations, understanding vectors, and tackling mathematical word problems involving distances, speed, or change in quantity.