5 Ways to Master Scientific Notation Operations

Scientific notation is a crucial tool in the world of mathematics and science, enabling us to deal with very large or very small numbers efficiently. Whether you're calculating the distance to distant galaxies or the size of subatomic particles, understanding scientific notation not only simplifies these calculations but also makes them more manageable. Here are five strategies to help you master operations involving scientific notation:
1. Understanding the Basics

Before diving into operations, ensure you understand what scientific notation is. At its core:
- A number is expressed as a product of two parts: a decimal number between 1 and 10 (called the coefficient), and a power of ten (the exponent).
- Example: 3.14159 × 10² (representing 314.159).

2. Addition and Subtraction
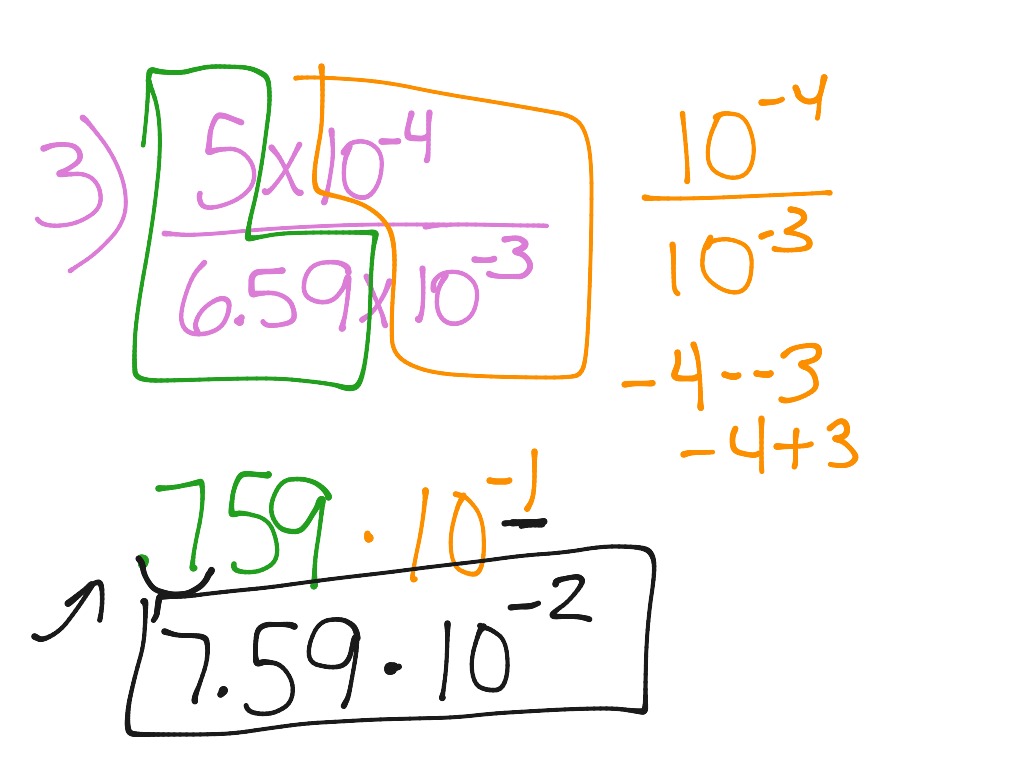
Adding or subtracting numbers in scientific notation involves aligning the exponents. Here’s how:
- Ensure that both numbers have the same exponent. If not, adjust one by multiplying or dividing the coefficient by an appropriate power of ten to match the exponents.
- Add or subtract the coefficients.
- Express the result in scientific notation if necessary.
| Example: | (3 × 10^3) + (4.5 × 10^4) |
| Adjust: | (3 × 10^3) → (0.3 × 10^4) |
| Operation: | (0.3 + 4.5) × 10^4 = 4.8 × 10^4 |

3. Multiplication and Division

Multiplying or dividing scientific notation:
- Multiply (or divide) the coefficients.
- Add (or subtract) the exponents.
⚠️ Note: The sign of the exponent determines the direction of the decimal point movement.
Example:
| Multiplication: | (5 × 10^6) × (3 × 10^-3) |
| Result: | (5 × 3) × 10^(6+(-3)) = 15 × 10^3 or 1.5 × 10^4 |
4. Handling Exponents

When working with exponents in scientific notation:
- Adding exponents when multiplying, subtracting when dividing.
- Know how to handle negative exponents (denotes division).
- Be aware of the “Product of Powers” rule.
Example:
| (5 × 10^6)^3 | |
| Result: | 5^3 × 10^(6×3) = 125 × 10^18 |
5. Converting Between Decimal and Scientific Notation
Conversions are often needed when dealing with real-world applications:
- To convert to scientific notation: Move the decimal until you get a number between 1 and 10, then adjust the exponent accordingly.
- To convert back: Reverse the process, moving the decimal left or right based on the exponent.
📝 Note: Converting should not alter the value of the number, just its representation.
Mastering scientific notation isn't just about performing calculations; it's about understanding the underlying principles of how numbers work in the vast expanse of measurements and mathematics. As you practice these strategies, you'll find that operations in scientific notation become second nature, enabling you to tackle scientific and mathematical problems with precision and ease.
How do I know when to use scientific notation?

+
Scientific notation is particularly useful when dealing with very large or very small numbers, which are common in scientific and engineering contexts. Use it when standard decimal notation becomes cumbersome or when significant figures need to be maintained accurately.
Can I use a calculator for these operations?

+
Yes, many calculators can perform operations with numbers in scientific notation. This can save time and reduce the chance of error in manual calculations, especially for complex operations.
What if I get different powers of 10 when adding or subtracting?

+
You must make the exponents the same by adjusting one number’s coefficient through multiplication or division by a power of ten to match the other number’s exponent before you perform the operation.